Sums of Powers of Positive Integers - Solutions to Exercises 7-8

Exercise 7.

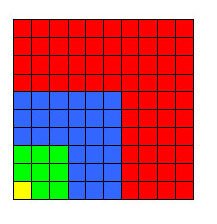
Figure 18. (1 + 2 + 3 + 4)² = 1³ + 2³ + 3³ + 4³

$$(1 + 2 + 3 + 4)^2 = (1 + 2 + 3)^2 + \left( {2 \cdot 4(1 + 2 + 3) + 4^2 } \right) \quad\quad {\rm (Step\ 1)}$$

$$= (1 + 2 + 3)^2 + \left( {2 \cdot 4{{3 \cdot 4} \over 2} + 4^2 } \right)$$

$$= (1 + 2 + 3)^2 + \left( {4^2 (3 + 1)} \right)$$

$$= (1 + 2 + 3)^2 + 4^3$$

$$= (1 + 2)^2 + \left( {2 \cdot 3(1 + 2) + 3^2 } \right) + 4^3 \quad\quad {\rm (Step\ 2)}$$

$$= (1 + 2)^2 + \left( {2 \cdot 3{{2 \cdot 3} \over 2} + 3^2 } \right) + 4^3$$

$$= (1 + 2)^2 + \left( {3^2 (2 + 1)} \right) + 4^3$$

$$= (1 + 2)^2 + 3^3 + 4^3$$

$$= 1 + (2 \cdot 2 \cdot 1 + 2^2 ) + 3^3 + 4^3 \quad\quad\quad {\rm (Step\ 3)}$$

$$= 1 + (2 \cdot 2^2 ) + 3^3 + 4^3$$

$$(1 + 2 + 3 + 4)^2= 1^3 + 2^3 + 3^3 + 4^3$$

Each cubic term represents a gnomon. In particular, 1³, 2³, 3³, and 4³ are, respectively, the areas of the yellow, green, blue, and red gnomons in Figure 18.

Exercise 8. $$1^4 + 2^4 + 3^4 + \cdots + n^4 = {1 \over 5}n^5 + {1 \over 2}n^4 + c_3 n^3 + c_2 n^2 + c_1 n + c_0,$$

where $$c_3 + c_2 + c_1 + c_0 = 1 - \left( {{1 \over 5} + {1 \over 2}} \right) = {3 \over {10}}.$$

Three possible formulas are

$${1 \over 5}n^5 + {1 \over 2}n^4 + {1 \over {10}}n^3 + {1 \over {10}}n^2 + {1 \over {10}}n,$$

$${1 \over 5}n^5 + {1 \over 2}n^4 + {1 \over 5}n^3 + {1 \over {10}}n, \quad {\rm and}$$

$${1 \over 5}n^5 + {1 \over 2}n^4 + {1 \over 5}n^3 + {1 \over {15}}n^2 + {1 \over {30}}n.$$

The correct formula is $$1^4 + 2^4 + 3^4 + \cdots + n^4 = {1 \over 5}n^5 + {1 \over 2}n^4 + {1 \over 3}n^3 - {1 \over {30}}n.$$

Surprised?