Sums of Powers of Positive Integers - Archimedes (287-212 BCE), Greece-Italy

The Greek mathematician, scientist, and inventor Archimedes spent most of his life in Syracuse in southern Italy. Considered the greatest mathematician of antiquity, he is famous for a number of mathematical and scientific discoveries and also for a number of inventions, many of them machines of war used in his city’s defense against Roman armies. Archimedes knew the Pythagorean “formula” for the sum of the first n positive integers. He almost certainly knew also a “formula” for the sum of the squares of the first n positive integers. His Lemma to Proposition 2 in On Conoids and Spheroids (Heath, p. 57) and his Proposition 10 in On Spirals (Heath, pp. 68-69), translated to our symbols, say that

$$(n + 1)n^2 + (1 + 2 + 3 + \cdots + n) = 3(1^2 + 2^2 + 3^2 + \cdots + n^2 )\quad (*),$$

as illustrated in Figure 3 (see also Nelsen, p. 77, and Stein, pp. 46-49).

Figure 3 shows literally that

$$3(1^2 + 2^2 + 3^2 + 4^2 ) = 5 \cdot 4^2 + (1 + 2 + 3 + 4).$$

Using the Pythagorean formula to replace \(1 + 2 + 3 + · · · ­+ n\) by \({{n(n + 1)} \over 2}\) in Archimedes' equation

$$(n + 1)n^2 + (1 + 2 + 3 + \cdots + n) = 3(1^2 + 2^2 + 3^2 + \cdots + n^2 )\quad (*),$$

we have

$$(n + 1)n^2 + {\frac{n(n + 1)}{2}} = 3(1^2 + 2^2 + 3^2 + \cdots + n^2 ).$$

Solving for the sum of squares, we get

$$1^2 + 2^2 + 3^2 + \cdots + n^2 = {\frac{(n + 1)n^2}{3}} + {\frac{n(n + 1)}{6}},$$

or $$1^2 + 2^2 + 3^2 + \cdots + n^2 = {{n(n + 1)(2n + 1)} \over 6}$$

for all positive integers n.

Since Archimedes concluded in a Corollary to his Lemma to Proposition 2 (Heath, p. 57) that

$$3(1^2 + 2^2 + 3^2 + \cdots + n^2 ) > n^3 > 3(1^2 + 2^2 + 3^2 + \cdots + (n - 1)^2 ),$$

presumably, he, too, replaced \(1 + 2 + 3 + · · · ­+ n\) by \({{n(n + 1)} \over 2}\) in his equation (*), and deduced that

$$1^2 + 2^2 + 3^2 + \cdots + n^2 = {{n(n + 1)(2n + 1)} \over 6}.$$

Again, Archimedes would have described these equations and inequalities in words rather than symbols.

Exercise 2: Use cubes (or squares) to represent the triangular numbers and to illustrate the identity

$$1 + 2 + 3 + \cdots + n = {{n(n + 1)} \over 2}$$

and the identity from Exercise 1.

Exercise 3: Use 60 or 120 cubes to illustrate the identity

$$1 + 3 + 6 + \cdots + {{n(n + 1)} \over 2} = {{n(n + 1)(n + 2)} \over 6}$$

for n = 3 or n = 4. Hint: One way to construct a pyramid representing the sum of the first four triangular numbers, 1, 3, 6, and 10, is to place a single cube representing 1 atop a configuration of three cubes, which, in turn, is placed atop a configuration of 6 cubes, as shown in Figure 4. Just one more layer of 10 cubes will result in a pyramid containing 1 + 3 + 6 + 10 cubes. How many such pyramids should you construct in order to form a 4-cube by 5-cube by 6-cube rectangular solid? (Nelsen, p. 95)

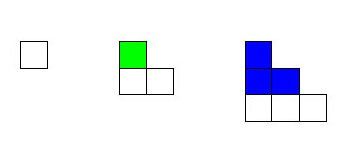
Figure 4. Place the single cube atop the shaded portion of the layer of three cubes. Place the resulting pyramid of 1 + 3 cubes atop the shaded portion of the layer of six cubes. Only tops of cubes are shown.

Solutions to these exercises can be found here.