Kepler and the Rhombic Dodecahedron: Building a Cube and an Octahedron Inside a Rhombic Dodecahedron

As previously suggested, the best way to understand three-dimensional bodies is to make physical models. As a complement to our construction of a rhombic dodecahedron, we close by building a few other related polyhedra.

We already know that we can put a cube inside a rhombic dodecahedron. The vertices of this cube are the vertices of the rhombic dodecahedron that join three rhombi.

 
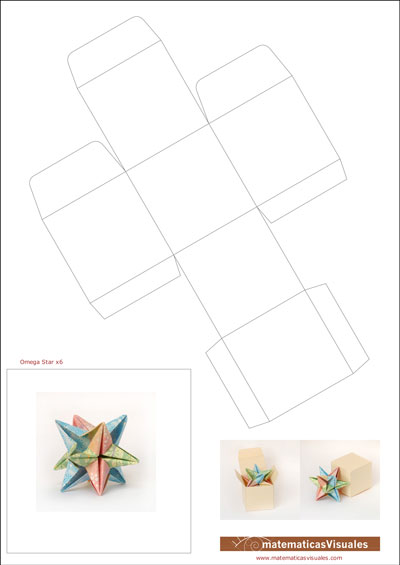
Figure 29. A cube inside a rhombic dodecahedron and its corresponding net.
(Note that the net is scaled to produce a model that fits inside the rhombic dodecahedron constructed earlier.)

As shown in the template given in Figure 29, we can also put a beautiful piece of origami, an omega star, inside our cube. The omega star has the structure of a cuboctahedron. It is both easy and a real pleasure to fold this example of “modular origami” using six pieces of paper. Highly recommended! The square in the lower left-hand corner of the template is correctly sized for building an origami star that fits inside the cube. (For instructions on how to build the star itself, see, for example, this YouTube video by Philip Shen.) With a bit of imagination, we can see from Figure 30 that the twelve vertices of the omega star (cuboctahedron) are located at the centers of the rhombic faces of the rhombic dodecahedron.

Figure 30. A rhombic dodecahedron, a cube, and an omega star (cuboctahedron).

Finally, as suggested in Figure 27, the six vertices of the rhombic dodecahedron at which four rhombi meet are also the vertices of an inscribed octahedron. The net given in Figure 31 below is again scaled perfectly to construct an octahedron that fits inside the rhombic dodecahedron that you constructed earlier.

 
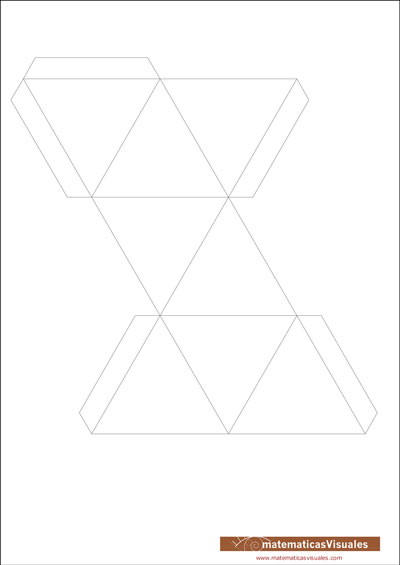
Figure 31. An octahedron inside a rhombic dodecahedron and its corresponding net. 
(Again, the net is scaled to to produce a model that fits inside the rhombic dodecahedron constructed earlier.)