A GeoGebra Rendition of One of Omar Khayyam's Solutions for a Cubic Equation - Shouldn't a Cubic Have Three Roots?

The Medieval Islamic geometrical perspective ruled out negative coefficients and solutions, but this constructive technique from Khayyam is nonetheless capable of finding all real solutions to a cubic of the form \(x^3 + C_1x^2 + C_2x = C_3.\) A few additions to the construction generate the other two real roots (if they exist). First, we need to construct the complete circle on diameter \(\overline{GD}\) and not simply the top semi-circle. Second, we need the other half of the hyperbola through point \(G\) with asymptotes \(\overline{HK}\) and \(\overline{HB}\). The lower half of the hyperbola intersects the circle at two points, or at one point of tangency, or not at all. If the lower half of the hyperbola intersects the circle at two distinct points, the \(x\)-values corresponding to those intersection points are two negative roots to the cubic. If the lower half of the hyperbola intersects the circle at a point of tangency, the \(x\)-value of that point corresponds to a repeated negative root. If the lower half of the hyperbola does not intersect the circle, then there is a pair of complex roots. We know, by Descartes' Rule of Signs, that polynomials of the form \(x^3 + ax^2 + b^2 x = c^3,\) with \(a, b, c>0,\) cannot have more than one positive root. Khayyam's construction as presented finds a single, positive root and, if enhanced by completing the circle and hyperbola, gives additional information about all roots to a cubic of the specified form.

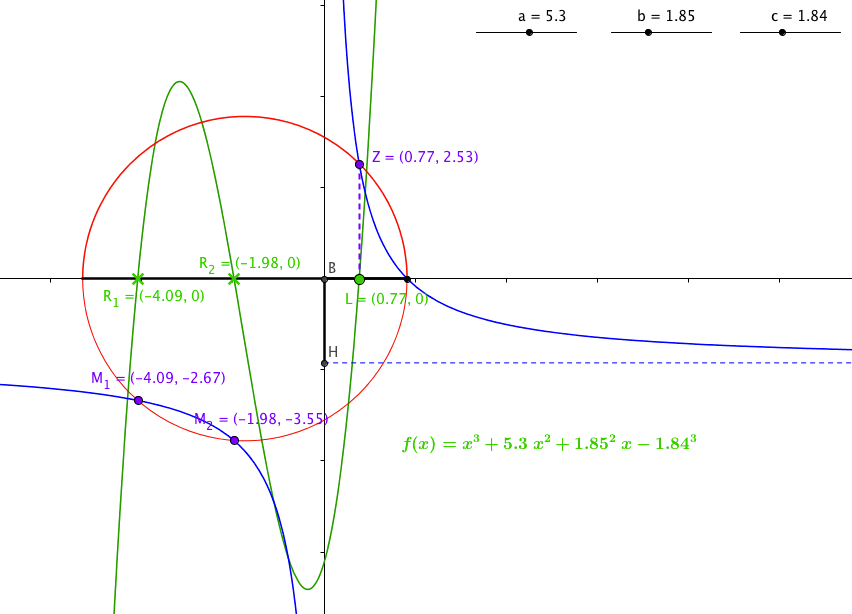
Figure 12. A reflection of Khayyam's construction for the cubic \(x^3 + 5.3x^2 + 1.85^2x = 1.84^3,\) enhanced with the complete hyperbola and both halves of the circle. The Cartesian graph of the cubic function \(f(x) = x^3 + 5.3x^2 + 1.85^2x - 1.84^3\) is superimposed on the construction. This illustrates that the \(x\)-coordinates of the points of intersection (not along the \(x\)-axis) between the circle and the hyperbola are, in fact, roots to the cubic.

Figure 13. A reflection of Khayyam's construction for the cubic \(x^3 + ax^2 + b^2x = c^3,\) enhanced with the complete hyperbola and both halves of the circle. The Cartesian graph of the cubic function \(f(x) = x^3 + ax^2 + b^2x - c^3\) is superimposed on the construction. This illustrates that the \(x\)-coordinates of the points of intersection (not along the \(x\)-axis) between the circle and the hyperbola are, in fact, roots to the cubic.