A GeoGebra Rendition of One of Omar Khayyam's Solutions for a Cubic Equation - Khayyam's Construction

Khayyam began by providing directions from which the reader can construct a line segment that is the solution to a polynomial of the form “cubes and squares and roots equal a number” (Fauvel and Gray, p. 233). Note that “cubes” corresponds to a modern \(x^3\) term. Likewise, Khayyam's “squares” would be \(x^2\) and his “roots” \(x.\) Modern notation expresses this sum of cubes, squares, and roots equal to a number in the form \(x^3 + C_1x^2 + C_2x = C_3,\) with \(C_1, C_2, C_3>0.\) In Khayyam's proof (Fauvel and Gray, pp. 233-234), he treated each of these terms as a parallelepiped volume, suggesting a contemporary articulation of the form \(x^3 + ax^2 + b^2x = c^3.\) (For example, in the cubic \(x^3 + 4x^2 + 6x = 8,\) values for the construction would be \(a = 4,\) \(b = {\sqrt{6}},\) and \(c = 2.\)) The following set of instructions constructs a line segment \({\overline{x}},\) whose length \(x\) is a solution to a cubic equation of the form \(x^3 + ax^2 + b^2x = c^3,\) \(a, b, c >0.\) The result of this construction can be seen in Figure 5, and Figure 6 is a movie showing all steps of this construction.

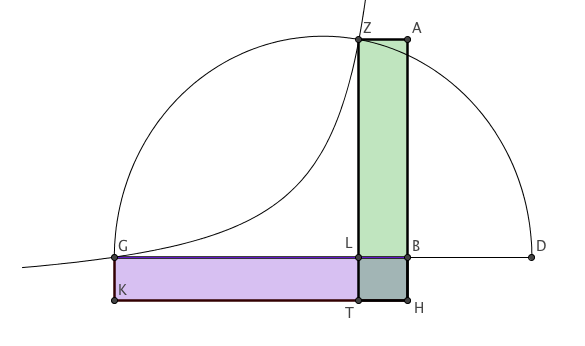
Figure 5. Khayyam's construction, in which line segment \(\overline{LB}\) (for Khayyam) or its length \(LB\) (for us) is a solution to the cubic equation \(x^3 + ax^2 + b^2x = c^3,\) where \(a, b, c >0.\)

First, Khayyam said to construct a line segment \(\overline{HB}\) of length \(b\), the square root of the given number of edges. Then build a rectangular solid of volume \(c^3\) whose base is a square with sides of length \(b\). Construct a line segment \(\overline{BG}\) perpendicular to \(\overline{HB}\) so that the length of \(\overline{BG}\) is the height of this rectangular solid. Since the area of the base is \(b^2\) and the volume \(c^3\), then in terms of coefficients of the modern polynomial, \(\overline{BG}\) is of length \(\frac{c^3}{b^2}.\) The final piece of the construction utilizing given information is to put point \(D\) in line with line segment \(\overline{GB}\) such that \(\overline{DB}\) is a line segment of length \(a.\)

Next, construct a semi-circle with diameter \(\overline{GD}\). In Khayyam's diagram, this is the semi-circle in the upper half plane. The line segments \(\overline{GB}\) and \(\overline{HB}\) then determine a rectangle that is completed by adding point \(K\) and line segments \(\overline{GK}\) and \(\overline{HK}\). Draw then a hyperbola passing through point \(G\) with asymptotes \(\overline{HK}\) and \(\overline{HB}\). This hyperbola will intersect the semicircle again at a known point \(Z\). Drop a line from \(Z\) perpendicular to diameter \(\overline{GD}\) that intersects \(\overline{GD}\) at point \(L\) and intersects line segment \(\overline{KH}\) at point \(T\). Khayyam asserted that the line segment \(\overline{LB}\) is the solution to the cubic, whereas we would say that its length \(LB\) is a solution. The conflation of a line segment and its length may appear sloppy to modern readers, but this is faithful to Khayyam's presentation, in which the line segment is viewed as the solution.

At this point, although Khayyam had constructed the segment which satisfies the given cubic, his instructions continued in order to complete the geometrical constructions necessary to justify \(\overline{LB}\) as a solution. So, he said, draw a line through point \(Z\) perpendicular to the line segment \(\overline{ZLT}\) just constructed. Extend line segment \(\overline{HB}\) to intersect that line at point \(A\). These rectangles concluded Khayyam's construction.

Khayyam's construction is illustrated dynamically in the two-minute movie and the interactive applet in Figures 6a and 6b below.

Figure 6a. Khayyam's construction, in which the length \(LB\) of the line segment \(\overline{LB}\) is the positive solution to the cubic equation \(x^3+7.9x^2 +{{1.3}^2}x={2.1}^3.\) This solution is shown as a zero on the graph of the cubic function \(f(x) = x^3+7.9x^2 +{{1.3}^2}x-{2.1}^3\) at right. After starting the two-minute movie, to make the viewing window larger, click the "Full screen" icon in the lower right corner. To return to this article, press the Escape key.

Figure 6b. Khayyam's construction, in which the length \(LB\) of the line segment \(\overline{LB}\) is the positive solution to the cubic equation \(x^3+7.9x^2 +{{1.3}^2}x={2.1}^3.\) This solution is shown as a zero on the graph of the cubic function \(f(x) = x^3+7.9x^2 +{{1.3}^2}x-{2.1}^3\) at right. Use the arrows to click through each step of the construction, or click the circular "Play" (or "Pause") icon to see the slideshow. The box labeled "s" may be used to change the speed of the slideshow. Finally, to change the cubic equation, adjust the sliders for coefficients \(a,\) \(b,\) and \(c.\)

Intermingled with the above instructions is a justification that the constructed line segment \(\overline{LB}\) indeed satisfies the cubic. A presentation of Khayyam's argument follows on the next page. Key steps are highlighted and explanations of geometric facts perhaps less familiar to modern readers are included. The main tools in Khayyam's argument are ratios and geometric reasoning, along with the properties of the hyperbola and circle.