A GeoGebra Rendition of One of Omar Khayyam's Solutions for a Cubic Equation - An Algebraic Rendering of the Demonstration

A geometric solution to a cubic equation may seem peculiar to modern eyes, but the study of cubic equations (and indeed much of medieval algebra) was motivated by geometric problems. Khayyam stated in his text that the arithmetic problem of the cubic remained to be solved. In particular, he sought to articulate an algorithm for finding root(s) of a cubic equation by operating on its coefficients. He never produced such a solution; nor did anyone else until Girolamo Cardano in the mid-16th century. Readers of this article may be particularly interested to see the relationships between a modern polynomial and Khayyam's geometric treatment of a cubic.

Consider the polynomial \(x^3 + ax^2 + b^2x = c^3.\) To follow Khayyam's demonstration, take \(HB = b,\) \(DB = a,\) and \(BG = \frac{c^3}{b^2} = p.\) Recall the hyperbolic property from Apollonius that states the product of the distances from any point on the curve to each of the asymptotes is a constant. We repeat Figure 5, which illustrates Khayyam's construction, for convenience:

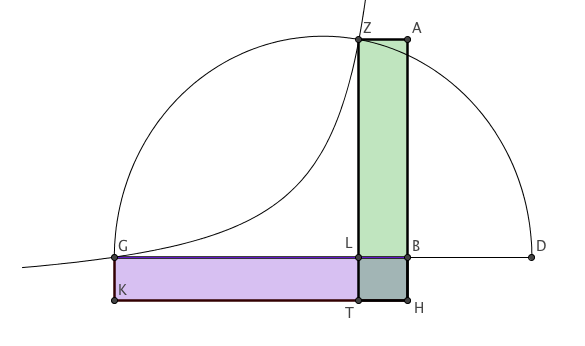
Figure 5. Khayyam's construction, in which line segment \(\overline{LB}\) (for Khayyam) or its length \(LB\) (for us) is the solution to the cubic.

If we take G = \((p,b)\) to be a fixed point on the hyperbola and Z = \((x,y)\) to be any point on the hyperbola, then this property for any point Z = \((x,y)\) could be written as \(pb = x(y + b)\).  This would be in relation to an origin \((0,0)\) at the intersection of segments \(\overline{BH}\) and \(\overline{TH}\). Readers may wish to work through the remainder of Khayyam's justification from this modern perspective.