Mathematical Treasures – Wallis's 1655–56 Works on Quadrature

Arithmetica Infinitorum (1656) is considered to be the most important work of John Wallis (1616–1703). On the cover the author promised “New methods of inquiry into the quadrature of curves.” In his book Wallis combined and extended the analytic methods of Rene Descartes and Bonaventura Cavalieri to provide a foundation for the new calculus.

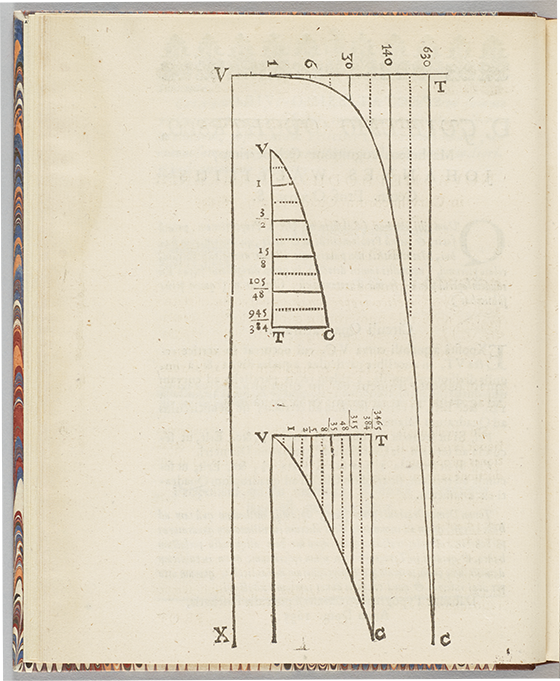
In a foreword note addressed to William Oughtred, John Wallis discussed his method of quadrature. The accompanying diagram illustrates his concept of partitioning the area under a curve into narrow strips and finding their sum. Wallis also dedicated the Arithmetica to Oughtred.

This is the first page of the text.

Here on pages 24 and 25, Wallis discussed the properties of the spiral.

In 1655, John Wallis published De Sectionibus Conicis, in which he also demonstrated his “new method” of employing indivisibles in the determination of quadrature.

Here on pages 4 and 5, the author demonstrated the use of indivisibles on conics.

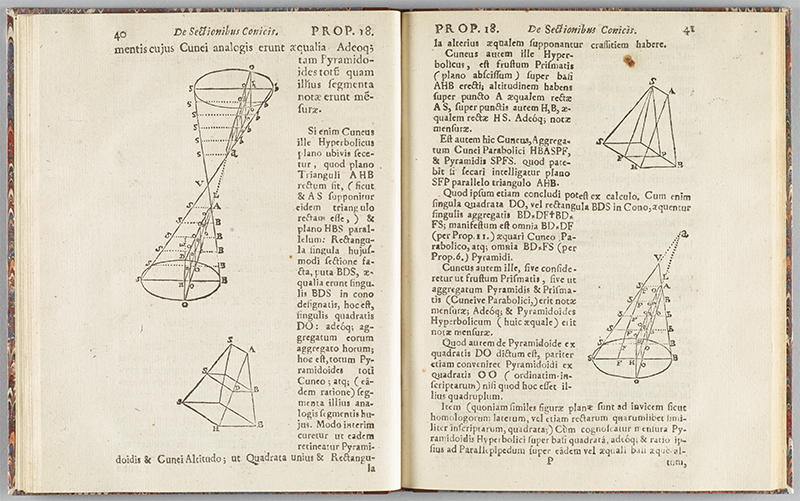
On pages 40 and 41 of the Sectionibus, Wallis generalized his method of indivisibles from conics to prisms.

These images from its George Arthur Plimpton Collection are presented through the courtesy of the Columbia University Libraries.

Index to Mathematical Treasures