Mathematical Treasures - Van Heuraet's Rectification of Curves

This is the title page of the brief work On the Transformation of Curves into Straight Lines, by Hendrick van Heuraet (1634–1660), published in the 1659 Latin edition of Descartes's Geometry, edited by van Schooten.  Although van Heuraet was not the first to accomplish a rectification, a task that Descartes had said could not be done, this is the first publication of a general procedure, a procedure very close to our standard calculus procedure for finding the length of a curve.

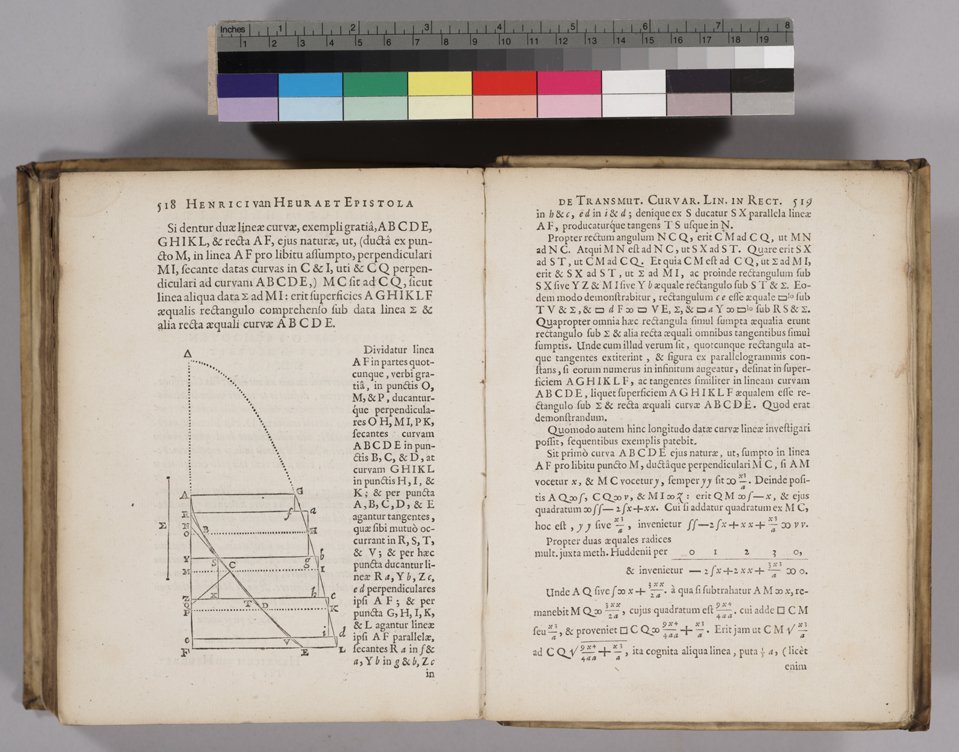
On these two pages, van Heuraet describes his general procedure for rectification, one which tranforms the length into an integral, that is, the area under a curve.  He then illustrates the procedure by calculating the length of the semi-cubical parabola, y² = x³/a.  (We can take a = 1 for simplicity.)  Note that since the procedure for finding arc length involved first finding dy/dx (or the tangent to the curve), van Heuraet accomplishes this by using Descartes's normal method and Hudde's rule for finding a double root.  Note also that van Heuratet uses Descartes's symbol for "equal" rather than our modern equal sign.

On this page, van Heuraet completes his calculation, noting that the answer is found by determining the area under the parabola.  He further notes that he could also determine the lengths of the curves y⁴ = x⁵, y⁶ = x⁷, and so on.  Finally, he shows that to find the length of a parabola he needs to be able to find the area under a hyperbola.

Index to Mathematical Treasures