Topology from Analysis: A Mini-Primary Source Project for Topology Students

During my second year at Ursinus, I taught an undergraduate course in point-set topology. My doctoral work was in topology, so I was quite excited to teach the course. We were almost halfway though the semester, and I thought the class was going great. “These kids are really getting it," I thought to myself. Yet one day in class while discussing a proof involving the product topology, a student remarked that “I can follow the proof and I understand it, but I don’t know what we are doing." What the student later told me this meant was that he could justify every line of the proof, but didn’t understand its significance. Great, now we know that the collection of all preimages of open sets under the projection forms a subbasis . . . so? This student’s experience was not unlike my own as an undergraduate. When I took point-set topology, I knew that what we were doing was math, but I could not tell you how concepts in topology would naturally arise from the other math that I had studied. The mini-Primary Source Project Topology from Analysis is an attempt to bridge this conceptual gap between topology and more familiar concepts in mathematics.

The student project Topology from Analysis begins by investigating what the question that interested Cantor means. By closely examining an example of an infinite set on which a function could be undefined but still have a unique Fourier series representation, the student is able to see why someone would write down the definition of a limit point. That same example also lays out the basic idea behind Cantor’s main result. Cantor’s definition of a derived set and its subsequent iterations are then introduced, to set up the notion that some infinite sets (e.g., point sets of finite type) are “more well-behaved" than others. Following some practice in computing derived sets, the project ends by guiding the student through Cantor’s proof of the main result of his 1872 paper. By tracing Cantor’s proof in this way, students are more naturally led to see the motivation behind the technique of proof by induction, as well as sophisticated topological concepts such as limit point and derived set.

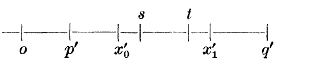
Diagram from Cantor's 1872 proof for uniqueness of Fourier series representation,
illustrating the relationship of points on an interval in key step of his proof.
See also the author's Convergence article, “Georg Cantor at the Dawn of Point-Set Topology.”

The complete project Topology from Analysis (pdf) is ready for student use, and the LaTeX source code is available from the author by request. A set of instructor notes that explain the purpose of the project and guide the instructor through the goals of each of the individual sections is appended at the end of the student project.

This project is the sixteenth in A Series of Mini-projects from TRIUMPHS: TRansforming Instruction in Undergraduate Mathematics via Primary Historical Sources appearing in Convergence, for use in courses ranging from first-year calculus to analysis, number theory to topology, and more. Links to other mini-PSPs in the series appear below, including six additional mini-PSPs on topics in undergraduate-level analysis and topology. The full TRIUMPHS collection offers a total of six mini-PSPs and two more extensive “full-length” PSPs for use with students of topology.

Acknowledgments

The development of the student project Topology from Analysis has been partially supported by the TRansforming Instruction in Undergraduate Mathematics via Primary Historical Sources (TRIUMPHS) project with funding from the National Science Foundation’s Improving Undergraduate STEM Education Program under Grants No. 1523494, 1523561, 1523747, 1523753, 1523898, 1524065, and 1524098. Any opinions, findings, and conclusions or recommendations expressed in this project are those of the author and do not necessarily reflect the views of the National Science Foundation. The author gratefully acknowledges this support, with special thanks to TRIUMPHS PI Janet Heine Barnett, who provided assistance with the historical content of the project.

References

Cantor, G. 1871. Über trigonometrischen Reihen. Mathematische Annalen 4:139–143.

Cantor, G. 1872. Über die Ausdehnung eines Satzes aus der Theorie der trigonometrischen Reihen. Mathematische Annalen 5(1):123–132.

Scoville, N. 2012, May. Georg Cantor at the Dawn of Point-Set Topology. Convergence 9. DOI:10.4169/loci003861.