Fourier's Heat Equation and the Birth of Modern Climate Science: A Mini-Primary Source Project for Differential Equations and Multivariable Calculus Students

The birth of modern climate science is often traced back to the 1827 paper "Mémoire sur les Températures du Globe Terrestre et des Espaces Planétaires" [Fourier 1827] by Jean-Baptiste Joseph Fourier (1768–1830). This work was of course not done in a vacuum, but rather beautifully combined Newton’s Law of Cooling with Euler’s work on differential equations, masterfully uniting ideas from his predecessors. The main ideas had been published five years earlier in Fourier’s groundbreaking study of heat, Théorie analytique de la chaleur [Fourier 1822]. The mini-Primary Source Project (mini-PSP) Fourier’s Heat Equation and the Birth of Climate Science walks the student through key points in that landmark work.

Designed for use in a differential equations course (but also suitable for use in multivariable calculus), the sections of this project tell the following story:

• Section 1. We see what Fourier’s starting assumptions were for his heat investigation. He essentially began with only Newton’s Law of Cooling!

• Section 2. We retrace one of Fourier’s primary examples: determining the temperature of a square prism of infinite length. Part of the way through, we find that Fourier snapped his fingers and solved a rather complicated-looking differential equation in just one step.

• Section 3. The magical incantation he used was some old magic due to Leonhard Euler (1707–1783). In this section, we present this technique in Euler’s own (translated) words, taken from the paper "De integratione aequationum differentialium altiorum graduum” [Euler 1743].

• Section 4. We return to the infinite square prism problem and apply Euler’s work to confirm Fourier’s solution.

• Section 5. We present Fourier’s more general heat equation and an intuitive explanation for why it holds.

• Section 6. We consider Fourier’s solution of the heat problem for an infinite rectangular solid—a geometric object that seems only slightly more complicated than a square prism of infinite length, but for which the century-old algorithm handed to him by Euler couldn’t simply be applied. Instead, Fourier’s work took a very surprising turn as he invented a whole new theory of infinite series, now called Fourier series.

• Section 7. Finally, students are briefly prompted to explore connections between Fourier’s work and modern climate science.

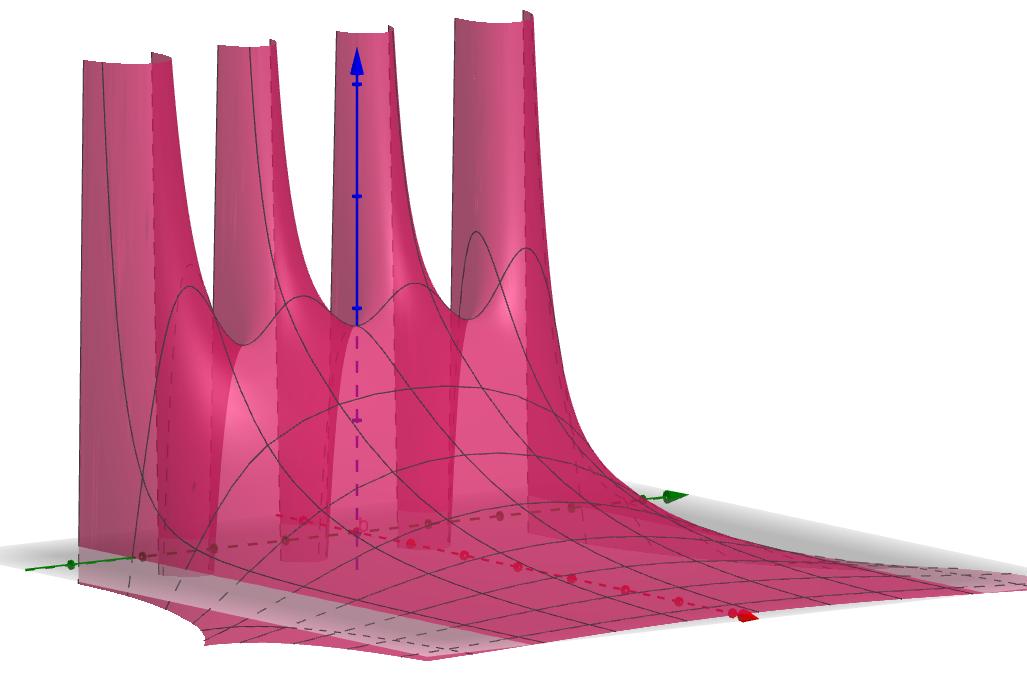
A graph of the four-term Fourier series expansion of the temperature surface
given by two parallel masses of ice with a mutually-orthogonal hot plate.
Image generated by the author using Geogebra 3D, freely available at https://geogebra.org/3d.

The project also provides a short biography of Fourier, who led a truly remarkable life. Born into a working-class family in Auxerre, France, he was orphaned at an early age. From there, he climbed his way all the way up to positions as prominent as the following: Founding faculty member at the École Polytechnique in Paris. Napoleon’s administrator of Egypt after its occupation by France in 1798. Prefect of the Department of Isère, where he led extensive infrastructure projects to quell chronic infections that were emanating from marshes in the area. Perpetual secretary of the Académie des Sciences. If there were ever an inspiration to students suffering from imposter syndrome as a result of humble origins, it’s Fourier!

Jean-BaptisteJoseph Fourier. Wellcome Library no. 3110i, public domain.

The complete project Fourier’s Heat Equation and the Birth of Modern Climate Science (pdf) is ready for student use, and the LaTeX source code is available from the author by request. Instructor notes are provided to explain the purpose of the project and guide the instructor through implementation of the project.

This project is the twenty-second in A Series of Mini-projects from TRIUMPHS: TRansforming Instruction in Undergraduate Mathematics via Primary Historical Sources appearing in Convergence, for use in courses ranging from first-year calculus to analysis, number theory to topology, and more. Links to other mini-PSPs in the series appear below. The full TRIUMPHS collection also offers dozens of other mini-PSPs and a similar number of more extensive full-length PSPs. These include a longer version of this mini-project (entitled Fourier’s Heat Equation and the Birth of Fourier Series) which, in addition to the content described above, takes the student for a joyride with Fourier by following him as he used his trigonometric series to prove a plethora of infinite series identities and briefly exploring how this work served as one impetus to future generations of mathematicians as they explored questions regarding rigor in analysis.

Acknowledgments

The development of the student project Fourier’s Heat Equation and the Birth of Modern Climate Science has been partially supported by the Transforming Instruction in Undergraduate Mathematics via Primary Historical Sources (TRIUMPHS) Project with funding from the National Science Foundation’s Improving Undergraduate STEM Education Program under Grants No. 1523494, 1523561, 1523747, 1523753, 1523898, 1524065, and 1524098. Any opinions, findings, and conclusions or recommendations expressed in this project are those of the author and do not necessarily reflect the views of the National Science Foundation.

References

Euler, Leonhard. 1743. De integratione aequationum differentialium altiorum graduum (On the integration of differential equations of higher orders). Miscellanea Berolinensia, 7:193–242. Eneström number 62. Written in 1742. Reprinted in Opera Omnia: Series 1, Volume 22, pp. 108–149. English translation by Alexander Aycock, Euler Circle-Mainz project, available at https://www.agtz.mathematik.uni-mainz.de/algebraische-geometrie/van-straten/ euler-kreis-mainz.

Fourier, Joseph. 1822. Théorie Analytique de la Chaleur (The Analytical Theory of Heat). Paris: F. Didot. English translation by Alexander Freeman in 1878, Cambridge University Press, Cambridge UK.

Fourier, Joseph. 1827. Mémoire sur les Températures du Globe Terrestre et des Espaces Planétaires (On the Temperatures of the Terrestrial Sphere and Interplanetary Space). Annales de Chimie et de Physique, XXVII:136–167.