Helping Ada Lovelace with her Homework: Classroom Exercises from a Victorian Calculus Course – Problem 9

The integral \[\int \frac{dx}{\sqrt{A+Bx+Cx^2}}\] is more sophisticated still. Try to prove for yourself that, if \(C>0\), \[\int \frac{dx}{\sqrt{A+Bx+Cx^2}}= \frac{1}{\sqrt{C}} \log\left ( 2Cx+B+\sqrt{4C(A+Bx+Cx^2)} \right ).\] In his Calculus, letting \(A=0\), \(B=2a\), and \(C=1\), De Morgan deduced that, as he put it: \[\int \frac{dx}{\sqrt{2ax+x^2}}= \log \left( x + a + \sqrt{(2ax+x^2)} \right ) + \log ⁡2. \mbox{ (Omit the constant.) } \]

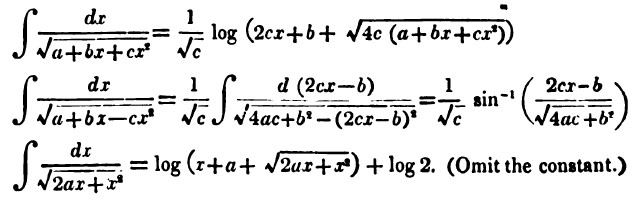
Figure 11. Part of page 116 from De Morgan’s Differential and Integral Calculus.

But when Lovelace tried to derive this result from first principles, she wrote [LB 170, 15 Aug. [1841], f. 115v]:

I cannot make it anything but \[\int \frac{dx}{\sqrt{2ax+x^2}} = \log \left ( x + 2a + \sqrt{(2ax+x^2)} \right )\] or else \[ = \log \left ( \frac{x}{2} + a + \frac{\sqrt{2ax+x^2}}{2} \right )+ \log 2⁡  \] \(\ldots\)  and I begin to suspect the book.

Her approach was straightforward. Setting \(2ax+x^2=(2a+x)x=y^2\), she derived the differential equation \((2a+x)dx=ydy\), from which she formed the integral \[\int  \frac{dx}{y}=\int \frac{dy}{2a+x}  .\]

Then, by analogy with the ‘fact’ that \(\frac{dx+dy}{x+y}=\frac{dx}{y}\), she obtained \[ \begin{array}{lcl} \int \frac{dx}{y} &=&  \int \frac{d(2a+x)+dy}{(2a+x)+y}\\ &=&\int \frac{d(2a+x+y)}{2a+x+y}\\ &=&\log⁡(2a+x+y)\\ &=&\log⁡(2a+x+\sqrt{2ax+x^2})\\ &=& \log⁡\left ( \frac{x}{2} + a + \frac{\sqrt{2ax+x^2}}{2}\right )+\log ⁡2.\end{array}\]

In addition to her erroneous assumption that \[\frac{dx+dy}{x+y}=\frac{dx}{y}\]

De Morgan was able to spot that, given \(y^2=(2a+x)x\), she had forgotten to apply the product rule, so that her differential equation should have been \[ydy=\frac{1}{2} xdx + \frac{1}{2} (2a+x)dx \] or   \[ydy=(x+a)dx.\,\,\,\,\,               \mbox{[2]}\] After correcting these errors, Lovelace wrote in a subsequent letter [LB 170, 21 Aug. [1841], f. 121v], that

we arrive then in my corrected paper, at \[\begin{array}{rcl}\int \frac{dx}{\sqrt{2ax+x^2}} &=& \log \left( x + a + \sqrt{(2ax+x^2)} \right )\\ &=&\log⁡\left( \frac{x}{2} + \frac{a}{2} + \frac{\sqrt{2ax+x^2}}{2} \right )+\log ⁡2.\end{array}\]

Can you derive Lovelace’s final result from equation [2]?

In his acknowledgement, De Morgan observed that Lovelace’s answer agreed with his result in all respects ‘but the log 2, which being a Constant, matters nothing’ [LB 170, 21 Aug. [1841], f. 121v]. Explain why his and Lovelace’s results are equivalent.

Return to Main Problems Page.
Continue to Problem 10.