Helping Ada Lovelace with her Homework: Classroom Exercises from a Victorian Calculus Course – Problem 10

Our final problem comes close to the end of Lovelace’s correspondence course with De Morgan. By early November 1841, she had progressed to the subject of second-order differential equations. One of the examples with which she had trouble was on page 156 of De Morgan’s Calculus. Given the nonhomogeneous equation

$$\frac{d^2 u}{d\theta^2 }+u=\cos⁡\theta$$

De Morgan gave its general solution as

$$u=C \sin ⁡\theta + C' \cos⁡ \theta + \sin⁡\theta \int cos^2 \theta d \theta - \frac{1}{2}  \cos⁡ \theta \int \sin ⁡2\theta  d\theta,$$ which, since

$$\int \cos^2 \theta d\theta = \frac{1}{2} \theta + \frac{1}{4} \sin 2 ⁡  \theta \, \,   \mbox{ and  } \,\, \int \sin ⁡2\theta  d\theta = -\frac{1}{2} \cos ⁡2\theta$$ resulted in

$$u=C \sin\theta  + C' \cos⁡ \theta +\frac{1}{2}\theta \sin⁡ \theta + \frac{1}{4}  \sin ⁡2 \theta  \sin⁡ \theta+  \frac{1}{4} \cos⁡\theta  \cos ⁡2\theta.$$ Using double angle formulae, he converted this into

$$u=C \sin⁡ \theta + C'  \cos⁡ \theta + \frac{1}{2}\theta \sin \theta+ \frac{1}{4}  \cos⁡ \theta,$$ which he then expressed in its final form as

$$u=C \sin⁡ \theta+C'  \cos⁡ \theta + \frac{1}{2} \sin⁡ \theta,$$ along with a challenge: ‘Explain this step?’ (See Figure 12).

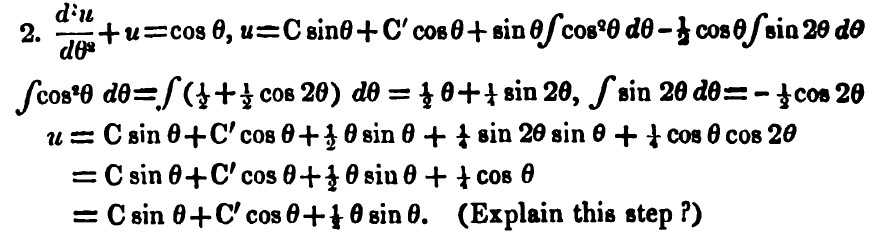
Figure 12. Problem from page 156 of De Morgan’s Differential and Integral Calculus.

The trouble was, as Lovelace remarked in a letter to De Morgan, ‘I cannot “explain this step”.’ She noted that ‘in the previous line, we have:   $$\begin{array}{r}  (1) \ldots u = C \sin⁡  \theta +C'  \cos⁡ \theta + \frac{1}{2}\theta  \sin⁡ \theta + \frac{1}{4} \cos \theta ⁡\mbox{ (quite clear)} \end{array}$$   $$\begin{array}{rcl}  (2) \ldots  \mbox{ And }  u &=&\cos⁡ \theta -\frac{d^2 u}{d \theta ^2 } \mbox{ (by hypothesis)} \\                                                             &=& \frac{1}{4}  \cos⁡ \theta+\left (\frac{3}{4} \cos⁡ \theta-\frac{d^2 u}{d\theta^2} \right ) \end{array}$$

whence one may conclude that $$C \sin⁡\theta+C'  \cos⁡ \theta + \frac{1}{2}\theta\sin⁡ \theta=\frac{3}{4}\cos⁡ \theta-\frac{d^2 u}{d\theta^2}$$ But how $u=C \sin⁡  \theta+C'  \cos⁡  \theta+\sin⁡\theta\cdot\frac{1}{2} \theta$ is to be deduced I do not discover.’ [LB 170, 4 Nov. [1841], ff. 132v-133r]

Not deterred, she tried again:

By subtracting $\frac{1}{4}\cos⁡ \theta$  from both sides of (1), we get $$u-\frac{1}{4} \cos⁡ \theta= C \sin⁡ \theta+C' \cos⁡ \theta+ \frac{1}{2} \theta \sin⁡ \theta$$ But unless $\frac{1}{4} \cos⁡ \theta=0$, (which would only be the case I conceive if $\theta=\pi/2$), I do not see how to derive the equation $\ldots$ [LB 170, 4 Nov. [1841], f. 133r].

De Morgan’s final solution is certainly correct. So can you explain why the $\frac{1}{4} \cos⁡ \theta$ mysteriously disappears?

Return to Main Problems Page.
Continue to Main Solutions Page.