A Euclidean Approach to the FTC - A Euclidean-style Statement of the FTC

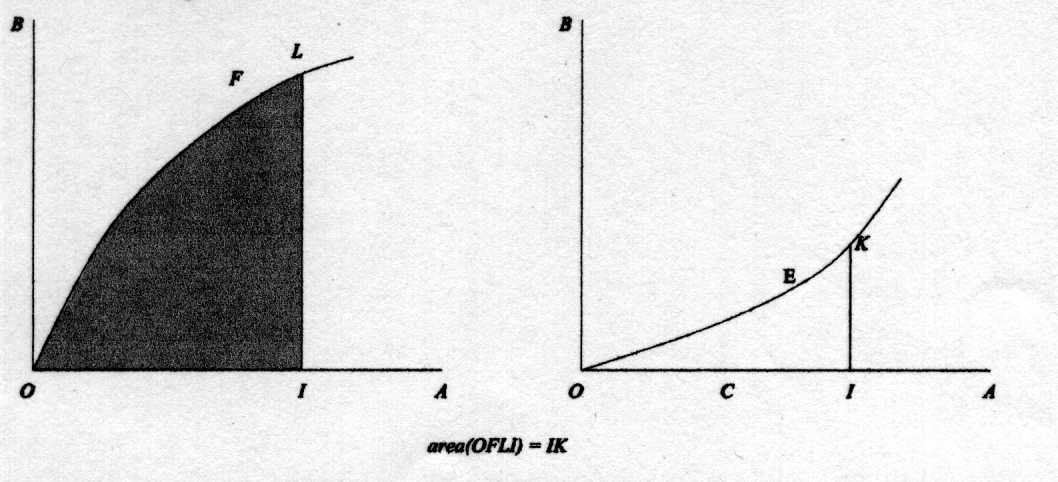
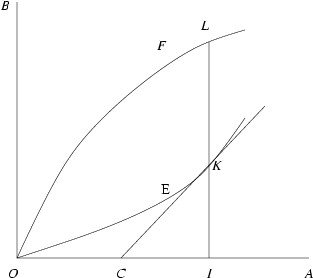
For us, the FTC is about a relation between a continuous function \(f(x)\) and its indefinite integral \(F(x) = \int^x_{x_0} f(t)\,dt\), so it may be surprising that Gregory was able to state the result without using the language of functions. The idea behind the alternative geometric approach is simply to focus on curves like those formed by the graphs of \(f(x)\) and \(F(x)\). In particular, instead of considering a function \(f(x)\) graphed on the \(x\)- and \(y\)-axes, suppose that \(OA\) and \(OB\) are line segments perpendicular to each other at the point \(O\) and that \(OFL\) is a curve that `passes the vertical line test', so that it can be thought of as formed by the graph of a function \(f(x)\). (Following the custom of many classical Euclidean geometers, Gregory denotes an arbitrary curve by three points chosen at random on the curve. Later on, these points will each take on a specific meaning.) Next, define another curve \(OEK\) point-wise as follows: at each point \(I\) on the line segment \(OA\) the length \(IK\) of the perpendicular line segment between \(OA\) and \(OEK\) is equal to the area of the curved region \(OFLI\).

In modern terms, the curve \(OEK\) is the graph of \(F(x) = \int^x_0 f(t)\,dt\). The FTC states that \(F'(x) = f(x)\) for each \(x\) in the interval. Since \(F'(x)\) is the slope of the tangent line to \(F(x)\) at \(x\), in geometrical terms this is equivalent to saying that for any \(I\) on the line segment \(OA\), if \(C\) is the point where the tangent line \(KC\) to the curve \(OEK\) at \(K\) intersects \(OA\), then the slope \(IK/IC\) of this tangent line equals \(IL\). The proof will be established by showing that this relation holds between the points \(C\), \(K\), and \(L\).