- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Wibold's Ludus Regularis, a 10th Century Board Game - Dice - Game Play
Wibold's Dice
Having established the various outcomes of rolling three dice and the corresponding virtues, Wibold described the design of the dice for his Ludus Regularis [2]:
Therefore because we have ordered the virtues and the numbers in a certain way, let us determine now in what manner they should arise by lot. Thus let us take three dice, which have six faces apiece, with various numbers inscribed – where the greatest is six (see note 7); the other remaining [faces] always one fewer, all the way to the ace, which taken together make 21 (see note 8); also, with our 5 vowels, namely A, E, I, O, U, let us decorate each [die] in the same order, so that the same [sequence of letters] repeated four times is set out upon each; and by continuing this process with a sustained and even course of movement, beginning at the ace there is written in the first place [on the first die] A, on the second E, the third I, always in direct sequence; and [the sequence] ends with the same letter [with which it began], thusly:
Thus having set forth these things, we desire that, by means of these three [dice], numbers be sought which are computed exactly as the lot will have dictated [and] always in its [designated] order, just as one is used to playing in the aforementioned dice game. Indeed we have omitted Υ, the sixth Greek vowel, not only because we so little require it within language and because we do not observe fully our rules of orthography, but because we desire of the work (see note 9), to profitably and commonly cultivate our sense of enjoyment.
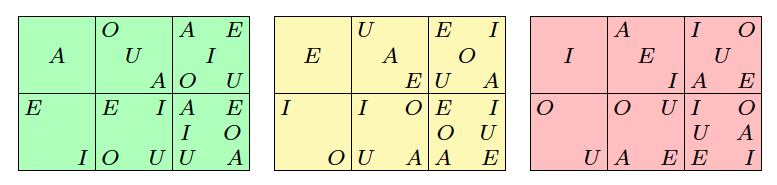
A second use of cyclic arrangements occurs in the construction of the cubical dice. The pips that appear on each face of the dice are composed of the vowels A, E, I, O, U. Wibold instructs us to write the sequence AEIOU over and over, forming from it in sequence blocks of length 1, 2, 3, 4, 5 and 6 to create the labeling of the faces of the first die, then we repeat the process to create the labeling of faces for the second and third dice.
|A|EI|OUA|EIOU|AEIOU|AEIOUA|⏟Die 1|E|IO|UAE|IOUA|EIOUA|EIOUAE|⏟Die 2
|I|OU|AEI|OUAE|IOUAE|IOUAEI|⏟Die 3 We may assume that these dice are constructed in the usual manner so that the sum of the pips on the opposing faces is always 7 (see note 10).
Wibold then proceeded to place consonants on another die. An inspection of Figure 1, Wibold's Table of Virtues, shows that the letter U represented a consonant (which today we write as V) whenever it did not appear as an initial letter of a word. This occurs, for example, in REUERENTIA. The letter U was both a vowel and a consonant in the Latin alphabet. Similarly, the letter I represented both the vowel and the consonant now written as J. Note, for example, IUSTITIA. He continues:
Seeing that there remain sixteen [letters] in the alphabet, all the rest are consonants, – with the exception of Q, which the grammarians call unnecessary, and which for that reason we have not counted, because absent its traveling companion, namely U, the letter loses its force, which can be very easily understood (see note 11); moreover, it is the seventeenth number for the completion, which we considered as though it were an extra [letter], – therefore in the same way it is proper to insert the vowels on a square [face], thus it is proper to insert the consonants on a single triangular pyramid, which has four faces, thus:

We see that Wibold omitted the consonant V and consonant I from the die. We conjecture that this is because both letters had been accommodated as vowels.
Game Play
Having constructed the dice, Wibold then described how to play the game:
By rule, what is held on the die, if it be any one of the consonants which has separated the vowels in the name of the virtue that is found underneath it, it is reckoned to stand for all the other [consonants]; moreover [whereas] what is on its square-side (see note 12) will be revealed on the upper face, here (see note 13) it is that which is on the lower [face], on its base.
. . . Thus when the cubes have been cast from the hand together with the triangle (sic), and with their letters individually inspected, then one will have recourse to the margin (see note 14), [where] the numbers [are] set adjacent to the virtues.
And in the same way as before, we should similarly record the separation of the three-fold [lot] into the individual vowels so found, which will be the virtue obtained alongside, its vowels having been made apparent; if we are successful in constructing [the virtue] with the vowels, and thus are in private possession of it, then partners playing with us may in no way prevail to claim the same one for themselves in the same game.
In any case, the dice in their whimsy, each by rolling and alternating in turn, [rendering] judgment, distribute all the virtues by lot; and if they [virtues] do not divide in mutually equal measure, those who will have gained more – even [just] one more – than the others should rejoice to have been granted preference with respect to the others.
Therefore as far as this is concerned, if it is necessary [to do so], then Charity, which is worth double, should be reckoned for two virtues; which furthermore, on account of the eclipse of just one letter, namely A, one should examine a second cast after the first – which we prohibit from happening in all other circumstances.
And until the sixth hour (see note 15) of that day, those [who are] more eminent, who call their lessers pupils, should urge that the virtues which are lacking to them by the lots, they should busy themselves to procure by good habits. Also the lessers, recognizing the equanimity (see note 16) of the dice, and just as . . . with preference being offered to them as tutors, so should they be prepared to call [the more eminent ones] teachers.
And if it should happen that no one is able to win over another, all those possessing virtues, with equanimity each in respect to another, then in charity alone they should always be united, and give thanks in the name of fraternity. – The same can happen in another more effective and pleasant manner perhaps for some [players], by which, with each of the three [cubical] dice only having been tossed, and referring back not to the power of the elements but to their sum taken together simultaneously, however many of this kind one obtains corresponding to [this] number, as has been inspected, he will boast to possess for himself [all those] virtues.
We propose these to you reader in such a way, that if what the pen completes, usefully you should adopt, or you should subtract by correcting; nevertheless you should promote no blasphemy; and if you are unwilling to approve, you must not disparage. Because if the game makes no sense [to you], or [if] it brings forth disgust from the soul, [then] at least the useful accurate matching of the numbers and the virtues, the playful investigation [of them], and [this] not idle exercise may convert [your] mind to it, so that you may have practice with the combination of numbers, and you may rejoice in the accumulation of virtues. In regard to what is to be acquired: if you win, [it is] not self-glorification, if you are conquered, you should not feel shame. For if you have gained less, [be] subject, if more, you should be kind to the one who is subject. To all those pursuing these virtues in Christ, cultivating [these] by their actions and habits, let there be grace and peace forever. Amen (see note 17).
During the play of the game, a player tries to acquire ownership of as many virtues as possible.
We assume that the four dice are placed in a cup and shaken out. In order to acquire a virtue four conditions must be met:
- The number of vowels on each of the three cubical dice taken as an ordered triple identify a unique virtue, as found in Table 1;
- the vowels appearing as pips on the exposed faces of the dice must include all those in the name of the virtue;
- at least one of the consonants appearing on the bottom face of the tetrahedral die must be included in the name of the virtue;
- the virtue must not have been previously acquired by another player.
For example, suppose a player has rolled (1,2,3) in the form {A, IO, AEI}. This leads the player to find PAX on the game board for which he has the vowel A. If the tetrahedron fell face down on either MNPR or on STXZ, the player would then own PAX – if it is still in play. We then suspect he would place a token in the spot on the game board to mark his acquisition.
Let's consider another example. To gain GAUDIUM, the player must roll a (2,2,3) in which are found the vowels A, I, U and U. Now there are three ways to produce (2,2,3): {EI, IO, AEI}, {EI, OU, UAE} or {IO, OU, OUA}. However, only two of these meet the vowel condition, the first of these not being viable. Of course, a consonant among G, D, and M must be cast on the bottom face of the tetrahedral die as well.
Play continues until all virtues have been acquired. Each player in turn is allowed to roll the dice and acquire another virtue.
Although not mentioned explicitly in the rules of the game, it seems that Wibold's own aesthetic made it desirable to him that a player acquire complementary virtues. Recall how he associated virtues together when their total point values summed to 21. Continuing with our example, if the player had acquired PAX with its value of 6, the player would also wish to acquire a virtue with value 15: CONFESSIO, SUSPIRATIO, or HILARITAS. This form of play would be natural for the players if Wibold had in mind a game board in the form of Table 6, as opposed to that of Table 5, in the section, Possible Game Boards, later in this article.
The cleric possessing the most virtues wins. If it should happen that a tie occurs, the cleric possessing KARITAS has his total incremented by one. This need not, however, determine a unique winner since the player with KARITAS might not be one of the tied players. If a tie cannot be broken, Wibold urged the players to rejoice in the outcome.
The outcome (1,1,1) corresponding to KARITAS can be obtained in exactly one way: A, E, I. In this one instance, Wibold permitted a second cast of the dice in order to attempt to obtain a second A to satisfy the vowel condition so as to acquire this most important virtue.
A similar problem occurs with IUSTITIA, the outcome (1,1,4), for which we need A, I, I, I, and U. There are three ways to obtain this pattern: {A, E, OUAE}, {A, I, IOUA}, and {E, I, EIOU}, none of which satisfy the condition required. Either Wibold made an error or he chose to ignore the initial I that here plays the role of a consonant just as he chose to ignore the consonant V. It may be verified that no other virtue has a similar difficulty in being able to satisfy the requisite conditions.
In order to appreciate the play of this game, an applet simulating the game is available here: Play the Game! The reader is invited to experiment with how Wibold's game parcels out the virtues.
The last paragraph of the passage above includes a simplified set of rules of the game presented by Wibold. Under these rules it suffices to examine the total of the three dice, the player acquiring all virtues corresponding to that sum. The game ought to proceed very quickly when using these simplified rules since a player can win up to six virtues on any one roll (see Table 2).
Notes
7. The Latin actually has where the greater is six [quae major senarius].
8. That is, 1+2+⋯+6=21.
9. By "the work," Wibold meant the game he was designing.
10. We mention also that such dice may be constructed to be either left- or right-handed as well.
11. Inspection of Table 1 will show that no virtue is spelled with the letter Q, which justifies Wibold's omission of this symbol from the labeling of the die. (As an aside, we note that one die in the modern word game Boggle is actually printed with the digraph QU rather than with a single letter.) Furthermore, the letter K, borrowed from the Greek alphabet, was used by Latin users only as the initial letter of a very small number of words and had long before Wibold's time fallen into disuse. It is interesting that Wibold chose the odd spelling KARITAS for the first in the list of virtues as this allowed the use of K in the game.
12. That is, the square-sided face of the cubical die.
13. On the tetrahedral die.
14. It seems the reference is to Table 1 or, more likely, to a game board.
15. The sixth hour is noon local time.
16. At this point the manuscript reads niati, not a known word, for which all editors make conjectures as to meaning. Colvener recommends aequitatem as supplying the most natural meaning. Indeed, all manuscripts are corrupt at this point and where the ellipsis appears later in the line.
17. The final Amen which appears in [2] is omitted in [4].
Richard Pulskamp (Xavier University) and Daniel Otero (Xavier University), "Wibold's Ludus Regularis, a 10th Century Board Game - Dice - Game Play," Convergence (July 2014)





