The Rule of False Position and Geometric Problems - Introduction

Editor's note: This article was published in 2006.

Historical examples in college mathematics courses can help students understand the process of creation in mathematics and how mathematicians have grappled with problems over the ages. This can enliven mathematics and humanize it [1].  Swetz proposes to have students solve some of the problems that were of interest for early mathematicians as a direct approach to enrich mathematics teaching and learning through history [7]. Historical remarks can help students understand the material better and help them see how it fits into the wider domain of mathematics [5]. Historical examples of problems can also provide an interesting background to tell the history of mathematical ideas [4]. Future teachers can benefit especially from the historical perspective. They will learn solution methods alternative to the ones usually taught in schools. Let us look at a problem Simon Stevin published in 1583 [6].

Problem:  Construct a square knowing the difference PQ between its diagonal and its side.

Solution: Let BCDE be any square. On the diagonal EC take the point F so that EF = ED.

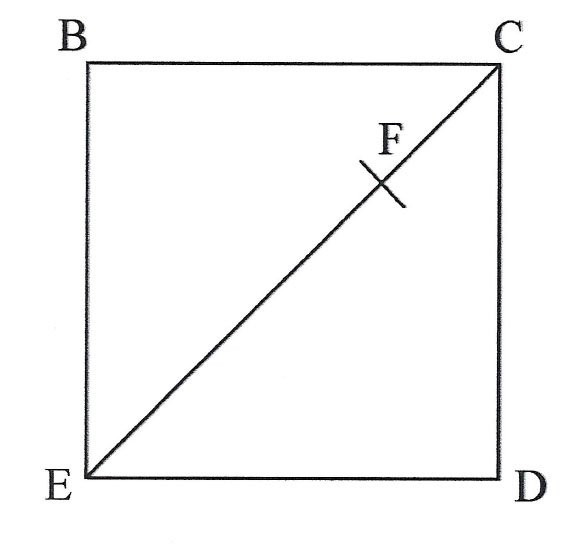
If FC = PQ, then the square BCDE is the solution to the problem. If not, the side of the solution square (say y) will be the fourth proportional with respect to the segments FC, PQ and ED. That is, FC:PQ = ED:y.

The method used by Stevin is called the rule of false position. The solution is especially interesting because he used this method in a geometrical problem; the method was mainly used in problems of algebraic nature. It is also interesting because our students do not use geometrical proportionality when they face problems about constructing figures that have some restrictions. To solve the problem of the square and its diagonal, students in high school (ages 16-18) frequently use methods involving trigonometry and algebra to compute the side of the square. For them the real challenge is to construct the figure.