Oliver Byrne: The Matisse of Mathematics - Biography 1860-1872

Byrne and his wife left the United States before the outbreak of the Civil War in 1861.⁶⁷ Fifty years old and back in London, Byrne touted his⁶⁸

two new mathematical sciences, not yet known … the ‘Calculus of Form’ and ‘Dual Arithmetic.’ Since I arrived [in London] I have been very ill and am now quite penniless, after having laboured and studied so hard my entire life …. These two are my greatest works, but it may take me some time to get them published so as to make them profitable to myself.

Byrne first mentioned The Calculus of Form in a revised version of an article that first appeared in the Civil Engineer and Architect's Journal in 1847.⁶⁹ In the revised version of this article later printed in Miscellaneous Mathematical Papers, Byrne touted his “new work on the Calculus, about to be published.”⁷⁰ An advertisement in Miscellaneous Mathematical Papers announced The Calculus of Form already in press. In his 1849 work on logarithms, Byrne cited specific page references from The Calculus of Form. In a somewhat later publication, Mechanics: Their Principles and Practical Applications, Byrne stated concerning The Calculus of Form:⁷¹

a very large work was printed on this new science in London, but was suppressed through conspiracy, the particulars of which we have not space to enter into here.

Figure 7. First page of The Calculus of Form © The British Library Board, C.40.i.5.

Byrne’s widow Eleanor donated a printed copy of The Calculus of Form to the Trinity College Library, Dublin. The Trinity College copy includes no use of color, although Byrne may have intended to use color if the book had been published. The British Library reports that the whole edition was cancelled except two copies.⁷²

In The Calculus of Form, Byrne viewed derivatives of geometric forms shrinking in size (going down a dimension), and integrals of geometric forms expanding (flowing) into one higher dimension. However, he also discussed the standard limit definition of the derivative. In the second author's 44 years of teaching and studying Calculus, he has used the same metaphors of going up and down one dimension for integration and differentiation. In fact, Isaac Newton called derivatives fluxions (changing by shrinking one dimension) and integrals fluents (flowing up one dimension). The second author does not think Byrne's work offers anything really new about teaching Calculus. However, it is helpful for many students to visually explain differentiation and integration using the fluxion and fluent approach, using movement (shrinking down a dimension and flowing up a dimension) to give a geometric and dynamic explanation of Calculus. The Trinity College collection includes a manuscript copy of Byrne’s Trinal Calculus, a work to which he sometimes referred during his later years and which appears to be the last text that he worked on.

Byrne contended that the new art of dual arithmetic “supersedes the use of all sorts of logarithms, and gives results with greater accuracy and precision than any other set of numbers and with less labour.”⁷³ In this work, published in 1863, Byrne proposed a new method of performing faster calculations that involved breaking numbers down into the form “a(1.1)^b(1.01)^c(1.001)^d…” and then operating on the quantities a, b, c, d, etc. to find the answer. Byrne contended that his method was superior to the use of logarithms for computations. The second author owns a copy of Dual Arithmetic, and finds that Byrne's method of dual arithmetic computational shortcuts is more laborious than using logarithms to simplify computations.

Figure 8. Title page of Oliver Byrne's Dual Arithmetic (from the book in the collection of Sid Kolpas)

On 19 January 1866, Byrne, along with Rev. Walter Mitchell, gave a lecture at the Royal United Service Institution entitled, “The New Science of Dual Arithmetic Applied to Naval and Military Calculations.” After the lecture, the chairman commented,⁷⁴

Mr. Byrne has thrown down the gauntlet, and such immense consequences in every department of mathematical science are involved, that the system ought to be fully tested . . .. The system is easy to be comprehended by the lowest intelligence.

However, Augustus DeMorgan, in A Budget of Paradoxes, was derogatory in his judgment of dual arithmetic. DeMorgan implied that it showed the lengths to which some mathematicians would go in attempting to ease the labor of doing arithmetic. Unlike many of Byrne’s engineering and practical texts, Dual Arithmetic: A New Art, was not a bestseller. See Janet Barnett's article, “The Dual Arithmetic of Oliver Byrne: A New Art which entirely supersedes the use of logarithms”  (2011), for further analysis of the “dual arithmetic” works of Oliver Byrne.⁷⁵

A gas explosion tragedy struck at the Byrne residence on Tollington Road, Holloway, London in 1864. “Terrific Explosion of Gas at Holloway,” a local newspaper reported,

Mr. Byrne on entering the front parlour with a light to see if all was safe before retiring was suddenly thrown down, the light having come in contact with the gas, which had been allowed to escape from the chandelier.

Both Byrne and his wife, Eleanor, were severely burned on the face and hands. On Byrne’s next Royal Literary Fund (RLF) application, his witness notes, “Mr. Byrne makes his mark in my presence, his hands having been severly [sic] burnt.”⁷⁶ Years later Eleanor developed blindness attributed to the explosion.⁷⁷

Soon after the explosion, the Byrnes moved to Upper Sydenham in the Parish of Lewisham, England, near their latest publishers, the Spons.⁷⁸ According to an 1891 obituary of the engineer and explosives expert Ernest Spon, Spon “was educated as a Civil Engineer for upwards of three years, from 1869 to 1872, in the office of the late Mr. Oliver Byrne.”⁷⁹ Spon’s uncles, Edward Spon and Francis Nicolas Spon, published at least two of Byrne’s books in 1867 and 1868 and two publications for Byrne’s wife, Eleanor Rugg Byrne, in 1869 and 1870.⁸⁰ Byrne edited Spons’ Dictionary of Engineering, containing mechanical, military, and naval applications, with technical terms in French, German, Italian, and Spanish, with the first volume published in 1869.⁸¹

Despite the success of Spons’ Dictionary, Byrne did not enjoy the fruits of his labor. In his November 1872 RLF application, Byrne complained,

I have been engaged, during the last four years and a half, compiling and editing a Dictionary of Engineering … consisting of 1952 pages which contain upward of 4000 engravings…. The publishers E. & F. N. Spon, without the slightest notice, refused, on the 29th of July last, to pay me any more money and they furnished me with an erroneous account, to make it appear, that I was overpaid. … To avoid paying me the royalty agreed upon; and to get the Dictionary into their own hands … they have involved me in Law … may God protect me, I am in bad hands.

After receiving the RLF grant, Byrne wrote in his letter of thanks,⁸²

It would be impossible for me to overrate the value of aid at peculiar umbilicus points in my simple career. I do not like to use harsh words, although I have been often in the hands of robbers, but, never before this time, was I in the clutches of the thugs of trade and the smeddumtails [slime] of society.

The Spons themselves, in a Preface to Volume 8 of the Dictionary, reported only that, "Until August, 1872, the editorial department was conducted by Mr. Oliver Byrne, assisted by Mr. Ernest Spon; at that period Mr. Byrne ceased to be editor, and the work has been completed under the direction of Mr. E. Spon." The title page of each volume of the Dictionary recorded “edited by Byrne and Spon,” with publishers E. and F. N. Spon. E. & F. N. Spon, later known as Spon Press, persisted as a London publisher of building, construction, and civil engineering books until Taylor & Francis purchased the company in 1998.⁸³

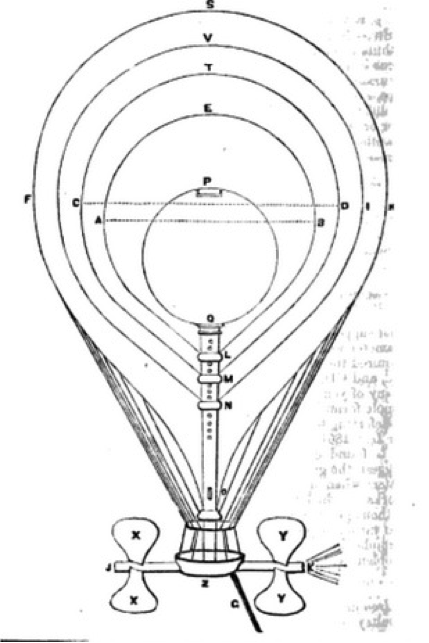
Figure 9. Eleanor Rugg Byrne's balloon in her article, “Safety Concentric Balloon,” The Civil Engineer and Architect’s Journal, 25:154-155 (May 1862).

Byrne’s wife, Eleanor Rugg Byrne, was an innovator and author like her husband. Her 1862 article, “The Safety Concentric Balloon,” in the Civil Engineer and Architect’s Journal, discusses a design for an innovative balloon that removes “all dangers while in the air” and extends “the art [of recreational ballooning] by a new guiding principle.” Her balloon design used a series of concentric interior balloons, the innermost of which was made of a strong material and permanently charged with gas. The innermost balloon was not connected to the basket containing occupants and was used to support the steering and propelling of the balloon. The diagram shows a center metallic balloon, surrounded by four other balloons made of flexible material. The intent of the balloon was to increase the safety of recreational ballooning. It is unknown whether the balloon was ever manufactured.⁸⁴ Her interest in ballooning may have resulted from her meteorological pursuits.

Figure 10. One of Eleanor Byrne’s meteorological publications. (This image is used by kind permission of the Syndics of Cambridge University Library.)

In 1869-1870 Eleanor published two books dealing with weather prediction based on observed patterns of historical seasonal variations, the wind, and the phases of the moon. Her weather prediction techniques were primitive by modern meteorological standards. She discussed how “the moon acts on the aqueous or vaporous qualities of the atmosphere in a manner similar to that in which she acts upon the sea.” Eleanor indicated that “the moon has mountains, atmosphere, seas, rivers, and lakes, like those we observe on the earth.” She estimated the earth's atmosphere at nearly 50 miles high.⁸⁵ According to Wikipedia, the boundary of our atmosphere with outer space measures 62 miles. It is interesting, and not surprising, to note that Eleanor's books were published by Spon, a publisher for whom her husband, Oliver Byrne, worked.

67 RLF application (4 November 1861). Byrne letter dated 5 November 1861 uses 33 Argyle Street, Kings Cross, London address.

68 RLF application (4 November 1861).

69 Oliver Byrne, “A New Theory of the Earth, that Fully Accounts for Many Astronomical, Geographical, and Geological Phenomena, Hitherto Unaccounted For,” Civil Engineer and Architect's Journal, 10:99-101 (April 1847) and 10:133-134 (May 1847).

70 John Byrne, The Miscellaneous Mathematical Papers of Oliver Byrne (London: Maynard, 1848), 5.

71 Oliver Byrne, Mechanics: their principles and practical applications (New York: De Witt & Davenport, 1853), 95.

72 Explore the British Library (http://explore.bl.uk : accessed 28 July 2015); entry for “The Calculus of Form. [By Oliver Byrne.],” Unique Identification Number BLL01000569301.

73 RLF application (4 November 1861).

74 Journal of the Royal United Service Institution (London: W. Mitchell and Son, 1867), 10:61.

75 Janet Heine Barnett, “The Dual Arithmetic of Oliver Byrne: A New Art which entirely supersedes the use of logarithms,” Proceedings of the Canadian Society for the History and Philosophy of Mathematics: Thirty-sixth Annual Meeting (Dublin), 24:12-30 (2011).

76 RLF application (7 February 1864). Byrne’s 1864 Royal Literary Fund application contains a newspaper clipping entitled, “Terrific Explosion of Gas at Holloway.” Byrne was registered in 1865 to vote at this address (Byrne, Oliver, 21 Tollington Road) in “London, England, Electoral Registers, 1832-1965,” Ancestry (http://www.ancestry.com : accessed 30 July 2014).

77 RLF application (28 June 1880).

78 “1871 England Census,” Kent, Lewisham, Sydenham, p. 26, Oliver Byrne; Ancestry (http://www.ancestry.com : accessed 2 August 2014); citing Census Returns of England and Wales, 1871 (Kew, Surrey, England: The National Archives of the UK, Public Record Office, 1871).

79 Ernest Spon, death; Minutes of proceedings of the Institution of Civil Engineers (Great Britain: Institution of Civil Engineers, 1891), 315-316.

80 Oliver Byrne, The Essential Elements of Practical Mechanics, based on the principle of work; designed for engineering students (E. & F. N. Spon: London, 1867). Also Oliver Byrne, General Method of Solving Equations of all degrees; applied particularly to equations of the second, third, fourth, and fifth degrees (London: E. & F. N. Spon, 1868). For Eleanor Rugg Byrne, A Short Philosophical Treatise on the Atmosphere, and the Influence of the Changes of the Moon on the Weather; and the Variations of the Weather Represented by Weather Indicators, from the First of July 1869, to the First of January, 1870, to Which is Subjoined Interesting Matter (London: E. & F. N. Spon, 1869) and The Useful Weather Guide, for the First Six Months of the Year 1870 (London: E. & F. N. Spon, 1870).

81 Oliver Byrne ed., Spons’ Dictionary of Engineering, containing mechanical, military, and naval applications, with technical terms in French, German, Italian, and Spanish (London: E. & F. N. Spon, 1869).

82 RLF application (2 November 1872). For definition of “smeddumtails" as "ore sludge" or "ore slime,” as someone from a mining town would know, see http://www.mindat.org/glossary/smiddum_tails.

83 "Taylor & Francis Timeline" (http://www.ulib.niu.edu/publishers/TaylorFrancis.htm : accessed 28 July 2015).

84 Eleanor Rugg Byrne, “Safety Concentric Balloon,” The Civil Engineer and Architect’s Journal, 25:154-155 (May 1862).

85 Eleanor Rugg Byrne, A Short Philosophical Treatise on the Atmosphere, and the Influence of the Changes of the Moon on the Weather; and the Variations of the Weather Represented by Weather Indicators, from the First of July 1869, to the First of January, 1870, to Which is Subjoined Interesting Matter (London: E. & F. N. Spon, 1869). Secondly, Eleanor Rugg Byrne, The Useful Weather Guide, for the First Six Months of the Year 1870 (London: E. & F. N. Spon, 1870).