- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Geometrical Representation of Arithmetic Series – Terminology and Trapezia
The systematic study of series, śreḍhī-vyavahāra (literally "series-practices") in Sanskrit, forms part of texts of arithmetic and mensuration called pāṭīgaṇita. Here we will consider finite arithmetic progressions where the difference between two consecutive entries is always the same.
To explain the historical descriptions, we will use modern notations when necessary. We note that the original texts contain no mathematical symbols and descriptions are given entirely in words. Thus the sum of a series is described as follows (Pāṭīgaṇita, v. 85.1):
The common difference multiplied by one-half of the number of terms minus one, increased by the first term, then multiplied by the number of terms, gives the sum of the series.
The Sanskrit terms used here are ādi for the first term, caya for the common difference, pada for the number of terms, and gaṇita for the sum. Rewritten in terms familiar to us: a generic arithmetic series containing n terms, beginning with a first term a and increasing by a common difference d, has the sum Sn, given by Sn=((n−12)d+a)n. We now recognize the familiar result!
Associated to a series S, we will describe a geometric figure whose total area equals Sn and whose partial areas equal the partial sums of the series (the units are neglected, since an area and a sum have necessarily different units). Such a figure is known as śreḍhī-kṣetra, which literally means “series-area.” Notice the following comparison, which brings to mind our teacup (Pāṭīgaṇita, v. 79):
As for an earthen pot [śarāva in Sanskrit], the width of the base is smaller and the top larger, so is it for a series-area.
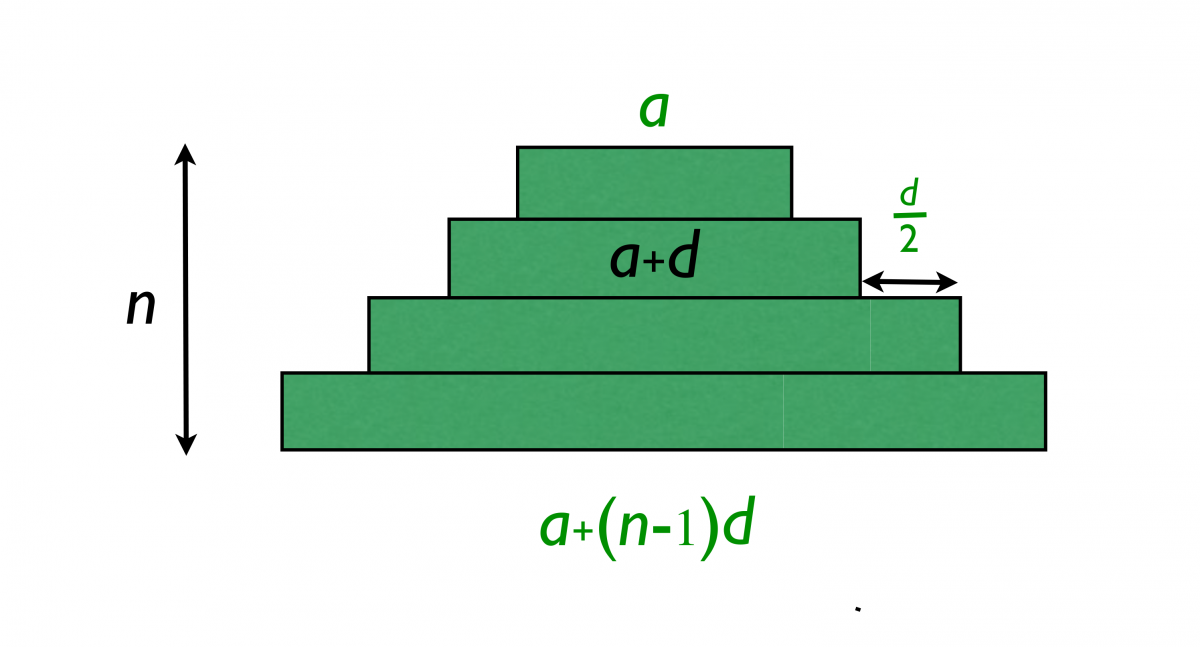
Figure 5. Geometric figure representing the sum of an arithmetic progression, or “series-area”
If we geometrically represent each term of the series by a rectangle with the same length as that term and with breadth 1, then the sum of the areas of the pile of rectangles would give the sum of the series. The representation in Figure 5 looks more like the front view of a staircase than a regular geometric figure.

Figure 6. Staircase in Lille, France
But what happens when we rearrange the rectangles a little? Imagine inverting the figure (so it looks more like an earthen pot or teacup) and smoothing it by drawing a line to remove the protruding parts (green) of the rectangles on the right and pasting these “extra” triangles on the other side (red), as shown in Figure 7. Now we get a trapezium with the same area as that of the staircase.
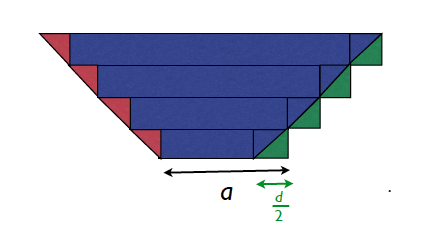
Figure 7. “Staircase” shape inverted and smoothed into a trapezium

Figure 8. Trapezium with area equal to the sum of the arithmetic progression
Furthermore, each term of the series, beginning with the first, is represented as the area of a trapezium. The following text describes this correspondence for the simplest case, i.e. when there is one term (Pāṭīgaṇita, v. 81).
The number of terms which is one is the altitude [lamba] of the series-figure, the first term of the series diminished by half the common difference of the series is the base [dharā], and [the base] increased by the common difference is the face [vaktra].
Let b, f, and h be the base (lower edge in Figure 8), face (top edge in Figure 8), and altitude (or height) of the figure. Then b=a−d2 and f=b+d=a−d2+d for h=n=1. This is precisely what we have obtained and the trapezium obtained does resemble a two-dimensional teacup!
We emphasize the fact that these descriptions were written in verse with strict metrical rules (lost in translation, alas!) meant to be learnt by heart. Thus they are cryptic and more “rules” than “proofs.” The reader must convince himself that these rules are indeed valid.
For instance the rearrangement we described above (which is a reconstruction of how the ancients might have argued) can be symbolically written as
| Sn | =a+(a+d)+⋯+(a+(n−1)d) |
|
=(a−d2)+d2+(a+d−d2)+d2+⋯+(a+(n−1)d−d2)+d2. |
To each term of a given series is associated a rectangle (in blue) with height 1 and two triangles (in red) the sum of whose areas is the corresponding term of the series. To obtain the area of the series-figure we use the following simple fact (Pāṭīgaṇita, v. 85.2):
The area [phala] of the [corresponding] series-figure is equal to the product of half of the sum of the base and face, and the altitude.
This is the familiar formula for the area of a trapezium. Thus, when the base is a−d2, the face a−d2+nd, and the altitude n, we get the area 12(a−d2+a−d2+nd)n=(a+(n−1)d2)n=Sn. The area of each rectangle with its two triangles gives the corresponding term of the series. Thus the lowest rectangle of length a−d2 and height 1 is a−d2. The sum of the areas of the two triangles is d2 and these two areas add up to give a, the first term of the series. The area of the trapezium above the lowest one is 12(a+d2+a+3d2)=a+d, the second term of the series, and so on.
Notice that if we used the first term of the series as the base and the nth term as the face of the trapezium, its area would still give the sum of the n terms but the previous terms would no longer correspond nicely to its subsections.
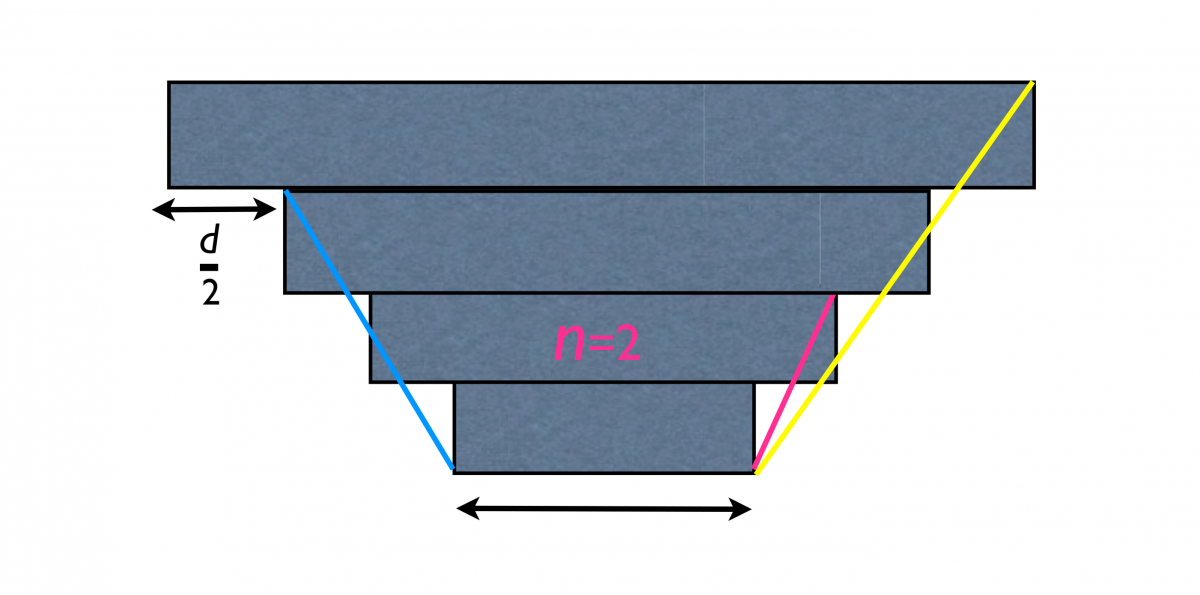
Figure 9. Series-area, or series-figure, with base (of length a) not reduced
Gautami Bhowmik (Université de Lille), "Geometrical Representation of Arithmetic Series – Terminology and Trapezia," Convergence (December 2015)





