Oliver Byrne: The Matisse of Mathematics - Using Byrne's Euclid in a Geometry Class

The following section of this article will discuss how Byrne's The First Six Books of the Elements of Euclid in Which Coloured Diagrams and Symbols Are Used Instead of Letters for the Greater Ease of Learners might be used in the classroom as a supplement to a geometry lesson, as an inspiration for using color-coded diagrams within an otherwise traditional geometry lesson, as a source for student geometry projects, or as a topic of discussion in the study of mathematics history and/or pedagogy.

Byrne’s Euclid uses little or no labeling of the diagrams and no algebraic arguments. It is primarily geometric, using appropriate bold primary colors to indicate the various parts of each diagram associated with a theorem to be proved. Therefore, Byrne’s proofs are the type of argument that Euclid used in his Elements, almost purely geometric in nature. Instructors teaching plane geometry at any level can use pages from Byrne’s Euclid, and require students to give the reasons for each step of the proof. In fact, students could also construct their own color-coded proofs using Byrne’s methods; in the 1970s, Sid Kolpas assigned each of his ninth grade Geometry students a different theorem, and had them create their own color dissection proofs similar to Byrne's. These proofs were displayed on the walls and windows of the classroom, and were used for periodic review throughout the semester. The Pythagorean Theorem and the Vertical Angles Theorem were chosen as the following two examples.

Oliver Byrne’s proof of the Pythagorean Theorem, Proposition XLVII of Book I of Euclid’s Elements, is a comprehensive color version of Euclid’s classic “Windmill” proof. Great care was taken in isolating various parts of the figure by choosing appropriate colors to aid in understanding the proof. According to Byrne's preface, careful choices of tint and form, and the isolation of parts of the diagram, help to better impress knowledge in the student’s mind.

Typically, today's geometry teachers use a simpler, similar triangles approach to prove the Pythagorean Theorem after the properties of similar triangles and proportions are established. Byrne’s color proof, a “proof without words” (or at least very few words!), could also be used to show students how Euclid proved it in his Elements. One should ask students to supply reasons, in words, for each step in Byrne’s color version of Euclid’s proof. Or they can devise their own color proof of whatever method they choose to prove the theorem.

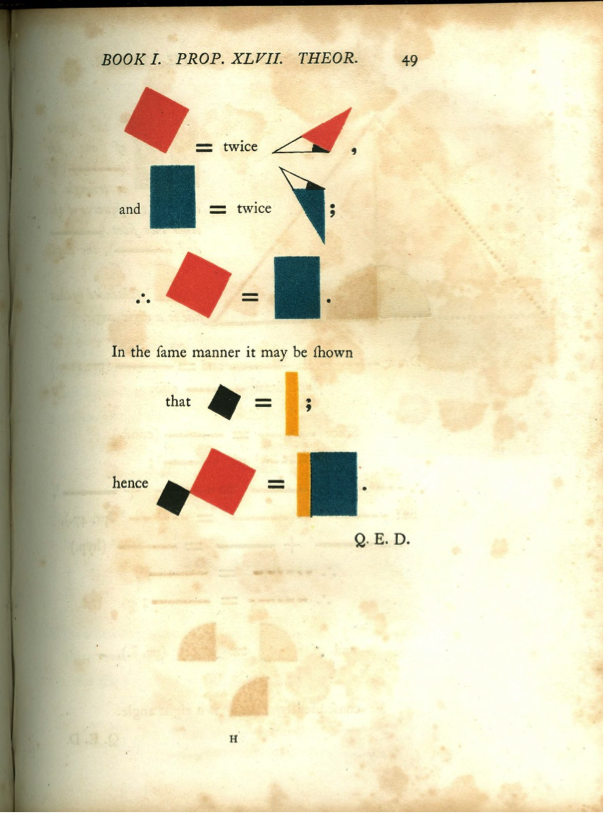
Figure 16. Byrne's proof of the Pythagorean Theorem (Book I, Proposition 47 of Euclid’s Elements). (Images from the book in the collection of Sid Kolpas)

Referring to Byrne’s proof, students typically come up with reasons similar to the following for each step (adapted from Kolpas' The Pythagorean Theorem: Eight Classic Proofs¹¹³):

• Construct blue and black squares on the legs, and a red square on the hypotenuse of the triangle.
• In a plane, through a point outside a given line, only one line can be drawn parallel to a given line; these are the dotted lines. Also, through two points exactly one line can be drawn. These are the solid black lines.
• All right angles are equal; these are the yellow angles. Therefore, by the addition property of equality, the overlapping obtuse angles (yellow and black) are equal.
• All sides of a square are equal. Therefore, the triangles isolated in the proof are congruent by side-angle-side.
• In a plane, if a line is perpendicular to each of two lines, then the two lines are parallel. Therefore, the solid yellow line is parallel to the dotted black line.
• The square on the longer leg and the triangle that overlaps it share a common base and altitude, so the area of the square is twice the area of the triangle. (If a parallelogram and a triangle share a common base and are contained in a common set of parallel lines, then the area of the parallelogram is twice the area of the triangle). The same relationship holds for the larger (blue) rectangular piece on the hypotenuse and its overlapping triangle.
• By substitution, the area of the square on the longer leg equals the area of the large blue rectangular piece on the hypotenuse.
• A similar argument can be used to demonstrate that the area of the square on the shorter leg equals the area of the small yellow rectangular piece on the hypotenuse. I ask students to supply this argument.
• By the addition property of equality, the sum of the areas of the squares on the legs of a right triangle is equal to the area of the square on the hypotenuse. Symbolically, if the legs are of lengths \(a\) and \(b,\) then the hypotenuse is of length \(c,\) where \(a^2+b^2=c^2.\)

Peter Lynch noted that "The Red and Blue Chair" designed in 1917 by Gerrit Rietveld has striking similarities to the color diagram used in Byrne’s proof of the Pythagorean Theorem.¹¹⁴

Figure 17. Red and Blue Chair, by Gerrit Rietveld, 1917 (Creative Commons License)

Another example is Byrne’s color proof of the theorem that if two lines intersect, vertical angles are equal (Euclid’s Elements, Book I, Proposition XV).

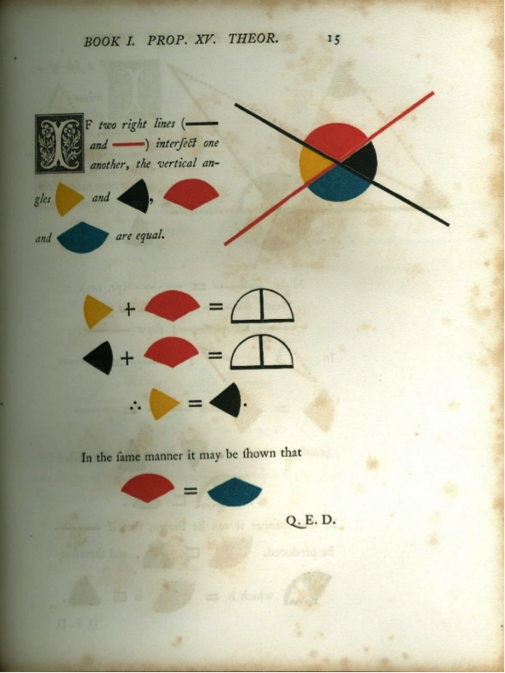
Figure 18. Byrne's proof of the Vertical Angles Theorem (Book I, Proposition 15 of Euclid’s Elements). (Image from the book in the collection of Sid Kolpas)

One should ask students to supply reasons, in words, for each step in Byrne’s color version of Euclid’s proof. Or they can devise their own color proof of whatever method they choose to prove the theorem.

Students typically come up with reasons similar to the following for each step:

• We are given a black line intersecting a red line.
• The yellow acute angle and the red obtuse angle add to a line (they are supplementary, or they add to half of a circle or 180 degrees or, as Euclid would say, "two right angles").
• The black acute angle and the red obtuse angle add to a line (they are supplementary, or they add to half of a circle or 180 degrees).
• The yellow acute angle plus the red obtuse angle is equal to the black acute angle plus the red obtuse angle since they are both equal to 180 degrees.
• Subtracting the red obtuse angle results in the yellow acute angle being equal to the black acute angle.
• A similar argument can establish the fact that the red obtuse angle is equal to the blue obtuse angle. I ask students to supply this argument.

Pages from Byrne’s Euclid would also make for interesting pedagogical discussions in Mathematics Education classes of the use of color in teaching mathematics and of the strategy of isolating parts of a mathematical diagram. Oliver Byrne’s colorful work changed the second author’s teaching of high school Geometry when he was first introduced to it in a History of Mathematics class in 1970. Because of its influence on his teaching, he always used colored chalk and color coded isolations of the appropriate sections of the geometric argument, along with appropriate labels and algebra where necessary, to explain the proofs of theorems to his high school students. Student feedback regarding those methods was always highly positive. Students regularly used colored pencils and markers when doing their homework and writing their exams.

Oliver Byrne’s Euclid can certainly be used to supplement the teaching of geometry at all levels, visually enhancing students’ understanding of the geometric arguments through color and the isolation of parts of the diagram. The reader might want to use Byrne’s proof of the sum of the angles of a triangle equaling “two right angles” (or 180 degrees or \(\pi\) radians; see Figure 19) – or any other of Byrne’s color proofs – with their students supplying the reasons for each step, or creating their own color proofs.

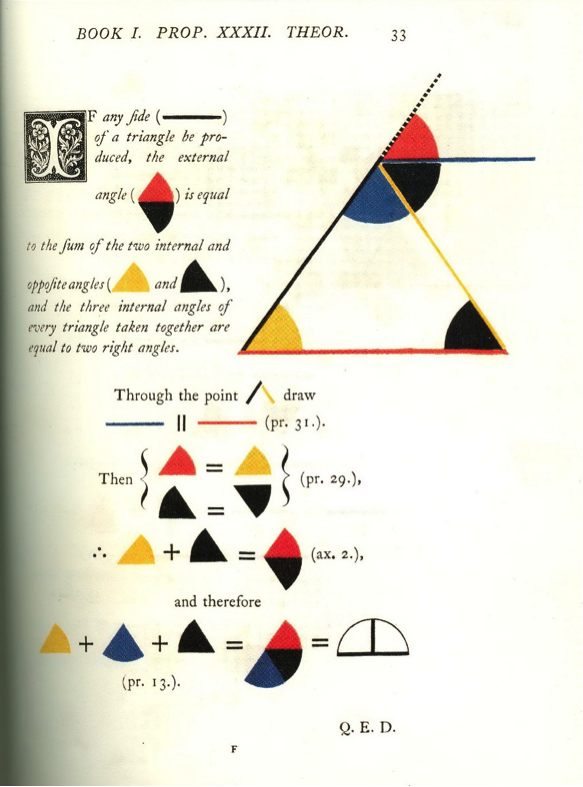
Figure 19. Byrne’s proof that the sum of the angles of a triangle is equal to two right angles (Book I, Proposition 32 of Euclid’s Elements). (Image from the book in the collection of Sid Kolpas)

Instructors of history of mathematics classes might use Byrne's Euclid as a catalyst to discuss the history of mathematics textbook design, the debate over the teaching of geometry in Victorian England, and the rise of non-Euclidean geometry. A good source for the debate over geometry education in Victorian England can be found in Euclid and His Modern Rivals by the English mathematician Charles Lutwidge Dodgson, better known as Lewis Carroll.¹¹⁵ The book debates the pedagogical merit of thirteen contemporary geometry textbooks, demonstrating how each is either inferior to or equivalent to the approach of Euclid's Elements. As Oliver Byrne supported the use of Euclid's Elements in the mathematics curriculum, Dodgson supported The Elements as the geometry textbook that should be used in schools instead of the more modern geometry textbooks that were beginning to replace it.

Debate over the importance of proof as taught in the high school Geometry class, the correct way to teach Geometry, and how to integrate geometry into the mathematics curriculum continues to this day. Also, in the nineteenth century, non-Euclidean geometry was challenging Euclidean geometry's place as the only true system of geometry. History of mathematics students could discuss the development of such geometries, and how they would make their own color proofs of theorems in Lobachevsky's or Riemann's non-Euclidean geometries. For example, we know that the sum of the angles of a spherical triangle is greater than 180 degrees. An example of a color-aided presentation of a spherical triangle, whose angles sum to 270 degrees, can be found at http://www.math2earth.oriw.eu/ via Math2Earth > Outcomes > Spherical trigonometry: sum of angles of a triangle on a sphere.

113 Sidney J. Kolpas, The Pythagorean Theorem: Eight Classic Proofs (Addison-Wesley Publishing / Dale Seymour Publications, Palo Alto, California, 1992). Kolpas dedicated this book to Oliver Byrne since Byrne's Euclid in Colours inspired him to write it! The book includes other color dissection proofs of the Pythagorean Theorem that could be used in Geometry classes, by historical figures as diverse as the Indian astronomer and mathematician Bhaskara, the Italian artist and scientist Leonardo da Vinci, and the future U.S. President James A. Garfield.

114 Peter Lynch, “That’s Maths: The rebel who brought Technicolour to Euclid,” Irish Times, February 20, 2014. On the preceding page of this article, Peter Lynch was introduced as a meteorologist and mathematics blogger.

115 Lewis Carroll (Charles Dodgson), Euclid and his Modern Rivals (New York: Dover Publications, 1973; originally published in 1879).