Cubes, Conic Sections, and Crockett Johnson - Classic Greek Constructions

What tools did Euclid use in these constructions?  Did he follow the tradition of the Greek philosophers, using only a compass and unmarked straightedge, or did he use a ruler with some type of markings such as centimeters, or a tool like a protractor?  It is clear from the postulates and propositions in Book I that actual lengths, which would require units of measurement, were not being used, hence there would be no rulers with markings nor would there be protractors.  Euclid’s Postulate 3, which hypothesizes the existence of compasses (“To describe a circle with any centre and distance” Euclid 1956, I:154), does not actually describe the instrument we now call a compass.  Rather, the compass Euclid postulated lost its setting when it was picked up and is referred to as a collapsing, or collapsible, compass.  If one considers the content of Propositions I.2 and I.3 (“To place at a given point (as an extremity) a straight line equal to a given straight line” and “Given two unequal straight lines, to cut off from the greater a straight line equal to the less” [Euclid 1956, I:244 and 246]), one can see that a collapsible compass can do all that a non-collapsing compass can.  Thus, we can assume Euclid’s compass was non-collapsing.  Heath remarked on this in his commentary on the Elements [Euclid 1956, I:246]: 

Proclus alludes … to the error of those who proposed to solve [Proposition] I.2 by describing a circle with a given point as center and with a distance equal to [the given straight line], which he says is a petitio principii [begging the question].  De Morgan puts the matter very clearly… We should “insist,” he says, “here upon the restrictions imposed by the first three postulates, which do not allow a circle to be drawn with a compass-carried distance; suppose the compasses to close of themselves the moment they cease to touch the paper.  These two propositions [I. 2, 3] extend the power of construction to what it would have been if all the usual power of the compasses had been assumed; they are mysterious to all who do not see that postulate iii does not ask for every use of the compasses.”

As we consider the role of constructions in the Elements, we will ask if, given a line segment of length \(1,\) is it possible to construct a segment of some other length using only a straightedge and compass?  For example, given a segment of length \(1,\) can we construct segments of length \[2,\,\,{\sqrt{2}},\,\,{\rm or}\,\,\,{\sqrt[{\scriptstyle 3}]{2}}\] using only those instruments?  Proposition I.2 allows us to construct a segment of length \(2\) by simply placing “at a given point [one endpoint of the given segment of length \(1\)] a straight line equal to the given straight line,” i.e., by just copying the segment of length \(1.\) To construct a segment of length \({\sqrt{2}},\) we simply construct an isosceles right triangle with legs of length \(1\) and then use the Pythagorean Theorem to show that the hypotenuse has length \({\sqrt{2}}.\)  In general, we will say that a number is constructible if a segment of that length can be constructed using only a straightedge and compass.

There are other relatively simple constructions, though not necessarily the ones employed by Euclid, that illustrate arithmetical calculations.  For example, given a segment of length \(1,\) it is certainly possible to construct an arbitrary positive integer by just repeated copying of segments.  Also, if \(a\) and \(b\) are constructible, then \(a+b\) and \(a-b\) \((a>b)\) are constructible: we get the sum by copying a segment of length \(b\) at the end of a segment of length \(a\) and get the difference by applying Proposition I.3.  The diagrams in Figure 1 (below) display methods for constructing \(ab\) and \(a/b\) for \(b\not=0.\)  In each of these diagrams we find the point D by constructing a line parallel to AB through C.  The calculations needed to show that \(x=ab\) and \(y=a/b,\) respectively, rely on the fact that the construction yields similar triangles so that corresponding sides are in proportion.  Thus the diagram on the left gives \[{\frac{a}{1}}={\frac{a+x}{1+b}}\] and the one on the right gives \[{\frac{b}{1}}={\frac{b+a}{1+y}}.\]

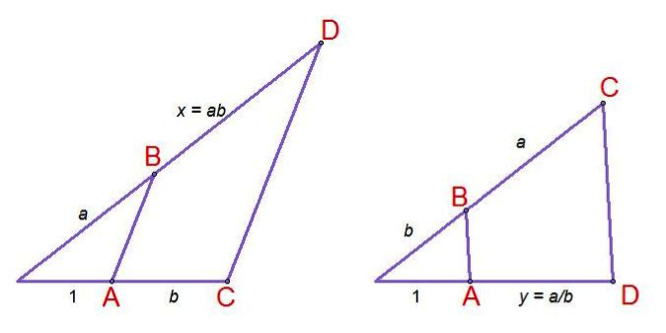
Figure 1. Construction of \(ab\) and \(a/b\) from \(a\) and \(b.\) (Constructed using Geometer’s Sketchpad.)

Although it is not quite as obvious, it is not difficult to show that if \(a\) is constructible, then \({\sqrt{a}}\) is also constructible.  This construction can be shown using a mean proportional, i.e., the solution to the equation \[{\frac{a}{x}}={\frac{x}{b}}\,\,\,{\rm or}\,\,\,x=\sqrt{ab}.\] It is common to see mean proportionals introduced using the diagram on the left in Figure 2 (below), where all three triangles are right triangles.  This diagram can be used to prove that the three right triangles are similar and, therefore, the vertical line is the length of the mean proportional between \(a\) and \(b.\)  We can form the diagram on the right by describing a circle with center the midpoint of a segment of length \(a+1.\)  Since angles inscribed in semicircles are right angles, the diagram shows the mean proportional between \(a\) and \(1,\) i.e., \({\sqrt{a}}.\)

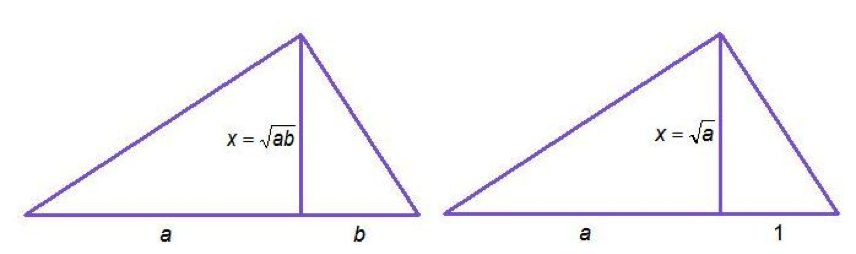
Figure 2. Construction of \(x=\sqrt{ab}\) and of \(x=\sqrt{a}.\) (Constructed using Geometer’s Sketchpad.) 

Since whole numbers are constructible, any number that can be built up from a whole number using a finite number of the “operations” of \(+,\) \(-,\) \(\cdot,\) \(\div,\) and \(\sqrt{\phantom{xx}},\) is constructible.  Thus, all (positive) rational numbers, and at least some positive irrational numbers, can be constructed.  However, since \({\sqrt[{\scriptstyle 3}]{2}}\) is not a rational number or a finite application of square roots, it cannot be constructed in the manner we have outlined, leaving unanswered the question whether it can be constructed using only a straightedge and compass.  In the seventeenth century, René Descartes, after whom the Cartesian coordinate system is named, showed that straightedge and compass constructions could be expressed algebraically in terms of the solution of iterated quadratic equations. Since François Viète had shown earlier that both trisection of an angle and duplication of a cube lead to cubic equations, Descartes concluded that neither of these problems was soluble with straightedge and compass. Although Descartes’ conclusion is correct, a rigorous proof depends on concepts that had not yet been formulated.  The final proof that \({\sqrt[{\scriptstyle 3}]{2}}\) is not constructible using a compass and unmarked straightedge is generally credited to Pierre Wantzel in a paper published in 1837.  Since it is necessary to construct a segment of length \({\sqrt[{\scriptstyle 3}]{2}}\) in order to duplicate a cube with edge of length \(1,\) this proof also showed that it is impossible to double an arbitrary cube with only a compass and an unmarked straightedge.  In fact, none of the three Greek problems can be solved with only a compass and an unmarked straightedge.

We may now ask if there are other techniques that make the duplication of a cube possible.  One answer involves conic sections but we will temporarily delay explaining how conic sections are involved and, instead, turn to the question of how an author of children’s books became interested in constructibility problems.