Cubes, Conic Sections, and Crockett Johnson - Problem of Delos (Menaechmus)

Figure 8.  Problem of Delos (Menaechmus), by Crockett Johnson (1968).  (Courtesy of Philip Nel.)

Answering the question: “What do the straightedge lines and compass arcs do when two parabolas and a hyperbola double a cube, just stand watching?”

As we can see in Figure 9, Crockett Johnson used the diagram in Figure 7 to produce a wonderful visualization of the two parabolas and a hyperbola doubling a cube in his painting The Problem of Delos (Menaechmus) (1968), shown in Figure 8.  If the side of the small square in the lower left-hand corner of this painting, outlined in blue in Figure 9, is considered to have length \(1,\) i.e. \(a=1,\) then the larger square, sharing its lower left hand corner, has sides of length \(\sqrt[{\scriptstyle 3}]{2},\) the necessary length to construct a cube of volume \(2,\) thus doubling a unit cube!  Note also that the rectangle that shares its base with the larger square has width \(\sqrt[{\scriptstyle 3}]{2}\) and height \({\sqrt[{\scriptstyle 3}]{2}}^2\) or \(2^{2/3}.\)  Thus, by superimposing Crockett Johnson's painting with the unlabeled graphs of Figure 7, one can understand why the answer to the question he posed is yes!

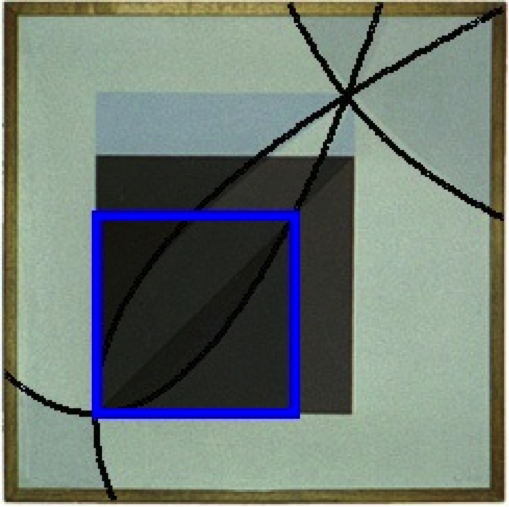
Figure 9.  Problem of Delos (Menaechmus), by Crockett Johnson (1968), with the unlabeled graphs from Figure 7 superimposed on the painting from Figure 8.  The square outlined in blue has side length \(1.\)