Pitfalls and Potential Solutions to Your Primary Source Problems: Conclusion – Objects in the review* mirror are more complicated than they appear.

There is rarely a geodesic from the genesis of an idea to its conclusion.

Example

I am currently struggling to solve this issue. I want to figure out a way to use history to help motivate Laplace Transforms. Teaching this concept has been a struggle for decades. In 1963, S. T. R. Hancock wrote of this challenge in a very contemporary way:

In technical colleges one is often called upon to introduce advanced mathematical techniques to students whose background is not very extensive. Such a method is the use of the Laplace Transform for the solution of linear differential equations with constant coefficients. Textbooks which deal with this topic, even those specifically written for engineers, derive the transform from the Fourier Integral, or from Heaviside’s Operational Calculus, or just brusquely define the process [Hancock 1963, p. 3].

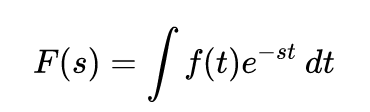
Figure 11. Statement of Laplace Transform. Wikipedia.

Laplace Transforms are obviously named after Pierre-Simon Laplace (1749–1827). But that is the only simple thing about their evolution; for instance:

• Laplace didn’t actually create or use the modern Laplace Transform.
• What Laplace did had previously been done by Euler.
• The modern Laplace Transform wasn’t used until 1910, by Harry Bateman (1882–1946).
• Lots and lots and lots involving the development of Laplace Transforms happened between Euler's time and Bateman's.
• Laplace transforms can be motivated in many different ways. They are a formalization of Oliver Heaviside’s (1850–1925) Operational Calculus; they can be viewed as a way of measuring how similar two functions are; they can be understood as a specific example of a functional transform; or they can be considered as a generalization of power series and generating functions.

For years, I’ve struggled to find the right way to use the history of this topic to help with the learning. And, I’ve not figured it out. Yet. But, what is good for the goose is good for the struggling mathematician. I’ve . . .

• Googled the topic repeatedly.
• Checked older textbooks such as [Ince 1944].
• Referenced modern textbooks with a historical bent, including Differential Equations with Applications and Historical Notes [Simmons 2017].
• Found dozens of secondary sources from journals, such as Archive for History of Exact Sciences, American Mathematical Monthly, Mathematical Gazette, CODEE Journal, and more.
• Read Laplace’s biography by Gillispie et al. [1997].
• Reached out to friends^[25] for help, including Danny Otero, Dick Pulskamp (and his extensive website [Pulskamp n.d.]), Ken Monks, and others.
• Begun to disseminate the work in other ways, such as talks at MAA conferences.
• And so on.

Each of these steps have clarified my path—a bit. But as I’ve not yet cracked the problem, I clearly don’t have all the answers. Nonetheless, hopefully you now know a few common pitfalls to avoid along with some possibly novel potential solutions to help you as you fight through them. And, knowing is half the battle. Good luck with the other half!