Algebra Tiles Explorations of al-Khwārizmī ’s Equation Types: Modeling al-Khwārizmī’s More Complicated Problems – Example 14

Example 14: A problem leading to the case “squares and numbers = roots” [Rosen 1831, 39–40]

I have divided ten into two parts; I have then multiplied each of them by itself, and when I had added the products together, the sum was fifty-eight dirhems.

Computation: Suppose one of the two parts to be thing, and the other ten minus thing. Multiply ten minus thing by itself; it is a hundred and a square minus twenty things.

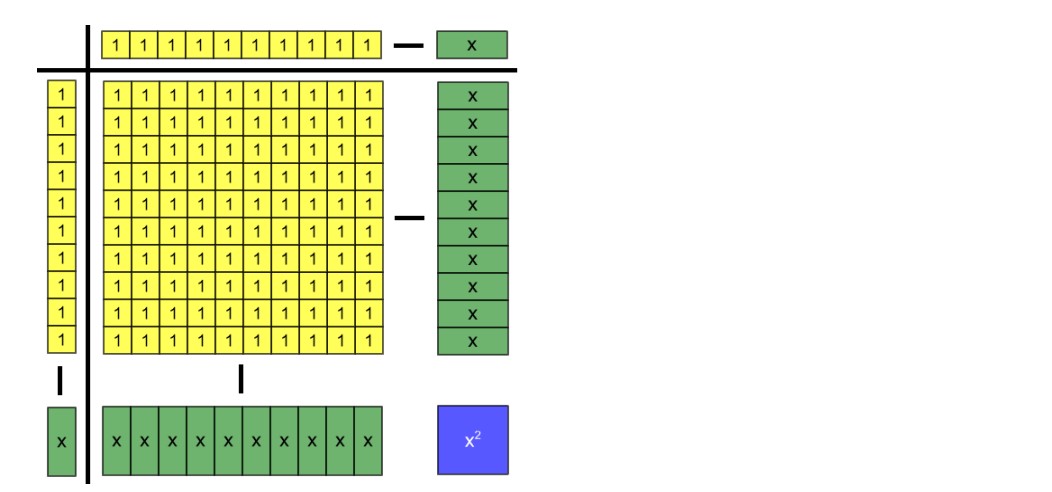
Then multiply thing by thing; it is a square.

The sum is a hundred, plus two squares minus twenty things, which are equal to fifty-eight dirhems.

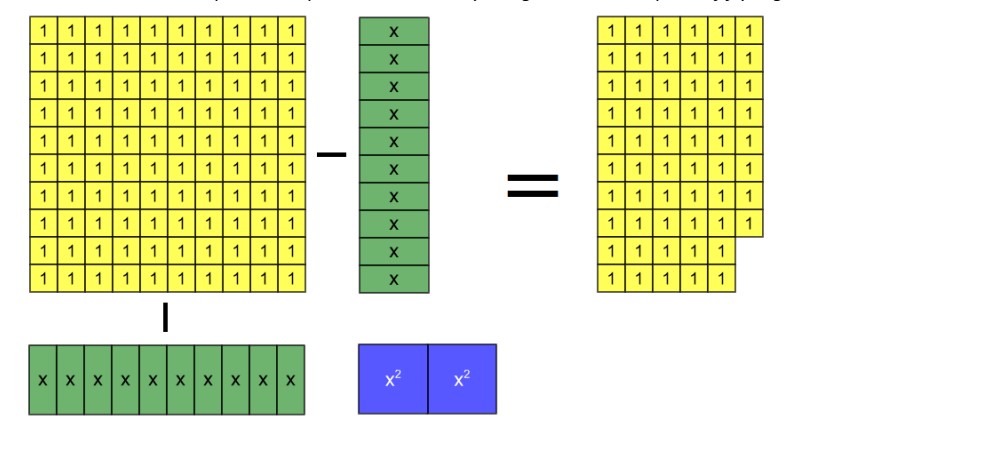
Take now the twenty negative things from the hundred and the two squares, and add them to fifty-eight; then a hundred, plus two squares, are equal to fifty-eight dirhems and twenty things.

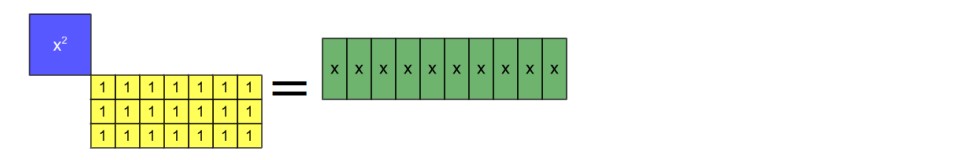
Reduce this to one square, by taking the moiety of all you have. It is then: fifty dirhems and a square, which are equal to twenty-nine dirhems and ten things.

Then reduce this, by taking twenty-nine from fifty; there remains twenty-one and a square, equal to ten things.

Halve the number of the roots, it is five; multiply this by itself, it is twenty-five; take from this the twenty-one which are connected with the square, the remainder is four. Extract the root, it is two. Subtract this from the moiety of the roots, namely, from five, there remains three.⁴ This is one of the portions; the other is seven. This question refers you to one of the six cases, namely “squares and numbers equal to roots.”

Continue to Example 15.
Back to Overview of al-Khwārizmī’s More Complicated Problems.

Notes

4. Note that “twenty-one and a square, equal to ten things” is a Type 5 equation that can be modeled using the procedure from the section "Squares and Numbers are Equal to Roots"; therefore, the algebra tile representations of these final steps have been omitted.