- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Algebra Tiles Explorations of al-Khwārizmī's Equation Types: Modeling the Compound Equations and the Moiety Principle
This section focuses on modeling the three compound species in al-Khwārizmī’s algebra text using a step-by-step problem-solving approach, with each case treated in a separate subsection:
- Type 4: roots and squares = numbers
- Type 5: squares and numbers = roots
- Type 6: squares = roots and numbers
As al-Khwārizmī noted, the three basic equations (Types 1-2-3) discussed in the previous section “do not require that the roots be halved” [Rosen 1831, 13]. In the latter three (Types 4-5-6), on the other hand, he asserted that “halving the roots is necessary” [Rosen 1831, 13]. The utilization of moities is thus also a key feature in modeling the solutions of equations of Types 4-5-6 using algebra tiles representations. He also provided geometric constructions (based on specific equation examples) for each of these three equation types “to point out the reasons for halving” [Rosen 1831, 13]. For equations of Types 5 and 6, the diagrams that accompany these synthetic constructions are not transferrable to an algebra tile representation. As indicated by Figure 2, however, the second diagram that al-Khwārizmī gave in his demonstration for Type 4 equations (based on the example x2+10x=39) is reminiscent of the algebra tile models we provide in this section. Notice in particular the small square of area 25 in the lower left-hand corner, which represents the final step in the "Completing the Square" technique for solving this equation. In fact, similar diagrams are widely used with an equation of Type 4 to illustrate that solution technique.
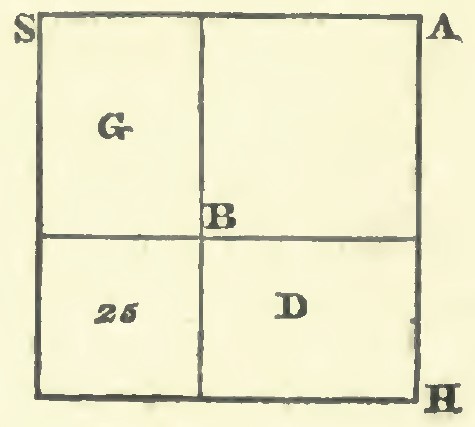
Figure 2. Second diagram in al-Khwarizmi's geometric demonstration of Type 4
using the equation “a Square and twenty-one Dirhems are equal to ten Roots” [Rosen 1831, 16].
Günhan Caglayan (New Jersey City University) , "Algebra Tiles Explorations of al-Khwārizmī's Equation Types: Modeling the Compound Equations and the Moiety Principle ," Convergence (October 2021)





