Van Schooten's Ruler Constructions - Verbatim First Solution to Problem I

We repeat Problem I, trisecting a given angle.

Problem I.  Given a rectilinear angle BAC, to cut it into two equal pieces.

In the detailed version of the solution to Problem I given on the preceding page, we slightly misrepresented the given data.  In fact, when van Schooten gives us the angle BAC, he seems to be assuming that AB is a segment, and that C is a segment with A as one of its endpoints.  It could also be that B and C are both line segments, sharing A as an endpoint, and that the symbol B is also used to denote a point on the segment B.  This seems to have no real consequence, but it is curious.  Van Schooten’s words translate as follows.

Suppose that in the line AB points B and D are placed. and are placed on line AC so that AE equals AD, and E is a point further along AC so that EF equals DB; And draw straight lines BE, DF, which intersect at G.  I say that the line AG cuts the angle BAC into two equal pieces.

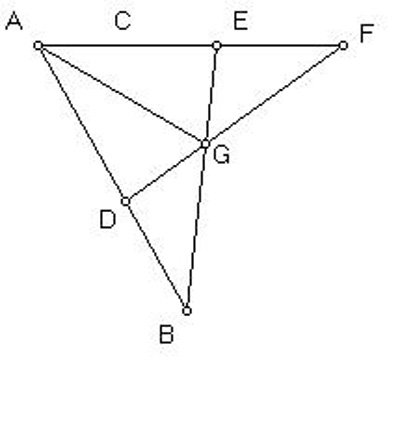
Van Schooten also includes a proof of the correctness of each of his constructions, but we will generally leave those to the reader.

Next:

Second solution to Problem I

Problem II