Triangles in the Sky: Trigonometry and Early Theories of Planetary Motion - Calculating Planetary Positions

Now that we have determined the parameters of the basic ancient model for each planet, we can calculate planetary positions. This involves solving the same triangle CEP that we solved to find the parameter r/R.

First we must initialize the model. Figure 8 shows two hypothetical positions of the planet: one at the initial time T₀ and another at a future time T.

Figure 8

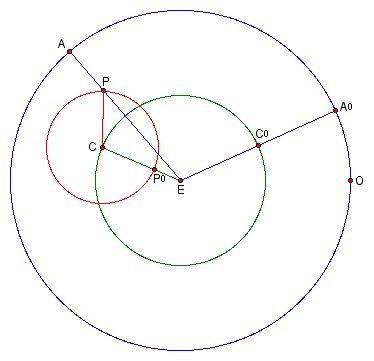
At time T₀ the planet is in the middle of its retrograde arc and occupies the position P₀ on the epicycle. The epicycle center is at the point C₀ on the deferent, and the apparent planet is aligned with the center at the point A₀ on the ecliptic. At this point one uses an astronomical instrument to observe the longitude of the planet. This gives the position in degrees of the apparent planet on the ecliptic, as measured from the point O on the ecliptic, and initializes the model. (Recall from Section 3 that O is the apparent position of the sun on the ecliptic at the spring equinox.)

The planet's longitude at time T is calculated by finding the measure of arc A₀A and adding it to the planet's longitude at T₀. To do this we must solve triangle ECP for angle PEC. A sample calculation for Mars is shown below. For simplicity in the calculation we take as our unit of measure the radius R of the deferent; with this unit of measure, r is equal to the parameter r/R. The solution presented makes use of modern trigonometry and notation.

Example: The motion of Mars over the course of a retrograde arc is observed with an astronomical instrument, and it is determined that the middle of the arc occurred at longitude 40º. Find the longitude of Mars 250 days later.

Solution:

By Table 1 in Section 6. we know that the Sidereal rate is 0.524º/day and the Synodic rate is 0.462º/day. R = 1 unit (assumed); r = r/R = 0.66 by Table 3 in Section 8.

The longitude at T₀ is given as 40º and the elapsed time from T₀ to T is given as 250 days.

At T, arc C₀C = (sidereal rate) x (elapsed time) = (0.524) x (250) = 131º and

arc P₀P = (synodic rate) x (elapsed time) = (0.462) x (250) = 115.5º.

Angle ECP = arc P₀P = 115.5º.

EC = R = 1 unit; CP = r = 0.66.

By the Law of Cosines, PE = Ö(EC² + CP² - 2EC(cos(ECP))) = 1.416

By the Law of Sines, sin(PEC) = CP sin(ECP) / PE= 0.421, so that angle PEC = arcsin(0.421) = 25º.

Arc A₀A = arc C₀C - angle = 131º - 25º = 106º.

Longitude at T = Longitude at T₀ + arc A₀A = 40º + 106º = 146º.

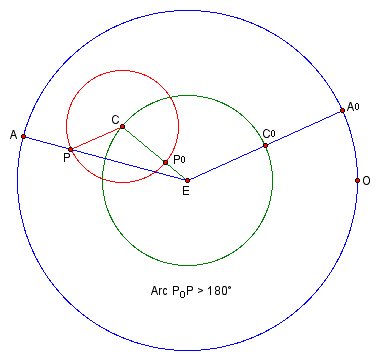
Note that all arcs are measured in a counterclockwise direction, and, by adding or subtracting multiples of 360º if necessary, have measure between 0º and 360º. Figure 9 shows a configuration in which arc P₀P is greater than 180º; this may be helpful to some readers in completing the following exercises.

Figure 9

Exercises

1. The motion of Jupiter over the course of a retrograde arc is observed with an astronomical instrument, and it is determined that the middle of the arc occurred at longitude 345º. Find the longitude of Jupiter 430 days later.

2. The motion of Mars over the course of a retrograde arc is observed with an astronomical instrument, and it is determined that the middle of the arc occurred at longitude 130º.

a. Find the longitude of Mars 500 days later.

b. Find the longitude of Mars 500 days before the observation.

3. The motion of Saturn over the course of a retrograde arc is observed with an astronomical instrument, and it is determined that the middle of the arc occurred at longitude 210º. Find the longitude of Saturn 1000 days later.

Answers

1. 15º

2. a. 52º b. 208º

3. 248º