Teaching and Learning the Trigonometric Functions through Their Origins

In this series, we present a collection of curricular units based on primary historical sources and designed to serve students as an introduction to the study of trigonometry. Each unit may be incorporated, either individually or in various combinations, into a standard course in College Algebra with Trigonometry, a stand-alone Trigonometry course, or a Precalculus course. These lessons have also been used in courses on the history of mathematics and as part of a capstone experience for pre-service secondary mathematics teachers.

Trigonometry is concerned with measurements of angles about a central point (or of arcs of circles centered at that point) and quantities, geometrical and otherwise, that depend on the sizes of such angles (or the lengths of the corresponding arcs). It is one of those subjects that has become a standard part of the toolbox of every scientist and applied mathematician. Why is it so valuable?

There is a key geometrical feature of the measurement of angles, or arcs that are traced out, about a point in the plane: as we might expect, the sizes of such angles (or the lengths of such arcs) grow as one end of the arc moves counterclockwise around its circle (while the other remains fixed); but when the moving point returns to the place of the fixed point after making a full turn around the circle, it continues to retrace the same path through another turn, and another and another as the angle/arc grows ever-larger. In other words, periodic behavior is at the core of the relationships between angles and arcs and the measurements we associate with them.

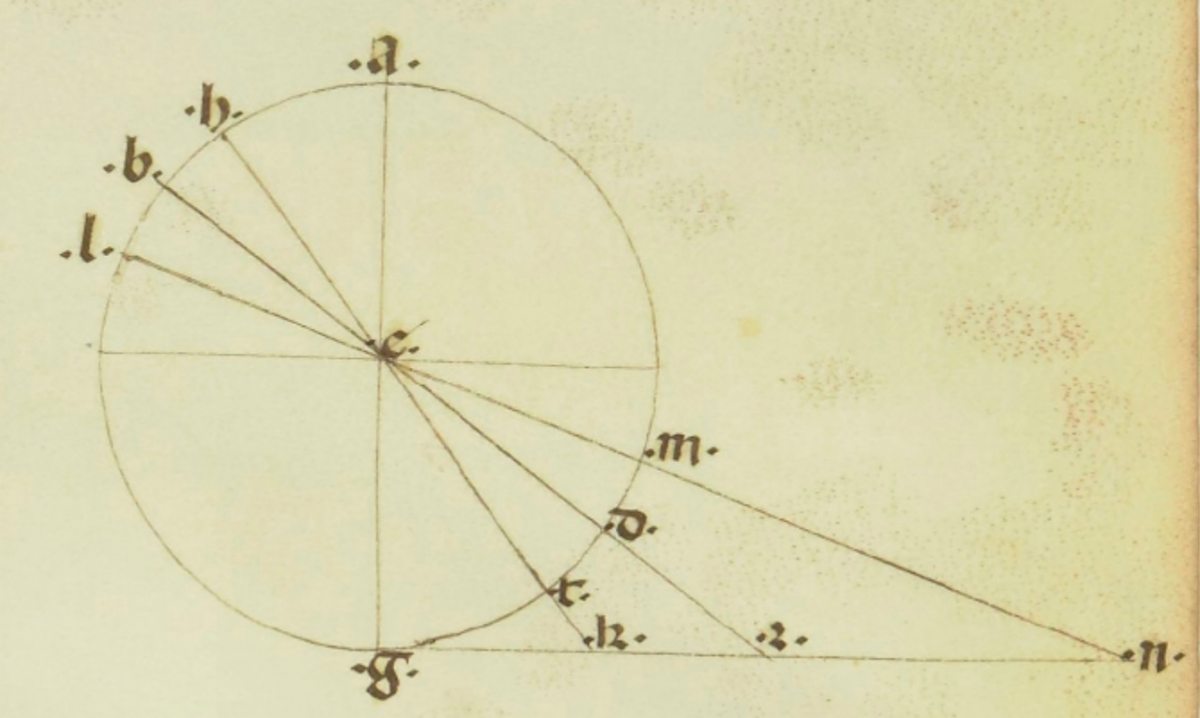
Diagram from 14th-century manuscript copy of Ptolemy's Almagest, folio 22 recto.
Manuscript owned and digitized by gallica.bnf.fr / Bibliothèque nationale de France.

As a consequence, wherever the mathematical description of cyclical phenomena is needed, trigonometric functions make appearances in pure mathematics and in applications of mathematics to the sciences. An introduction to trigonometry is a staple of the mathematics curriculum in high schools and colleges, many of whose students later study calculus and other forms of mathematical analysis in which periodic phenomena are explored.

It is the goal of the curricular units presented in this series to impart to students some of the story of where and how the central ideas of this subject first emerged, in an attempt to provide context for their study of this mathematical theory. Students who work through the entire collection of units will encounter six milestones in the history of the development of trigonometry. Our journey will span a vast interval of time, from some unidentifiable moment dating as far back as around 1000 BCE, to roughly the year 1500 CE. We will consider developments of the subject that took place in many different parts of the world: in ancient Mesopotamia; in Hellenistic Greece and Roman-era Egypt; in medieval India; in central Asia during the height of Islamic science; and in Renaissance Europe. The term “milestones'' is quite appropriate here, since we are only touching on a few moments in a long and complex history, one that brings us just to the edge of the modern scientific era in which we now live.

More specifically, each unit looks at one of the following episodes in the development of the mathematical science of trigonometry:

• the emergence of sexagesimal numeration in ancient Babylonian culture, developed in the service of a nascent science of astronomy;
• a modern reconstruction (as laid out in [Van Brummelen 2009]) of a lost table of chords known to have been compiled by the Greek mathematician-astronomer Hipparchus of Rhodes (second century, BCE);
• a brief selection from Claudius Ptolemy's Almagest (second century, CE) [Toomer 1998] in which the author (Ptolemy) shows how a table of chords can be used to monitor the motion of the Sun in the daytime sky for the purpose of telling the time of day;
• a few lines of Vedic verse by the Hindu scholar Varāhamihira (sixth century, CE) [Neugebauer and Pingree 1970/1972] containing the "recipe" for a table of sines as well as some of the methods used for its construction;
• passages from The Exhaustive Treatise on Shadows [Kennedy 1976], written in Arabic in the year 1021 by Abū Rayḥān Muḥammad ibn Aḥmad al-Bīrūnī, which include precursors to the modern trigonometric tangent, cotangent, secant and cosecant;
• excerpts from Regiomontanus' On Triangles (1464) [Hughes 1967], the first systematic work on trigonometry published in the West.

Woodcut showing Regiomontanus with an astrolabe, from the Nuremberg Chronicle by Hartmann Schedel (1493), folio 255 recto. Wikimedia image from copy owned by University of São Paulo, believed to be in the public domain.

Readers who want to learn more about the history of trigonometry are recommended to consult Glen van Brummelen's masterful The Mathematics of the Heavens and the Earth: The early history of trigonometry [Van Brummelen 2009] from which much of this work took inspiration.

The onscreen introduction for each individual unit to appear in this series will provide additional historical detail about the episode in question, a brief instructor guide specific to the goals and implementation of that particular unit, and a downloadable pdf of the unit itself that can be shared with students (after removing the Notes to Instructors section). Readers who want to see the entire Primary Source Project (PSP) from which these units are drawn can obtain that PSP, A Genetic Context for Understanding the Trigonometric Functions, without waiting for future installments to appear, from the website of the NSF-funded project TRansforming Instruction in Undergraduate Mathematics via Primary Historical Sources (TRIUMPHS). LaTeX source code of the entire PSP and of each individual unit is also available directly from the author by request. The concluding chapter of this series will describe the various paths that instructors have followed in their implementation of these materials in the range of courses described in the opening paragraph of this introduction, and it will provide comments from instructors and students about their experiences on those journeys.