Proportionality in Similar Triangles: A Cross-Cultural Comparison - The Ancient Greek Contribution

Similarity has its roots in antiquity, and ideas of proportion were likely known to the Pythagoreans in the fifth century [4, p. 82-83], while Eudoxus of Cnidus (ca. 408-355 ) established a notion of what today would be called the equality of ratios [3, p. 28], crucial in the case of incommensurable magnitudes. Euclid (third century ) devotes Book V of The Elements to a study of proportion, incorporating many of the ideas developed earlier in ancient Greece, particularly those practiced by Pythagoras and Eudoxus. Proposition Four in Book VI of The Elements [1] states:

VI.4. In equiangular triangles the sides about the equal angles are proportional, and those are corresponding sides which subtend the equal angles.

In a model of deductive reasoning, Euclid proves this statement, which relies on propositions VI.2, VI.1, Book V, and ultimately on Book I for constructions in Euclidean geometry. To provide the reader with a sense of development for VI.4, and a glimpse into the ideas behind the result, here are the propositions VI.2 and VI.1, in reverse order as presented in The Elements [1].

VI.2. If a straight line be drawn parallel to one of the sides of a triangle, it will cut the sides of the triangle proportionally; and [conversely].

VI.1. Triangles and parallelograms which are under the same height are to one another as their bases.

Height here refers to perpendicular height, and by the ratio of two triangles "to one another" is meant the ratio of their areas. In modern parlance, VI.1 states that the ratio of the area of two triangles with the same height is equal to the ratio of their bases, and this result also holds for parallelograms. For triangles with commensurable bases, Euclid's proof of VI.1 begins by constructing whole number multiples of the two bases to arrive at two triangles with equal bases. The result then follows from I.38, discussed in the introduction. Issues of incommensurability are addressed via the Eudoxan theory of proportion.

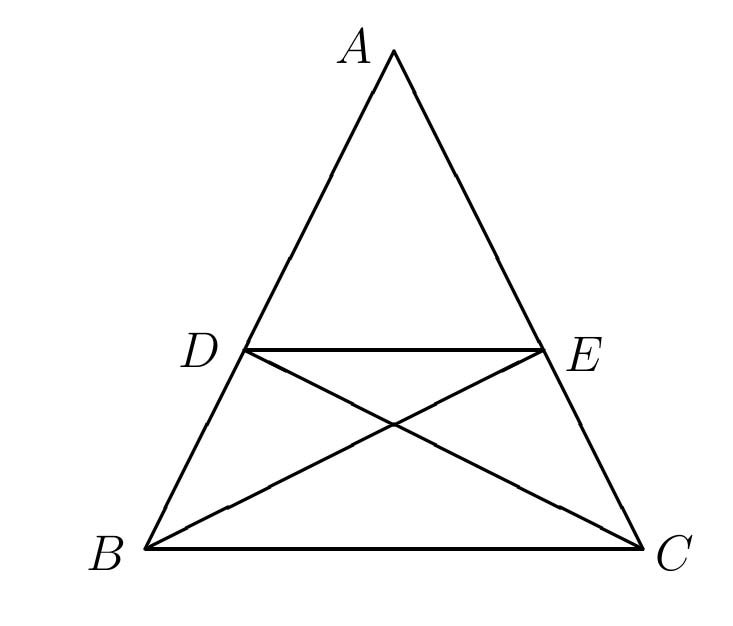
Figure 1: Proposition VI.2.

To prove VI.2, Euclid begins with triangle ABC (not necessarily isosceles) and constructs DE parallel to BC (Figure 1). Note that triangles DEB and DEC have the same area, since they are on the same base DE and are in the same parallels (between DE and BC). It follows that triangle ABE and triangle ACD have the same area. A modern interpretation of Euclid would read

Since triangles ABE and DEB are under the same height, they are to one another as their bases, and similarly for triangle ACD and triangle DEC. Thus,

It follows that AB/DB = AC/EC.

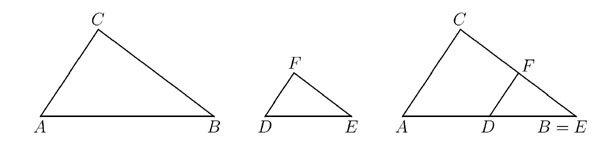
Figure 2: Proposition VI.4.

To prove VI.4 quickly, begin with similar triangles ABC and DEF and construct triangle DEF inside triangle ABC along a congruent pair of angles (Figure 2). The result then follows from Proposition VI.2, and a general position argument stating that triangle DEF could be constructed inside triangle ABC along any pair of congruent angles. Euclid, however, avoids a general argument in favor of a more literal proof. (See [1]).