Online Museum Collections in the Mathematics Classroom – Spherometers and Basic Trigonometry

Classroom Application #7: Spherometers and Basic Trigonometry

A spherometer is a device for measuring the curvature (positive or negative) of a surface, usually a convex lens made by an optometrist or lenses and mirrors used in telescopes.

Clark Spherometer, 19th century, Smithsonian Institution negative number DOR2014-05922.

The three legs of the device sit on the object to be measured, such as a lens. A micrometer (very precise screw) is lowered in the middle until it just touches the surface. The distance of the crown of the curve from the plane of the three legs is read off the micrometer on the spherometer. This measurement is the sagitta, denoted by \(h\) in the diagram below. Deriving the radius of curvature, \(R,\) from \(h\) involves a considerable amount of simple right triangle trigonometry that students can figure out on their own. For instance, the side length of the equilateral triangle formed by the three legs can be denoted by \(a\). Then some nice right triangle geometry shows that the distance from one leg to the center of the spherometer is \({\frac{a}{\sqrt{3}}}.\) From this, the formula for the radius of curvature, \(R,\) can easily be found: \[R={\frac{a^2}{6h}}+{\frac{h}{2}}.\] The figures below show \(R,\) \({\frac{a}{\sqrt{3}}},\) and \(h\), in top and side views of the surface being measured.

Top view diagram of the distance between the legs and central screw of a spherometer measuring a radius of curvature.

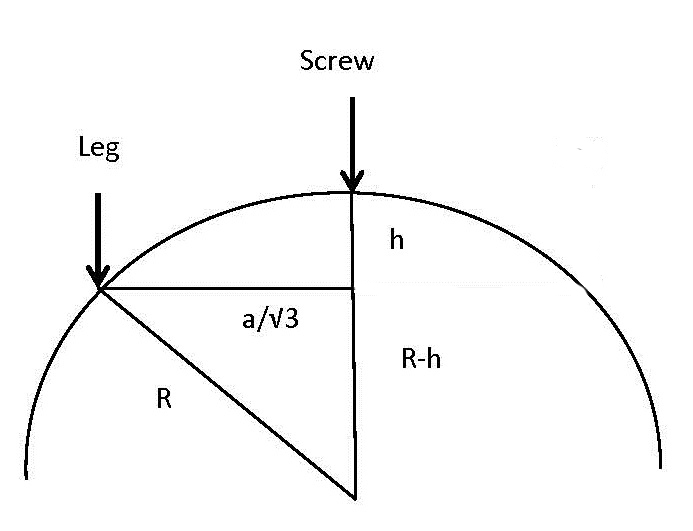
Side view diagram of a spherometer measuring the radius of curvature, R, of a curved surface.

Since spherometers are not commonly found, a simple one can be made by students or the instructor in fairly short order. Here is one Amy Shell-Gellasch made for her students to demonstrate how the device works.

Cardboard and screw spherometer constructed by Amy Shell-Gellasch.