Mathematical Treasure: Keuffel & Esser Trammel Ellipsograph

Ellipsographs are, as the name implies, devices for drawing ellipses. Ellipsographs were used by surveyors, engineers, draftsmen, architects and machinists to produce technical drawings. Many elements of these drawings require rendering a circle in perspective, which results in an ellipse. For example, architectural elements such as windows and arches are elliptical.

There are several methods for drawing ellipses by hand, the most common being the two pins and a loop of string approach, which works surprisingly well. There are also several methods for drawing them mechanically. German artist Albrecht Dürer, known for his precise perspective drawings, invented a compass to draw ellipses in 1540.

Most ellipsographs are Trammels of Archimedes, in which two sliders move perpendicularly to each other, constrained by a connecting bar. By adjusting where the sliders are attached to the bar, ellipses of various eccentricities can be generated. A pencil attached to the end of the bar traces the ellipse. This model was manufactured (or imported) in approximately 1930 by the Keuffel & Esser Company of New York and is marked “Keuffel & Esser Co N.Y. Switzerland.”  It is a precision device used by draftsmen and engineers. It can draw ellipses of major axis 6 to 18 inches in length.

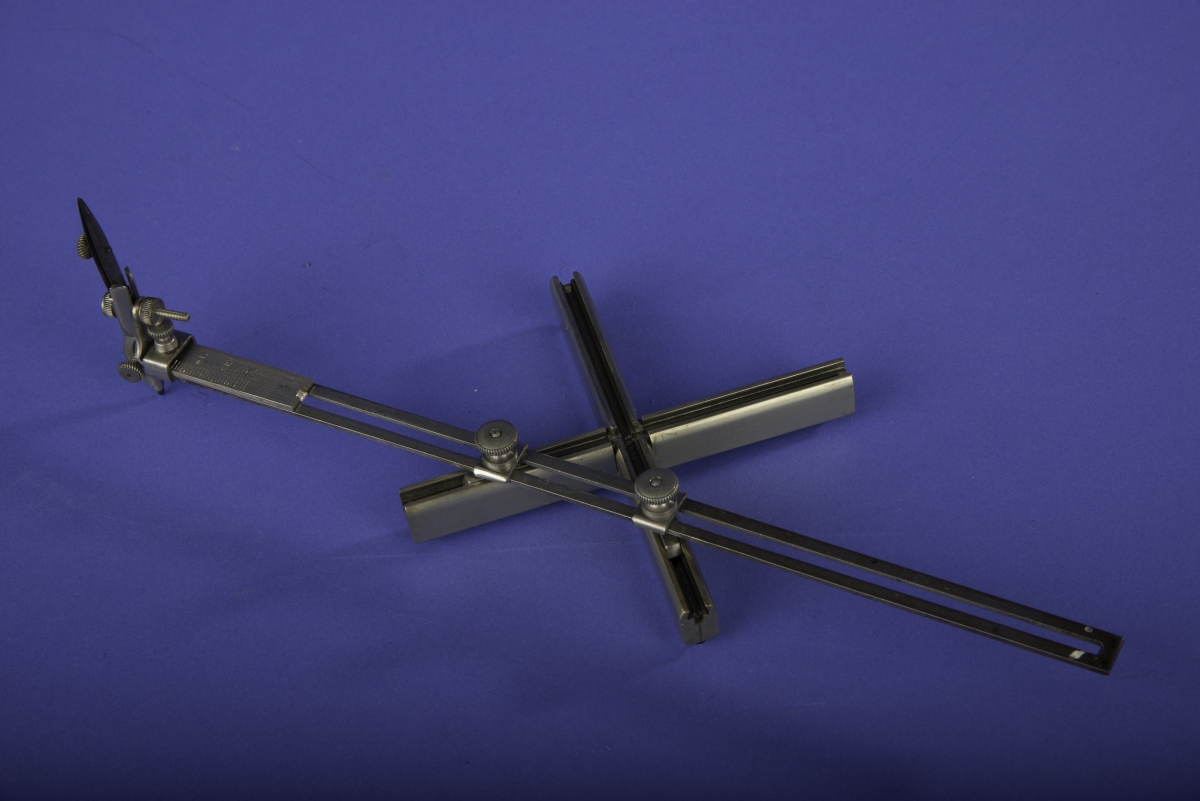
Keuffel & Esser Trammel Ellipsograph, ca 1930, Smithsonian Institution negative number DOR2013-00718.

This device was a gift from Brown University in 1973. The Smithsonian also owns ellipsographs of much more complicated design. All of them were built between the mid-19th and early 20th centuries. All eight ellipsographs from the collection of the Smithsonian Institution’s National Museum of American History are shown at the website https://www.si.edu/spotlight/ellipsographs.

Elsewhere in Convergence, four ellipse drawers, including string and trammel drawers, are illustrated dynamically in "Curve Drawing Then and Now." The remaining ellipse drawers from "Curve Drawing Then and Now" are due to Proclus and Frans van Schooten. Van Schooten's ellipse drawer is illustrated on page 317 of his 1657 Five Books of Mathematical Exercises. Another illustration of Van Schooten's ellipse drawer from this book can be seen in the Convergence article, "Van Schooten's Ruler Constructions."

Index of Mathematical Treasures

Index of Mathematical Objects