Combining Strands of Many Colors: Episodes from Medieval Islam for the Mathematics Classroom - Tassels of Colored Silk

A Moorish problem dealing with colored threads of silk makes a great starting point for learning about certain combinatorial relationships. I present the module, "Combinations and Their Sums," to students in Statistics and Finite Math courses after we’ve already covered the basic facts about factorials, permutations and combinations in the standard course syllabus.

The silk problem was posed by Ahmad ibn Ibrāhīm ibn ‘Alī ibn Mun‘im al-‘Abdarī (d. 1228), a physician and mathematician born in Spain who lived much of his life in Marrakech. During this period, what is now Spain and Morocco were united under the Almohad dynasty. This was a major silk-producing region, and ibn Mun‘im began by listing 10 colors that were available for dyed silk thread. He then asked: in how many different ways can threads in 3 of the 10 colors be combined to form a silk tassel? Breaking the possibilities down into mutually exclusive cases, ibn Mun‘im reasoned that one could select either the 3rd color in the list together with both of the colors above it; or the 4th color in the list together with any 2 of the 3 colors above it; or the 5th color together with any 2 of the 4 colors above it; and so on, up to the 10th color together with any 2 of the 9 colors above it. Written in the modern notation of binomial coefficients (“combinations”), his conclusion was as follows:

\[\binom{10}{3}=\binom{2}{2}+\binom{3}{2}+\binom{4}{2}+\binom{5}{2}+\binom{6}{2}+\binom{7}{2}+\binom{8}{2}+\binom{9}{2}.\]

In this way, ibn Mun‘im was able to express the number of combinations of colors taken 3 at a time as a sum of combinations of colors taken 2 at a time, for which he had already derived the formula:

\[\binom{n}{2}=\frac{n(n-1)}{2}.\]

My students have also previously learned this formula, and I ask them to complete the tassel calculation in the same way as did ibn Mun‘im:

\[\binom{10}{3}=1+3+6+10+15+21+28+36=120\,\,{\rm{different}}\,\,{\rm{tassels.}}\]

The students go on to learn that ibn Mun‘im generalized this pattern so that he could expand any given binomial coefficient as a sum of coefficients of lower order. To facilitate the use of this rule, he organized the results on successive rows of an arithmetical triangle that I present (see Figure 1). The calculation above is summarized on the third-lowest row of numerals in ibn Mun‘im’s triangle. Note the similarity between this triangle and “Pascal’s Triangle” that appeared centuries later in France (Lamrabet 1994: 215-216, 300-2; Katz 2009: 292-294).

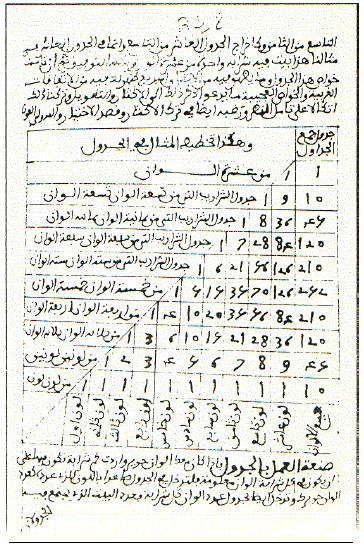
Figure 1a. Ibn Mun‘im’s arithmetical triangle. (Image from Actes du Huitième Colloque Maghrébin sur l’Histoire des Mathématiques Arabes, Tunis, les 18-19-20 Décembre 2004, Tunis: Tunisian Association of Mathematical Sciences, 2006.)
 

Figure 1b. An adaptation of ibn Mun‘im’s arithmetical triangle for classroom use.
 

The tassel problem exemplifies the first of the five points of my strategy because it captures the interest of students, and its concreteness aids them in understanding the concepts involved. Going through this problem allows them to grasp the resulting pattern more durably than if it were derived—or simply stated—as an abstract formula. To test and solidify students' grasp of the pattern, I follow up with a series of exercises along these lines:

Exercise.  Use the same logic as ibn Mun‘im to complete these expansions:

a)   \(\binom{10}{6}=\binom{5}{5}+{\phantom{xxxxxx}}+{\phantom{xxxxxx}}+{\phantom{xxxxxx}}+{\phantom{xxxxxx}}.\)

b)   \(\binom{10}{7}={\phantom{xxxxxx}}+{\phantom{xxxxxx}}+{\phantom{xxxxxx}}+{\phantom{xxxxxx}}.\)

Interpret your answer in words, in terms of making a tassel from silk threads of various colors.

c)   \(\binom{8}{3}=\)

Interpret your answer in words, in terms of selecting whom, from the workers in one office, will be chosen to attend a professional development seminar.

d)     Use numbers and words of your own choosing to make up a problem
        like that in part (c), and provide an answer for it.

The type of verbal interpretation that I am looking for in part (b) is: “To make a tassel from 7 of the 10 colors, choose either color 7 and all 6 of the 6 colors above it, or color 8 and any 6 of the 7 colors above it, or color 9 and any 6 of the 8 colors above it, or color 10 and any 6 of the 9 colors above it.”

This exercise illustrates three more of the five points of my strategy. First, notice that I use modern binomial notation even though it did not exist in ibn Mun‘im’s time. Such “translations” help streamline the work (but take care, in making such translations, not to inadvertently misrepresent the way in which the knowledge was conceived in the original context). Second, this exercise leads students to state their results in both a numerical and a verbal form. Other exercises in the same module make use of graphical and algebraic representations, in the form of the arithmetical triangle and formulae with symbols such as \(\binom{n}{r}.\) The use of such multiple perspectives leads to a sturdier understanding by the student, appeals to different learning styles, and is also intrinsically interesting. Third, even within this single exercise, the tasks required of the student progress gradually from easier to more difficult. At first the scaffolding of blank spaces is pre-filled in part, then not pre-filled at all, and finally the scaffolding is withdrawn altogether. In addition, what is requested is only a numerical answer at first, then a verbal interpretation in terms of the by-then-familiar 10 colors of dyed silk, then in terms of a different situation altogether, and finally in terms of a situation dreamt up by the student.

Download:

• the module that introduces students to counting colored tassels as ibn Mun‘im did, Combinations and Their Sums (Elementary Statistics, Finite Mathematics), and
• the follow-up module, Binomial Coefficients and Subsets (Finite Mathematics).