- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Approximate Construction of Regular Polygons: Two Renaissance Artists - Albrecht Durer
Albrecht Dürer (1471-1528), considered the father of modern German painting, was also a great mathematical amateur. He wrote a book titled Unterweysung der Messung..., which deals with all sorts of geometrical problems. In this book he gives an exact construction of the regular pentagon, but he also gives an approximate construction which is quick to execute in practical drawing. (His book was aimed at artisans, stonemasons, etc., who cared more for simple procedures than for geometrical accuracy.)
This construction is shown below:
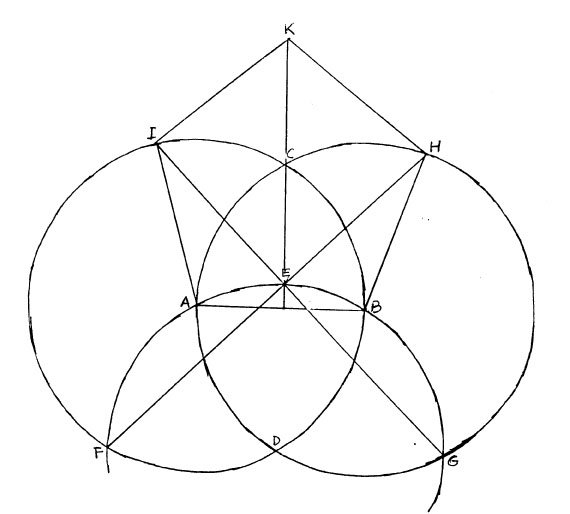
Take a fixed opening of the compass: AB = a. Draw circles of radius a, centered at A and B; let these circles intersect at C and D. Then AB = AD = BD, as we know. Draw the circle of center D and radius DA. This circle will pass through B; let E be the point where it intersects CD, and let F and G be the points where it intersects the circles centered at A and B, respectively. Produce FE until it intersects at H the circle centered at B; produce GE until it intersects at I the circle centered at A. Then the intersection K of the circles of radius a, centered at H and I, gives the fifth vertex of the pentagon.
How regular is this pentagon? If it were exactly regular, all sides should subtend an angle of 360°/5 = 72° at the center of the pentagon. Therefore, all angles at the vertices of the pentagon should be equal to 180°-72°= 108°(since each of them is the sum of two equal angles of [180°-72°]/2). In fact, we will see that angle ABH= 108°21’58” --- a little more than 108°, so that some other angle must be a little less than 108°. First note that angle FBG = 90°; then, since FG = 2a and GB = a, we have FB = a√3 . Also, angle DFE = 45°and angle DFC = angle DFA = 60° (F, A, C are collinear, as well as C, B, G and F, D, G ) , and therefore angle AFE = 15°. Since angle BFE subtends an arc of circle FABG equal to that subtended by angle AFE, we also have angle BFE= 15°.
We now use the law of sines for triangle FBH; this gives sin(angleBHF) = √3 sin15° (observe that since angle BHF < 90°, there is no ambiguity). From here angle BHF can be found and then angle HBF; then, subtracting angle ABF = 30° from angle HBF, we find that angle ABH is approximately 108°22’, a value close to that of Pedoe [2].
Since angle BAI is equal to angle ABH, it too is a little greater than 108°. Also, each of angles BHK and AIK is a little greater than 107°, while angle HKI is a little greater than 109°... and still, this would be barely noticeable in our drawing.
Dürer does not warn the reader of his book that this construction is approximate. (It is, also, executed with a “rusty” compass, i.e., a compass with a fixed opening.) In fact, he also quotes, as we said, the exact construction of the pentagon.
“Dürer’s interest in the construction of regular polygons is explained by the applications of geometry during the Middle Ages in Islamic and Gothic decorative design and, after the invention of guns, in the building of fortified towns. (It is curious that very few buildings in history have been built based on the pentagonal shape. The Pentagon, near Washington, DC, is a notable exception.)” [2]
Summarizing this survey of Renaissance polygon constructions, we may say that both Leonardo da Vinci and Albrecht Dürer were great mathematical amateurs. “Leonardo wrote a lot about polygons; but it was Dürer, more than Leonardo, who transmitted to us the popular medieval constructions.” [2]
Raul A. Simon, "Approximate Construction of Regular Polygons: Two Renaissance Artists - Albrecht Durer," Convergence (August 2010)




