- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
An Investigation of Historical Geometric Constructions - Construction of the Quadratrix I
Hippias’ quadratrix was described as follows: “ABCD is a square and BED is part of a circle, centre A radius AB. As the radius AB rotates about A to move to the position AD then the line BC moves at the same rate parallel to itself [i.e., B′C′] to end at AD. Then the locus of the point of intersection F of the rotating radius AB [i.e., AE] and the moving line BC is the quadratrix” (from Book IV of Pappus' Synagoge circa 340) (see Figure 1).
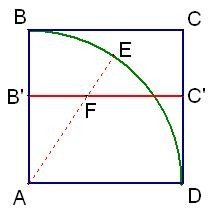
Figure 1: Quadratrix of Hippias
To construct the quadratrix using Geometer’s Sketchpad, you will first need to construct a square, ABCD, in your sketch window. Select any point on CD and call it C'. Construct a parallel line to BC, through the point C'. Name the point of intersection of AB and the parallel line point B'. Construct the segment B'C' and hide the line B'C' (see Figure 2).
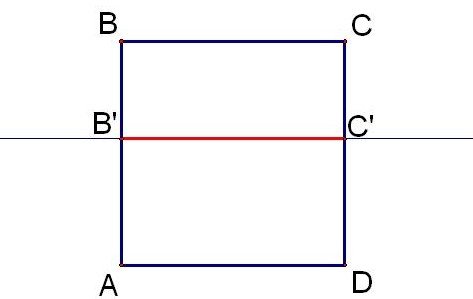
Figure 2: Construction of the Quadratrix
Construct the circle with center A and radius AB. Next, construct arc BXD by identifying a point on the circle inside the square (point X). Then select, in order, points B, X, D and choose Arc Through 3 Points under the Construct menu (see Figure 3).
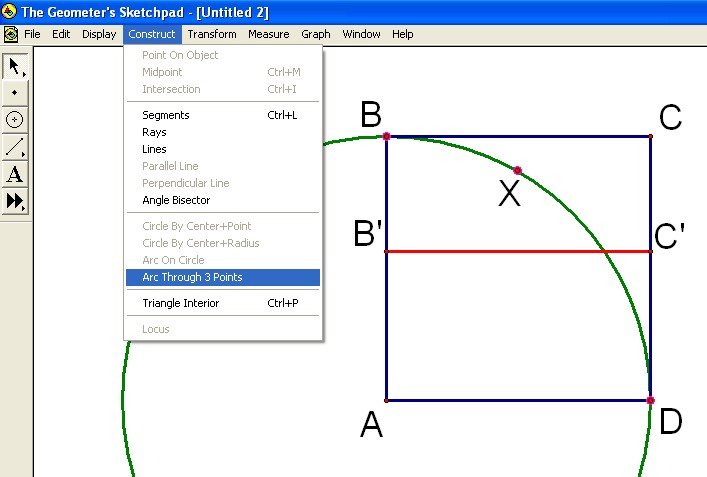
Figure 3: Continuing the Construction of the Quadratrix by Constructing Arc BXD.
Suzanne Harper and Shannon Driskell, "An Investigation of Historical Geometric Constructions - Construction of the Quadratrix I," Convergence (August 2010)





