Aiding the Teaching of Geometry and Affording Mathematical Recreation: Paper Folding in the Spirit of Sundara Rao of Madras – Reforms to Geometry Teaching in Secondary Schools

Also in the 19th century, mathematics educators were questioning then-standard approaches to teaching geometry as the subject moved from colleges into secondary schools while enrollment in secondary education expanded. From at least the time of the introduction of the printing press, university students in Britain and continental Europe had routinely studied geometry by memorizing translations and condensations of Euclid’s Elements. By 1850, many European countries had abandoned Euclid for use in introductory geometry, preferring alternative organizations of propositions and more practical treatments. As Joan Richards [1988] and Michael Price [1994] have shown, British mathematicians and mathematics teachers disagreed sharply about what texts were most appropriate for students.

The London firm of Macmillan published two textbooks that well illustrate this debate. The first was by Isaac Todhunter (1820–1884) of St. John’s College, Cambridge University, who had graduated from there as Senior Wrangler in 1848. In the preface to his The Elements of Euclid for the Use of Schools and Colleges, which first appeared in 1862, Todhunter wrote:

In England the text-book of Geometry consists of the Elements of Euclid; for nearly every official progamme of instruction of examination explicitly includes some portion of this work. Numerous attempts have been made to find an appropriate substitute for the Elements of Euclid; but such attempts, fortunately, have hitherto been made in vain. The advantages attending a common standard of reference in such an important subject, can hardly be overestimated; and it is extremely improbable, if Euclid were once abandoned, that any agreement would exist as to the author who should replace him. It cannot be denied that defects and difficulties occur in the Elements of Euclid, and that these become more obvious as we examine the work more closely; but probably during such examination the conviction will grow deeper that these defects and difficulties are due in a great measure to the nature of the subject itself, and to the place which it occupies in a course of study [Todhunter 1862, pp. vii–viii].

Todhunter went on to present Euclid’s definitions, postulates, propositions, theorems, and corollaries.

 
Figure 4. Isaac Todhunter as an undergraduate at Cambridge, as drawn by T. C. Wageman,
ca 1848, and James Maurice Wilson as photographed by Marcus Guttenberg in the 1870s.
MacTutor and National Portrait Gallery, available via a CC BY-NC-ND 3.0 license.

Another graduate of St. John’s College of Cambridge University, James Maurice Wilson (1836–1931)—who was Senior Wrangler in 1859 and then taught mathematics at Rugby School—took a different view of Euclid. In the preface to his Elementary Geometry, first published in 1868, Wilson wrote:

Euclid’s recognised and acknowledged faults as a system of Geometry, and as a specimen of analysed reasoning, are of slight importance compared with others of greater magnitude. The real objections to Euclid as a text-book are his artificiality, the invariably syllogistic form of his reasoning, the length of his demonstrations, and his unsuggestiveness [Wilson 1868, vol. 1, p. v].

Wilson’s book, while including many of the results of Euclid’s Elements, did not follow the order of the classic text.

In 1871, a few British secondary school teachers who were critical of the use of Euclid as a geometry text banded together with two university faculty members to form the Association for the Reform of Geometry Teaching, an organization promptly renamed the Association for the Improvement of Geometry Teaching (AIGT). They strove to develop a standard curriculum for geometry teaching that could be incorporated into national and university matriculation examinations, but the organization accomplished its goal of replacing Euclid as the standard introduction in geometry for students in Britain and British colonies only slowly [Price 1994]. It did welcome and publicize alternative approaches to the discipline, including Sundara Rao’s Geometrical Exercises in Paper Folding. The goals of the AIGT broadened over the years, and it officially changed its name to the Mathematical Association in 1897. Rao joined the society in 1904 [Anonymous 1911, p. 32].

Figure 5. Thomas Archer Hirst (1830–1892) was the first president of AIGT. MacTutor.

At the same time some sought to replace Euclid as a textbook, others, both in Britain and in the United States, sought further reforms. These instructors hoped to provide budding scientists and engineers with a thorough knowledge of the practical mathematics needed in their work. Handling protractors and drawing instruments, studying mathematical models, using slide rules and mathematical tables, and exploring functions using graph paper were all deemed valuable activities. Some reformers stressed the advantages of combining the study of geometry and algebra, the better to understand functional relationships. Many of these reforms were associated with the name of the British educator John Perry (1850–1920), whose influence on British mathematics teaching has been discussed by Brock and Price [1980]. David L. Roberts [2012] has considered the reception of his ideas in the US.^[4] In Massachusetts, Englishman Walter Smith developed a curriculum for teaching industrial design that included a set of wooden models. Other drawing courses, such as that of Louis Prang, encouraged use of models made from paper and even supplied templates for them [Kidwell 1996]. William T. Campbell [1899] would develop this approach in detail at the end of the century, while William W. Speer [1888] of Chicago extended the idea of folded paper models to children.^[5]

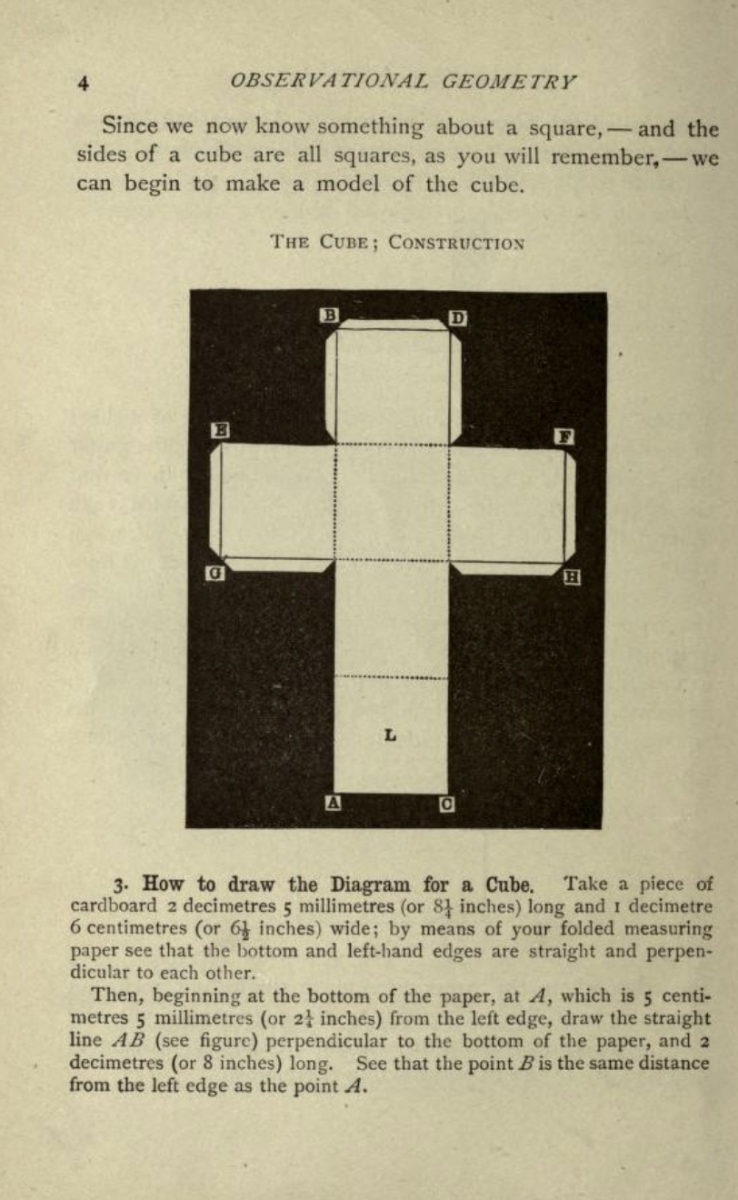
Figure 6. Campbell’s 1899 Observational Geometry showed how to
draw, cut out, fold, and paste geometrical solids such as the cube. Internet Archive.