Pantas’ Cabinet of Mathematical Wonders: Images and the History of Mathematics – To Know How to See

Let me give two examples of instances when the use of historical images resulted in fruitful discussions and prompted further learning. During the 2004 History and Pedagogy of Mathematics Conference in Uppsala, Sweden, Leo Rogers gave a talk, “Robert Recorde, John Dee, Thomas Digges, and the ‘Mathematicall Artes’ in Renaissance England” [Rogers 2004], in which he employed several illustrations. One simple image, from Robert Recorde’s Pathway to Knowledge [1551] excited the audience: “Where did you get that? How can I get a copy?” (See Figure 2.)

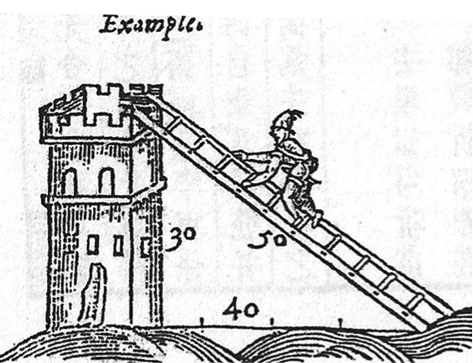
Figure 2. Image from Robert Recorde’s 1551 Pathway to Knowledge,
illustrating an application of the Pythagorean Theorem.

The situation depicted is elementary: it shows a man ascending a ladder to reach the top of a tower. The geometry reveals a right triangle—actually a 3-4-5 right triangle—and the viewer is asked to determine the length of the ladder when the height of the tower is given as 30 feet and the foot of the ladder is 40 feet away from the tower. What is the pedagogical / psychological appeal here?

• The ladder against a wall problem is familiar to almost all young algebra and geometry students.
• The illustration is from the 16th century: “They were doing the same problems as us almost five hundred years ago!”
• The Pythagorean theorem is being demonstrated: Given the lengths of the two legs of the triangle, the viewer can find the length of the hypotenuse. (Or, given the lengths of the hypotenuse and one leg, the viewer can find the length of the remaining leg.)
• A 3-4-5 right triangle is involved.
• The illustration appeals to the imagination of a young viewer: A castle is being stormed.

At a talk I gave in the U.S. at a National Council of Teachers of Mathematics (NCTM) Conference (Indianapolis, 1994), I briefly discussed and illustrated the galley method of division by using an image from a 1604 manuscript presented to King James I of England as a gift [Waymouth 1604]. (See Figure 3.)

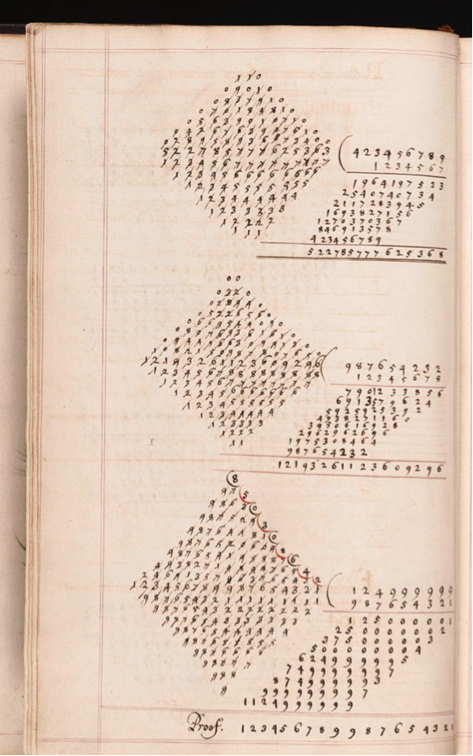
Figure 3. "Galley division" demonstrated in the handwritten and illustrated manuscript, The Jewell of Artes, prepared by George Waymouth, who presented it as a gift to King James I of England in 1604. (Image provided courtesy of the Beinecke Rare Book and Manuscript Library, Yale University. You may use this image in your classroom; all other uses require permission from the Beinecke Library.)

After this talk, the student aides, all secondary school students, solicited me to show them this diagram again and to explain it in detail, elaborating on just how the operation of division was being carried out. First, they were visually attracted to the number configuration: a large rhombus (or diamond) of numbers. They soon became conceptually involved: if this is a division exercise, “How was the process undertaken?” I explained the algorithm, line by line, pointing out the significance of the crossed-out or “scratched-out” terms, and noted that, due to its appearance, the technique was sometimes referred to as “scratch division.” In this discussion, I also interjected the fact that at this time in history, there were several division algorithms in use and this one, more popularly known as “galley division,” was the most frequently performed. I left my audience with the provocative comment that I believe this algorithm to be more mathematically efficient than the one we have actually adapted for school teaching, the “downward” or “Italian division” method. I hoped some of the students would investigate and attempt to substantiate my claim.

In selecting images for instructional purposes, two requirements should be fulfilled:

1. The material should appeal to the viewer; that is, be visually or conceptually attractive or intriguing. It should seduce the viewer “to want to find out more.”
2. It should have purpose and power by pertaining to the mathematical situation, topic, or concept you wish to promote and clarify.

Figure 4. Basket from northern Thailand.

For example, view the object in Figure 4. Can you see any mathematics? Ask a companion to perform the same task and compare what each of you see. Hopefully, each of you has perceived some similar images: a circle, trapezoids, hexagons, etc. (Do you see the star?) You would probably eventually query, “What is this object? Where did it come from?” If so, I have set you up to pursue the topic of ethnomathematics. The object in question is a container for sticky rice as served during a meal in northern Thailand. The tribal people who made the basket never had formal schooling or studied geometry, and yet they exhibited knowledge of the geometric shapes you have discovered and were certainly concerned with the concept of volume—in this case, the volume of a circular cylinder of rice.