Cubes, Conic Sections, and Crockett Johnson - Conic Sections and Doubling a Cube

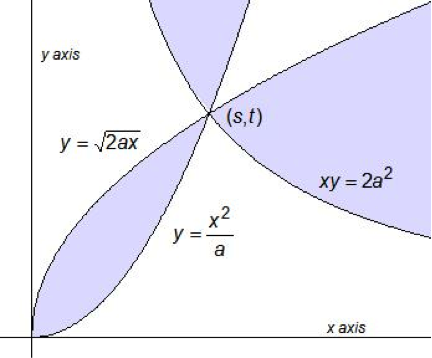
Figure 7. Two parabolas meeting a hyperbola at the point \((s,t).\) (Constructed using Geometer’s Sketchpad.)

Given a cube with edge of length \(a,\) how do we now utilize conic sections to double that cube; i.e., how do we find \(x\) so that \(x^3=2a^3\)?  Consider the point of intersection, \((s,t),\) in Figure 7 above.  The curves in this figure represent the parabolas \(x^2=ay\) and \(y^2=2ax,\) together with the hyperbola \(xy=2a^2;\) i.e., the graphs of the functions \[y={\frac{x^2}{a}}, {y=\sqrt{2ax}},\,\,{\rm{and}}\,\,{y={\frac{2a^2}{x}}},\] for \(x>0.\)  The equation of the first function tells us that \(t={{s^2}/{a}},\) so that the equation of the second parabola becomes \[{\left({\frac{s^2}{a}}\right)}^2={2as},\,\,{\rm or}\,\,\,s^3=2a^3.\] Thus the \(x\)-coordinate of the point of intersection, \(s,\) is the length of the edge of a cube which is double the volume of the original cube with edge of length \(a.\)  If we look at the cube of the \(y\)-coordinate of the point we find \[t^3={\frac{s^6}{a^3}}={\frac{(s^3)^2}{a^3}}={\frac{4a^6}{ a^3}}=4a^3\] so the \(y\)-coordinate is the length of the edge of a cube which is quadruple the volume of the original cube, or the larger of the mean proportionals mentioned previously.