A Selection of Problems from A.A. Markov’s Calculus of Probabilities: Andrei Andreevich Markov

Andrei Andreevich Markov was born on June 14, 1856, in Ryazan Gubernia (governorate, similar to a state in the US) in Russia, the son of Andrei Grigorevich Markov, a successful lawyer. He graduated from the gymnasium (high school) in 1874 and enrolled in Petersburg University, where he received a gold medal for his work, On Integration of Differential Equations with the Help of Continued Fractions.

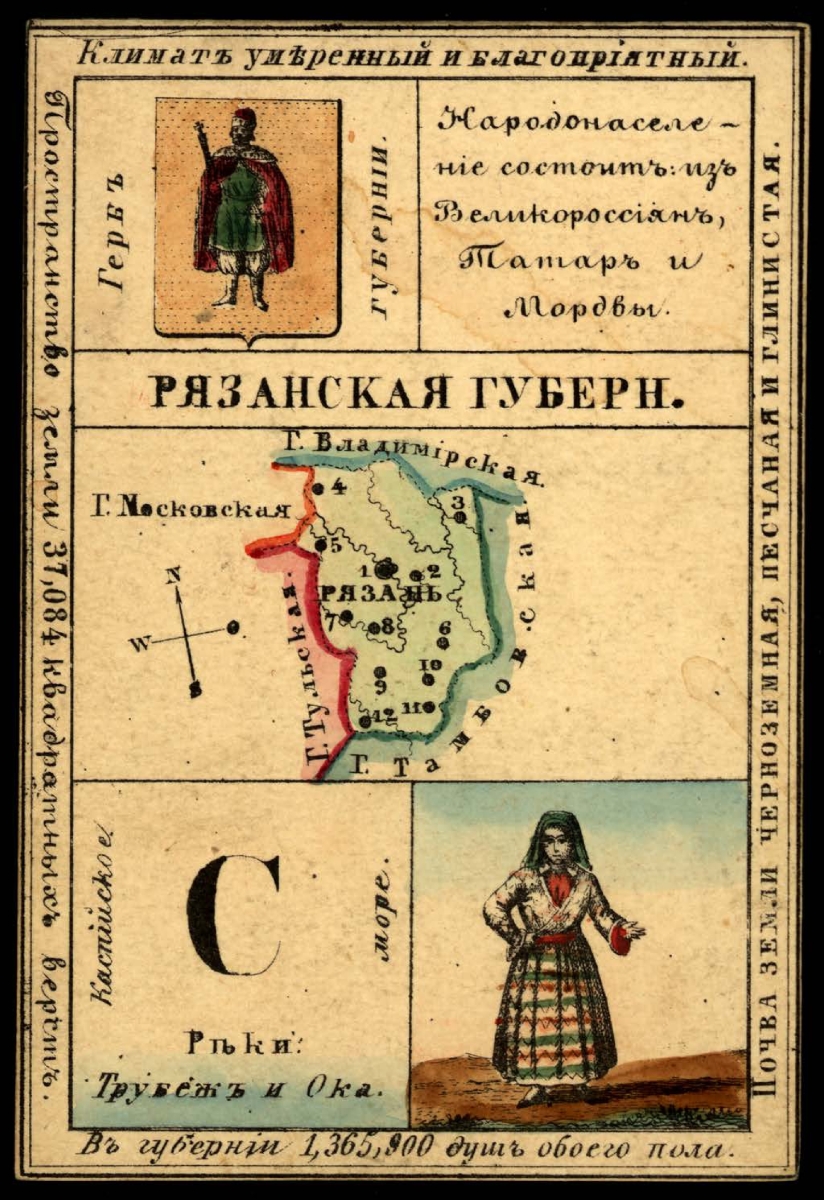
Figure 2. A card from a geographical card set with a map of Ryazan Gubernia in 1856
and the governorate located within the Russian Empire as it existed in 1914.
Wikimedia Commons, public domain and created by Milenioscuro, CC BY-SA 4.0 DEED.

He defended his master’s degree dissertation, On Binary Quadratic Forms with Positive Determinant, in 1880, under the supervision of Aleksandr Korkin (1837–1908) and Yegor Zolotarev (1847–1878). He then wrote his doctoral dissertation, On Some Applications of Algebraic Continued Fractions, under the supervision of Pafnuty Lvovich Chebyshev (1821–1894), in 1884. Markov taught at Petersburg University until 1905, but he continued to conduct research there until his death from sepsis after an operation on his leg on July 20, 1922.

Markov married Maria Ivanova Valvatyeva in 1883. They had one son, also named Andrei Andreevich Markov (1903–1979), who became a mathematician, noted for his work in constructive mathematics and logic. In 1951, the younger Andrei wrote a book, Selected Works (Избранные Труды), containing some of his father’s contributions to mathematics.

At some point in the 1890s, Markov became interested in probability, especially limiting theorems of probabilities, laws of large numbers and least squares (which is related to his work on quadratic forms). One point of emphasis was on generalizations of the Central Limit Theorem, where he eventually weakened the independence requirement, replacing it with dependence of each variable only on its immediate predecessor. This ultimately led to his work on what would be known later as Markov chains in 1906.² Specifically, a Markov chain is a discrete-state stochastic process \(\{X_1,X_2,X_3,\ldots,\}\) in which the probability mass function of \(X_n\) depends only on the value of \(X_{n-1}\) and not on the values of any previous variables; that is, \(P(X_n=j|X_{n-1}=i, X_{n-2}, X_{n-3},\ldots)=P(X_n=j|X_{n-1}=i)=p_{ij}\). The first use of the term “Markov chain” was most likely by Sergei N. Bernstein (1880–1968) in [Bernstein 1927].