Helping Ada Lovelace with her Homework: Classroom Exercises from a Victorian Calculus Course – Solution to Problem 8

In the 19th century, the word ‘impossible’ was widely used in the way that ‘imaginary’ is employed by mathematicians today, with real numbers often referred to as ‘possible’. Thus, in claiming in connection to Problem 8 that the expression
\[\int \frac{dx}{x^2-c} = \frac{1}{2\sqrt{c}} \log⁡\left(\frac{\sqrt{c}-x}{\sqrt{c}+x}\right )\] ‘becomes impossible when \(x\) is greater than \(\sqrt{c}\),’ De Morgan merely meant that when the integrand is positive, the logarithm on the right will become a logarithm of a negative number, and hence imaginary, or more accurately, complex. Lovelace’s first mistake was therefore to have misinterpreted the term ‘impossible quantity’ to mean something meaningless or illogical. As she said:

I fancy I had a little misunderstood the mathematical meaning of the words impossible quantity. I have loosely interpreted it as being equivalent to ‘an absurdity’ [LB 170, 21 Aug. [1841], f. 122r].

Her second mistake was to have assumed in the standard definition of a logarithm with base \(a\), that \(a\) could be positive or negative. Thus, if a logarithm happened to be odd, she inferred that its base could be negative, a circumstance which, she speculated, ‘would I think be admitted theoretically (tho' inconvenient practically) on the common beginner's introduction on the Theory of Logarithms. Am I right?’ [LB 170, 21 Aug. [1841], f. 122r]. A cursory glance at page 224 of De Morgan’s Elements of Algebra—which, as the letters attest, she had certainly read—reveals that the answer to this question is no!

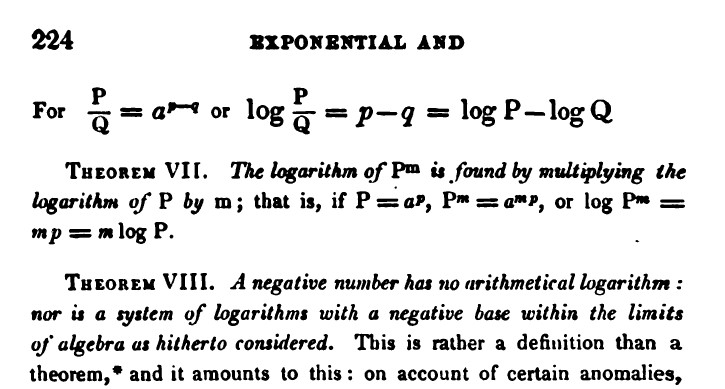
Figure 14. Theorem VIII on page 224 of De Morgan’s Elements of Algebra
states that logarithms cannot have a negative base.

Return to Main Solutions Page.
Continue to Solution to Problem 9.