Mathematical Treasure: Oronce Fine's Arithmetica practica

The works of Oronce Fine (1494–1555), a late-Renaissance mathematician and cartographer from France who taught in the Collège Royal in Paris (1531–1555), are well-represented in Convergence's Mathematical Treasures:

• Protomathesis (1532), which consisted of four books published together as well as separately:
  • De arithmetica practica
  • De geometria
  • Cosmographia
  • De solaribus horologiis
• a map of the world (1534)
• De mundi sphaera (1542)
• Le sphere du monde (1549 manuscript, 1551 publication)
• De re et praxi geometrica (1556)
• Opere Di Orontio Fineo (1670, original 1587)

The volume shown on this page is one of the separate publications of De arithmetica practica, from 1544. The text covered operations on integers, fractions, astronomical fractions, and proportions.

Title page and binding of Arithmetica practica, from the collection of Dr. Sid Kolpas.

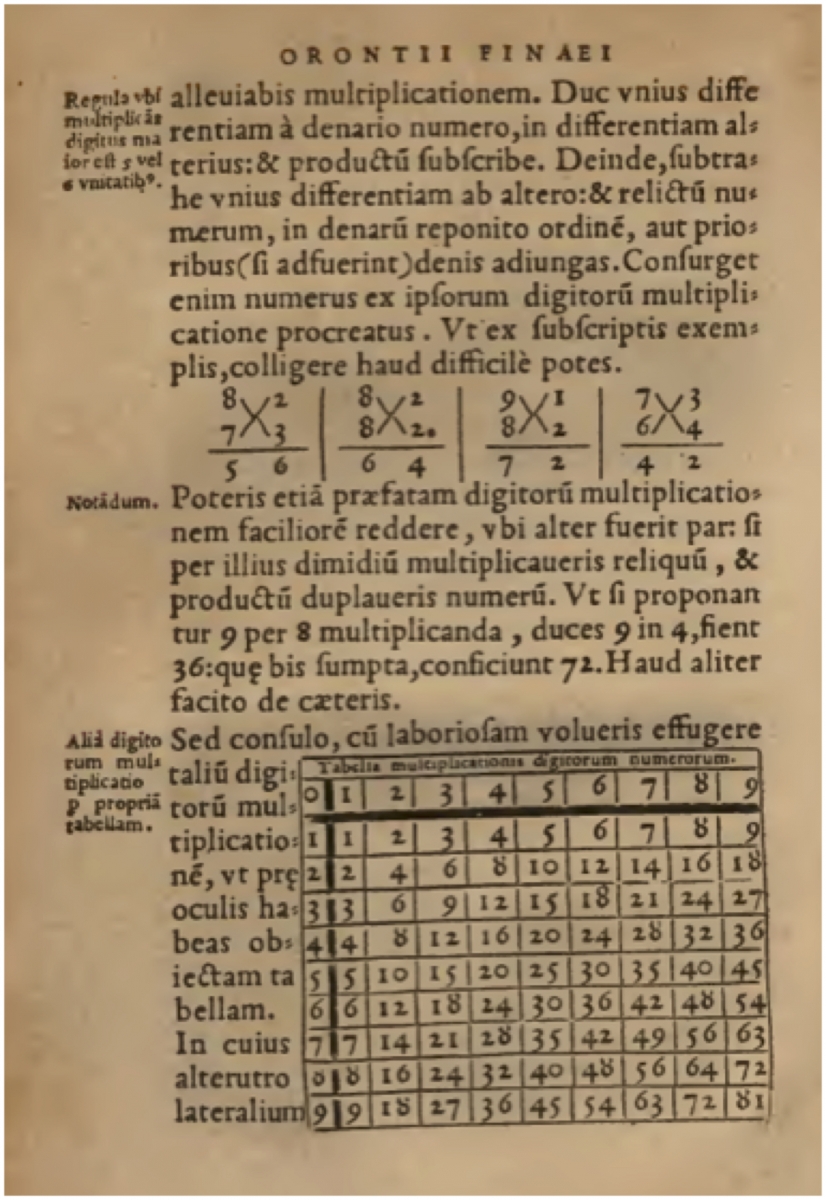
The 12th folio of the 1544 printing of Arithmetica practica, from the collection of Dr. Sid Kolpas.

The page on the left has a method for multiplying two single digit numbers that I have not seen before:

• Use the larger of the 2 single digits as the multiplicand and the smaller of the 2 single digits below it as the multiplier.
• Write the tens complement of each digit next to it.
• Multiply the complements and write down the product in the ones place of the answer, remembering any carried digit.
• Take the difference of the top digit and the bottom tens complement, add any previous carry, and write the result in the tens place of the product.

The reader might want to prove why this algorithm works.

The page on the right above has an early printed example of gelosia or lattice multiplication. Gelosia multiplication first appeared in print in Luca Pacioli’s Summa de arithmetica (Venice, 1494).

Luca Pacioli's Summa de arithmetica, geometria, proportioni et proportionalita. Images from Columbia University's copy of the 1494 first edition (in Mathematical Treasures) and GoogleBooks' scan of the 1523 printing.

References

"Lattice Multiplication." Wikipedia. https://en.wikipedia.org/wiki/Lattice_multiplication.

O'Connor, J. J., and E. F. Robertson. "Oronce Fine." MacTutor. September 2005. http://mathshistory.st-andrews.ac.uk/Biographies/Fine.html.

"Oronce Finé." Wikipedia. https://en.wikipedia.org/wiki/Oronce_Fin%C3%A9. The article on French Wikipedia adds substantial detail on Fine's biography and publications.

Index to Mathematical Treasures