Mathematical Treasure: Petri’s Reckoning and Cypher Book

Nicolaus Petri (fl. 1567–1603) was a Dutch mathematician and astronomer. His Practicque om te leeren reeckenen, cypheren, ende boeckhouwen ... (1635 printing, first published in 1583) was a manual on reckoning, bookkeeping, simple algebra, and both plane and spherical geometry. The title page plate features a portrait of the author designating him as a man of science and learning. The scene is littered with scientific paraphernalia and activity: Petri holds a globe and dividers; a suspended polyhedron, a symbol of proficiency in geometry, hangs above his right shoulder; similarly, the armillary sphere over his left shoulder indicates excellence in astronomy. The motto above his head, “Man proposes, God disposes,” marks his humility. This portrait now resides in the Arthur M. Sackler Museum of Art at Harvard University, Cambridge, Massachusetts.

The first page of the table of contents list topics of contemporary importance: the Rule of Three, interest, allegation, etc.

The introduction to arithmetic and its operations is rather brief and concise. Numeration and the designation of numbers on a counting table is the initial topic, followed by addition, subtraction, multiplication, division, progressions, and finally a series of rules and applications relevant to merchants.

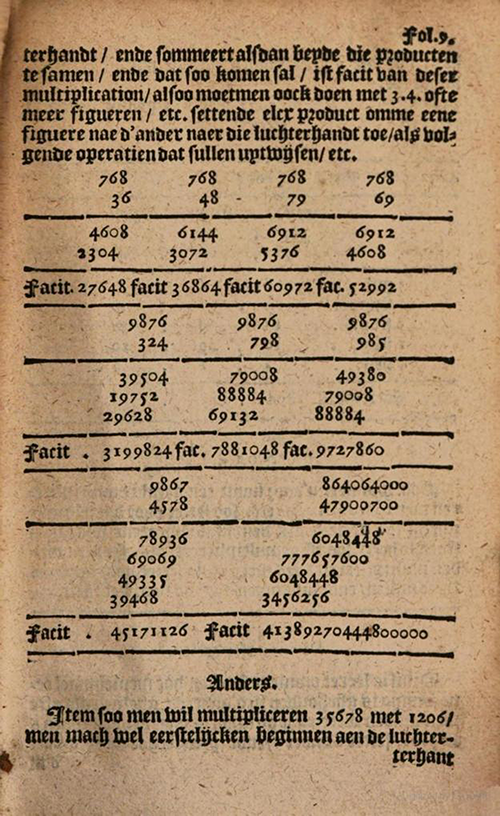
The multiplication algorithm Petri taught is the downward form, which is still popular today.

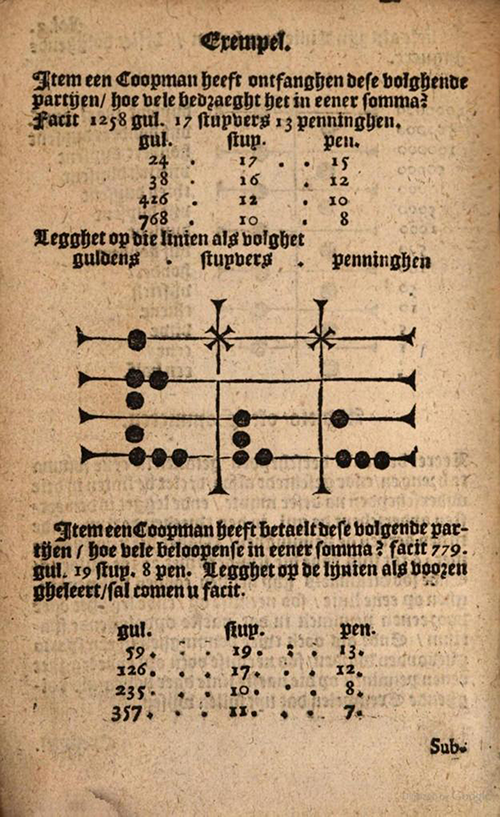
On folio 48, the reader finds a discussion and demonstration of the “Converse Rule of Three.” In modern form, the problem given at the bottom of the first page below can be read as “32 is to 6 as 48 is to x” and x is found to be 9.

Introducing the concept of a cubic equation on folio 166, Petri constructed such an equation:  \[(x + 2)^3  = (x + 2)(x + 2)(x +2) = x^3 + 6x^2 +12x + 8.\]

The section on geometry is initiated with the discussion of a 9-12-15 (3-4-5) right triangle.

Among the given geometry problems is the situation of an inscribed triangle with sides of lengths 40, 28, and 32, where the diameter of the inscribing circle is required. Note the use of construction lines in obtaining the solution.

A section on measurement employing a quadrant contains the example of finding the depth of a well.

The discussion of solid geometry includes a discussion of the triangular pyramid and its truncated form.

The latter section of the work concerns spherical geometry.

The images above were obtained through the courtesy of the University of California Libraries. The work may be viewed in its entirety in the Internet Archive.

A later Dutch arithmetic textbook, the 1682 Cijffer-boeck der heylige schrift, was written by the Middelburg schoolteacher Adriaan de Neeff. His intention was to teach children arithmetic by using numbers from the Christian Bible. Students were provided Biblical references to money, measures, or weights and shown how to convert them into contemporary Dutch units.

This image shows the copy offered for sale by Asher Rare Books.

Index to Mathematical Treasures