Mathematical Treasure: Newton's Method of Fluxions

In the frontispiece for Isaac Newton’s Method of Fluxions (1736), the ancient philosophers contemplate the principles of motion while the contemporary, seventeenth century gentlemen hunters utilize them in the quest for a moving target. The situation depicted is similar to modern day trap-shooting. The Latin inscription above the illustration reads, “The sensible measure of velocity.”

The title page for Newton’s The Method of Fluxions and Infinite Series; with its Applications to the Geometry of Curved-Lines. The work was completed in 1671, but Newton’s reluctance to publish resulted in it appearing posthumously in 1736 in a translation by John Colson (1680-1760), the fifth Lucasian Professor of Mathematics at Cambridge. (Newton was the second Lucasian Professor.) As Colson noted in the Preface:

I thought it highly injurious to the memory and reputation of the great author, as well as invidious to the glory of our own nation, that so curious and useful a piece should be any longer suppress'd.

A discussion of fluents and fluxions (in modern terms, a discussion of functions and their derivatives)

On pages 44 and 45 of his Method of Fluxions, Newton considered the determination of maximum and minimum points for a curve.

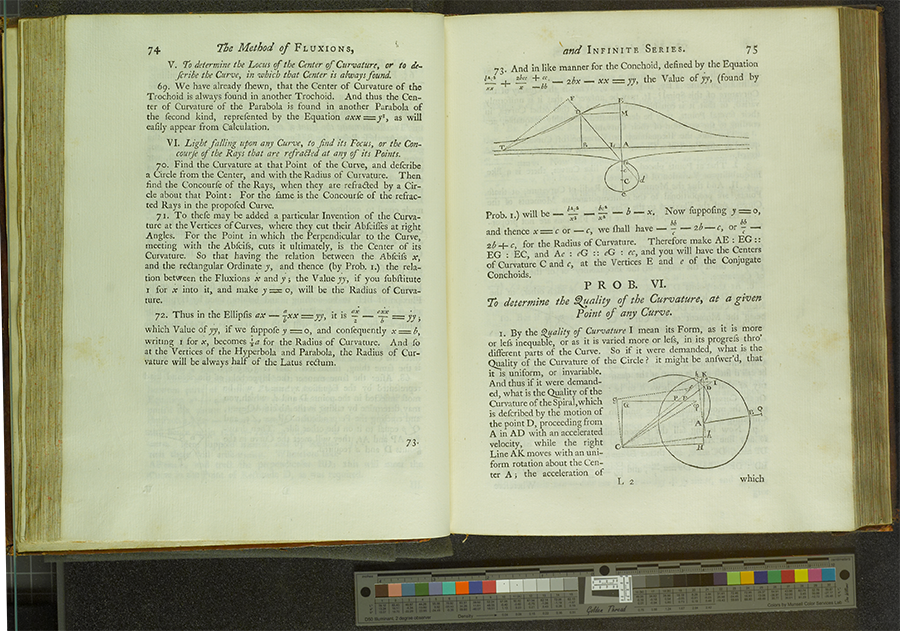
A discussion of finding the center of curvature of a given curve

The Special Collections staff at the Linderman Library of Lehigh University in Bethlehem, Pennsylvania, is pleased to cooperate with the Mathematical Association of America to exhibit this and other items from the Library’s holdings in “Mathematical Treasures.” In particular, Convergence would like to thank Lois Fischer Black, Curator, Special Collections, and Ilhan Citak, Archives and Special Collections Librarian, for their kind assistance in helping to make this display possible. You may use these images in your classroom; all other uses require permission from the Special Collections staff, Linderman Library, Lehigh University.

Index to Mathematical Treasures