- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Mathematical Treasure: James A. Garfield's Proof of the Pythagorean Theorem
 |
 |
Figure 1. Carte de visite (CDV) or visiting card featuring a photograph (by W. D. Gates & Co.) of James A. Garfield circa 1881 from the collection of the author. CDV’s were popular at the time. The card shown here was produced after Garfield’s death in 1881, presumably as a memorial souvenir. Similar but larger CDV’s were also popular at this time.
A Short Biography
James A. Garfield (1831-1881) was the twentieth president of the United States. After he graduated from Williams College in 1856, he taught Greek, Latin, mathematics, history, philosophy, and rhetoric at Western Reserve Eclectic Institute, now Hiram College, in Hiram, Ohio, a private liberal arts institute. In addition to teaching, he also practiced law, was a brigadier general in the Civil War, served as Western Reserve’s president, and was elected to the U.S. Congress.
Garfield was inaugurated on March 4, 1881. He was a popular president who supported a tenure process in civil service jobs. He was the last of seven presidents to be born in a log cabin, and the first president to be left handed. He was ambidextrous, and was known to entertain friends by simultaneously writing with one hand in Latin and the other hand in Greek. President Garfield was shot in the back on July 2, 1881, by Charles Guiteau, who had been turned down by Garfield when he requested government employment. Alexander Graham Bell fashioned an electric probe to find the bullet lodged in Garfield’s spine (see Note for further details), but after weeks of suffering, Garfield died of infection and blood poisoning on September 19, 1881. It was not the bullet that killed him, but the doctors’ unsterilized hands and equipment.
Garfield contributed an original proof of the Pythagorean Theorem to the hundreds that have been recorded over the centuries. For a repository of original proofs of the Pythagorean Theorem, see Elisha Loomis’ The Pythagorean Proposition. Garfield developed his proof in 1876 while a member of Congress; that was the year Alexander Graham Bell developed the telephone. This “very pretty proof of the Pythagorean Theorem,” as Howard Eves described it, was published in the April 1, 1876 issue of the New-England Journal of Education. Evidently the editor of the journal erroneously (or perhaps in political jest) called the theorem Pons Asinorum or “Bridge of Asses,” actually a nickname for the isosceles triangle theorem (Euclid's Elements, Book I, Proposition 5). The latter theorem may have earned its name because many medieval students had difficulty understanding the proof, for which the diagram somewhat resembles a bridge, and therefore could not cross over the bridge to subsequent proofs in Euclid's Elements.
 |
|
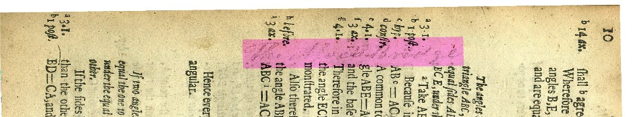
Figure 2. Isaac Barrow’s Euclid's Elements (1686) from the collection of Dr. Sid Kolpas. Proposition 5 of Book I (Euclid I-5) is shown at right. A late 17th century student wrote in the margin, “The Asses Bridge” (now very faded, highlighted in pink).
Garfield's proof of the Pythagorean Theorem essentially consists of a diagram of a trapezoid with bases a and b and height a+b. He looked at the area of the diagram in two different ways: as that of a trapezoid and as that of three right triangles, two of which are congruent.
Garfield's Proof
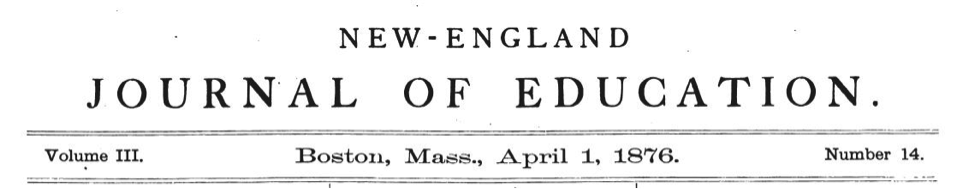
Figure 3. From the title page of the New-England Journal of Education (Vol. 3, No.14, April 1, 1876) (image from Google Books)
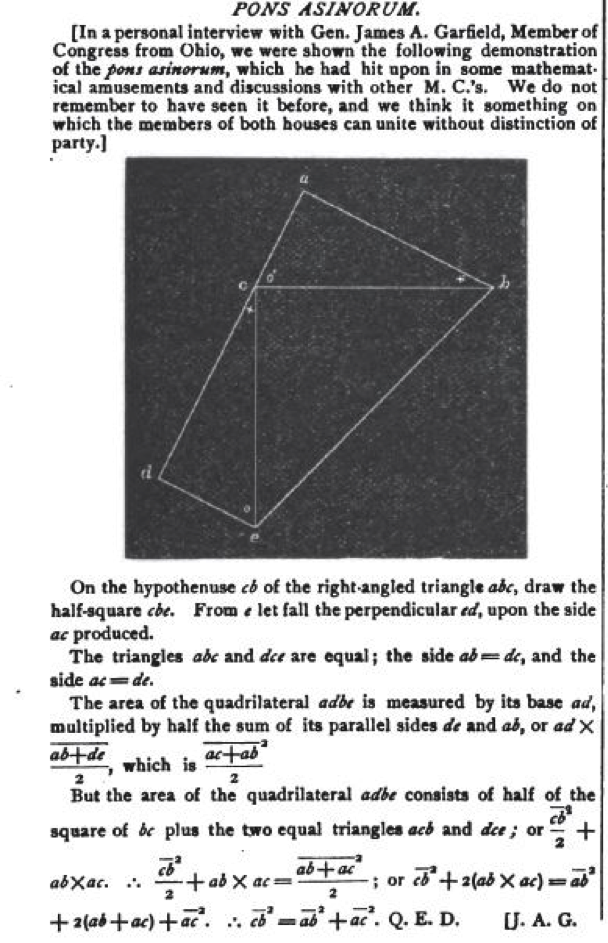
Figure 4. Garfield’s proof of the Pythagorean Theorem on page 161 of the New-England Journal of Education, April 1, 1876 (image from Google Books)
A modernized version of Garfield's proof from the author's The Pythagorean Theorem: Eight Classic Proofs follows.
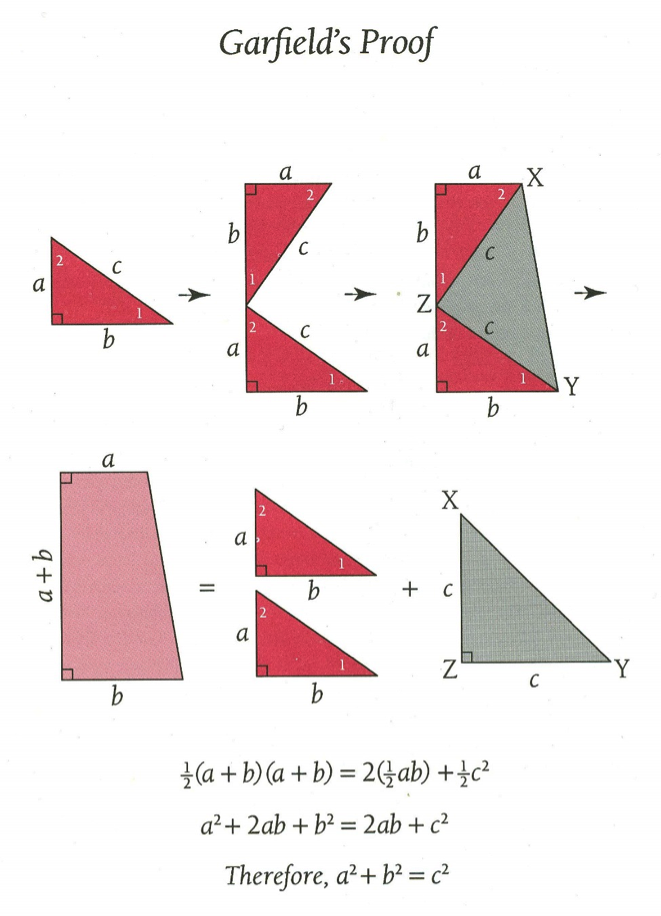
Figure 5. Modern diagram illustrating Garfield's proof (from the author's The Pythagorean Theorem: Eight Classic Proofs)
A more detailed version of the proof above follows.
- Start with a right triangle with legs a and b and hypotenuse c.
- Extend leg a by b units and construct a duplicate right triangle along this extension.
- Upper leg a is parallel to the original leg b since, in a plane, if a line is perpendicular to each of two lines, then the two lines are parallel.
- Draw segment XY to close the figure.
- The resulting quadrilateral is a trapezoid with bases a and b and altitude a+b.
- The trapezoid is composed of two congruent right triangles and right triangle XYZ. Triangle XYZ is isosceles since two of its sides have length c. Angle XZY is a right angle since angle 1 + angle XZY + angle 2 = 180o and angle 1 + angle 2 = 90o. (The acute angles of a right triangle are complementary.)
- Therefore, the area of the trapezoid equals the sum of the areas of the three right triangles of which it is composed, two of which are congruent.
- Expressing this relationship algebraically:
| Area of the trapezoid | = | 2(area of the scalene right triangle) | + | area of the isosceles right triangle |
| 12(a+b)(a+b) | = | 2(12ab) | + | 12c2 |
| (a+b)(a+b) | = | 2ab | + | c2 |
| a2+2ab+b2 | = | 2ab | + | c2 |
| a2+b2 | = |
c2 |
Note: The PBS film, "Murder of a President," depicts the physician who was attending Garfield as insisting on running Alexander Graham Bell’s electronic device over Garfield’s abdomen himself, much to the displeasure of Bell. According to the program, the physician ran it only over Garfield’s right side, insisting the bullet must be there. It turned out the bullet was on his left side, closer to the point of entry. By that time rampant infection had taken over Garfield’s body. Return to biography of Garfield.
References
Kenneth D. Ackerman. Dark Horse: The Surprise Election and Political Murder of President James A. Garfield. New York: Carroll & Graf Publishers, 2003 (now available from Viral History Press, Falls Church, Virginia, 2011).
Howard Eves. Great Moments in Mathematics (Before 1650). Washington, DC: Mathematical Association of America, 1983.
Jane C. Finn. Little Known Facts about the U.S. Presidents. Lanham, Maryland: Rowman and Littlefield Publishing Group, 2016.
James A. Garfield. "Pons Asinorum." New-England Journal of Education, Vol. 3, No.14, page 161. Boston, MA: April 1, 1876. Available via Google Books.)
J. L. Heilbron. “The Bridge of Asses.” Encyclopedia Britannica. http://www.britannica.com/topic/The-Bridge-of-Asses
Sidney J. Kolpas. The Pythagorean Theorem: Eight Classic Proofs. Palo Alto, CA: Dale Seymour Publications, 1992 (all rights held by author since 2011).
Elisha S. Loomis. The Pythagorean Proposition. Reston, VA: National Council of Teachers of Mathematics, 1968.
James D. McCabe. Our Martyred President. The Life and Public Services of Gen. James A. Garfield. Philadelphia, PA.: National Publishing Company, 1881.
Rob Rapley (director, co-producer, telescript author). "Murder of A President" in American Experience television series. PBS: first aired Feb. 2, 2016. http://www.pbs.org/wgbh/americanexperience/films/garfield/
Sid J. Kolpas (Delaware County Community College), "Mathematical Treasure: James A. Garfield's Proof of the Pythagorean Theorem," Convergence (February 2016)






