Mathematical Treasure: Geometry of Jakob Steiner

Jakob Steiner (1796–1863) was a Swiss mathematician who specialized in geometry. Considered one of the greatest pure geometers who ever lived, he introduced the concept of geometric forms, perfected the theory of duality in geometry, and contributed much to the development of modern projective geometry. Shown above is the title page of his Lectures, originally published in 1867 after his death by his former student Heinrich Schröter (or Schröder). The copy shown above is from the second edition of 1876, to which Schröder added his own second part, “The theory of conic sections based on projective properties,” further demonstrating Steiner’s theories.

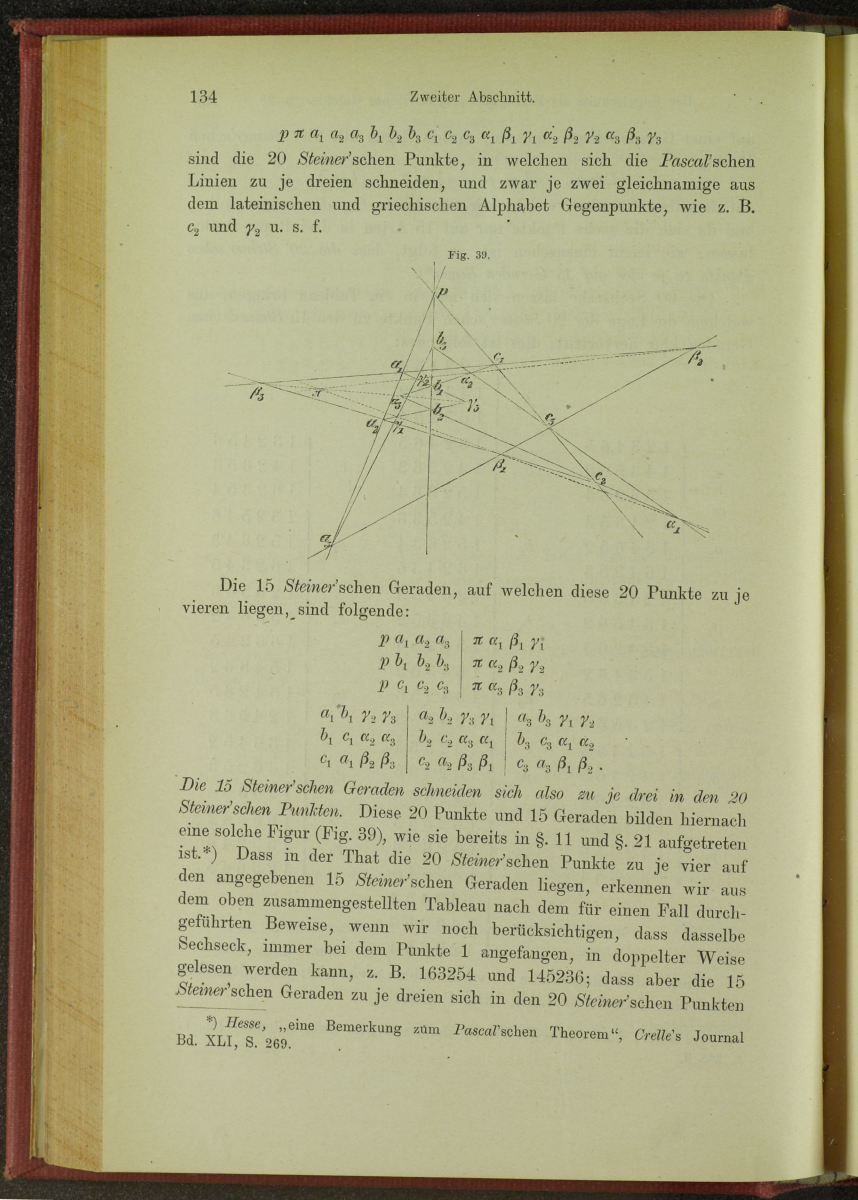
On page 134, Schröder employed the concept of Steiner points to examine the relationship of the lines in Figure 30.

The Special Collections staff at the Linderman Library of Lehigh University in Bethlehem, Pennsylvania, is pleased to cooperate with the Mathematical Association of America to exhibit this and other items from the Library’s holdings in “Mathematical Treasures.” In particular, Convergence would like to thank Lois Fischer Black, Curator, Special Collections, and Ilhan Citak, Archives and Special Collections Librarian, for their kind assistance in helping to make this display possible. You may use these images in your classroom; all other uses require permission from the Special Collections staff, Linderman Library, Lehigh University.

Index of Mathematical Treasures