- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Do We Teach Too Much Mathematics . . . ? What is Mathematics?
Starting with the “What?” It's mathematics! "Well, then what is mathematics?" This is a question every teacher of mathematics should confront. In high school, we usually teach algebra before geometry. Both are mathematics; how are they the same? What is the common thread? The (hidden) connection perplexed me as a student. I’ll supply an answer but readers should also seek their own. Mathematics is a study of relationships and implications. It has a structure and is a deductive science. A learner identifies quantity by examining sets of different sizes and arriving at an ordering cognitively and then, perhaps, physically—thus symbols such as >, <, = and ≠. Differences are then distinguished by use of a measure and numbers. With the addition of operations, a working structure for arithmetic is established. Patterns appear. A process of repeated addition leads to the operation of multiplication and in a similar manner, the recognition of multiplication and division as inverse operations provides methods for computational checks involving these operations. In geometry, the perpendicularity of two lines implies the existence of right angles. In the study of calculus, a definite integral —> a derivative —> mathematical change. And so it goes on: one concept leading to another. A good textbook, or scope and sequence, would be mindful of such a progression of implications. It is an understanding of this network of connections that we want to impart to students. But in appreciating this “What?” the humanity of mathematics—the peoples, cultures, times and places—should also be mentioned.
Confronted with such an idea, often a teacher will respond; “I teach mathematics and have no time for history!” An understandable response, but I would counter that the teaching of a subject also involves the teaching about a subject. No extensive diatribe is required—just a word or comment, an analogy, or pointed reference to a visual illustration. [1] For example, in a lesson involving the operation of division, a teacher might interject “that during the Middle Ages, performing division was considered very hard, it was called the Iron Operation and there were several algorithms for attempting the task." (See Figure 2.) Noting that Italian merchants (such Marco Polo, Luca Pacioli, and Fibonacci) were the group of people who really developed our arithmetic relates people with mathematics. In a similar manner, algebra should be associated with the Arab word “al-jabr”; the name “Euclid of Alexandria” mentioned in geometry lessons; the Newton-Leibniz controversy cited to add intrigue and relevant questions to the understanding of calculus. Often the historical sequence in the development of a concept suggests the pedagogical approach used in the classroom.
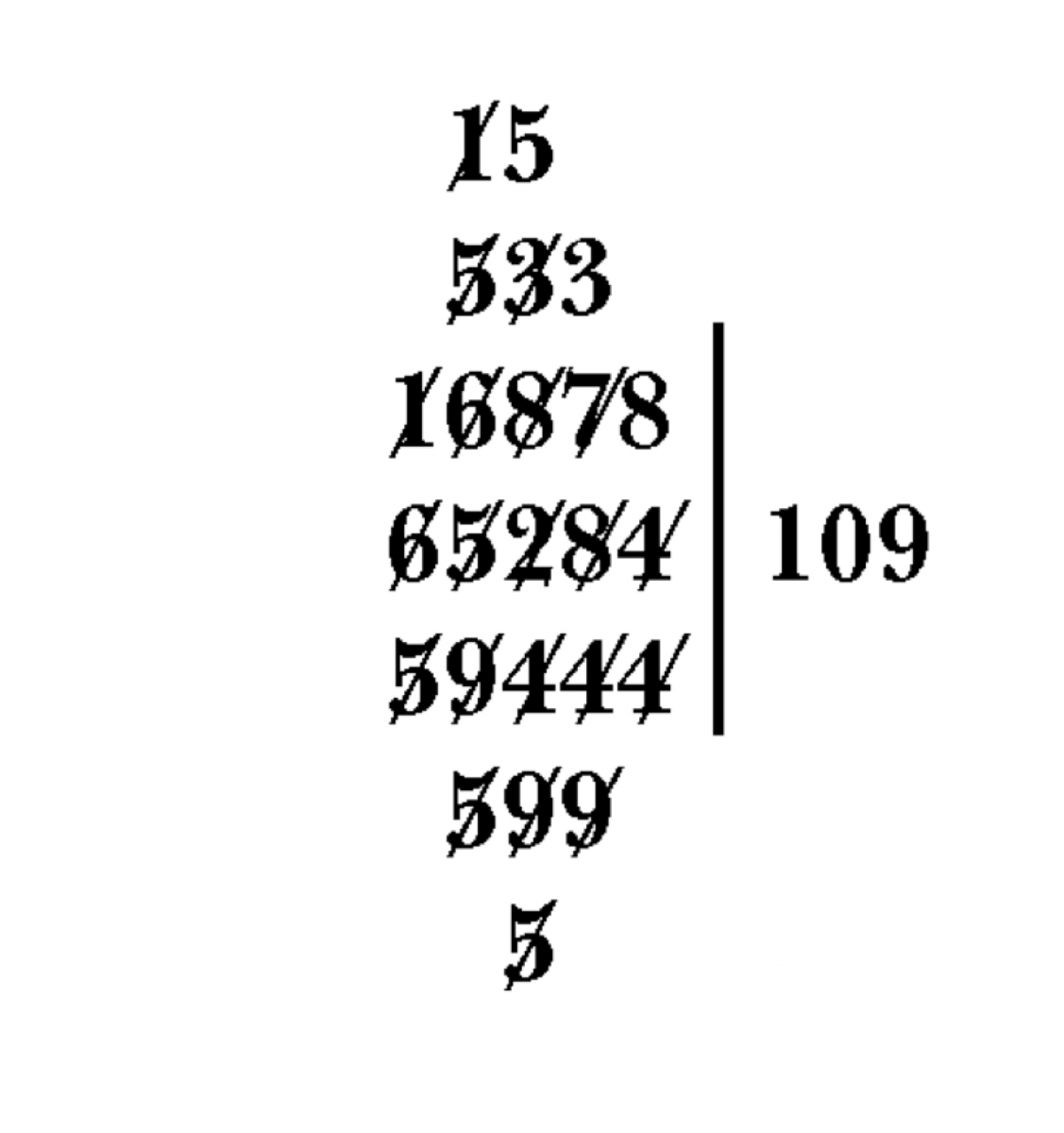
Figure 2. “Galley Division” was the most popular European form of division. Each algorithm bore a distinctive name to assist in its memorization. “Galley” refers to a ship’s sails. At left above, the quotient of 65284 divided by 594 is found to be 109 538/594. While this technique was considered fairly easy to use, printers did not like it and it fell out of fashion. The figure at right above, from Hodder's Arithmetic (9th ed., 1671, pp. 54–55), may help illustrate printers' objections.
For example, the trigonometric functions are first introduced as discrete ratios found from a right triangle, ratios that were first realized through the physical task of “shadow reckoning”; projected heavenly, they became continuous, circular functions, derived from sweeping arc lengths. (See Figure 3.) Ask open-ended questions—let pupils find out, for instance, “Why is the weight measure 'pound' abbreviated as 'lb.'?" “Did Pascal really discover Pascal’s Triangle?" “Why is division by zero undefined?” Little mysteries, inserted as an aside, arouse curiosity and often further enhance self-learning. So mathematics is revealed as a structured, deductive science; a game, with a long history and played by humans, where the number of rules and pieces constantly increase, a game in which our students are invited to participate. Pedagogical emphasis on the structure of mathematics, to be fully successful, should be a cooperative effort within a school system. From at least the middle-school level onwards, mathematics teachers ought to recognize and follow a similar deductive teaching strategy that constructs a chain of understanding for their students.
Figure 3. In 1532, the scholar and instrument maker Peter Apian published Quadrans Apiani astronomicus, an instructional manual on the use of the quadrant. In this illustration from Apian’s book, a quadrant, rather than the available shadow of a tower, is used to find the inclination of the sun’s rays. From the information provided, can you determine this angle?
Notes:
[1] The Mathematical Association of America maintains an archive of historical mathematical images, “Mathematical Treasures”, available free of charge for use by teachers: https://www.maa.org/press/periodicals/convergence/index-to-mathematical-treasures.
Frank J. Swetz (The Pennsylvania State University), "Do We Teach Too Much Mathematics . . . ? What is Mathematics?," Convergence (June 2019)