Who's That Mathematician? Paul R. Halmos Collection - Page 35

For more information about Paul R. Halmos (1916-2006) and about the Paul R. Halmos Photograph Collection, please see the introduction to this article on page 1. A new page featuring six photographs will be posted at the start of each week during 2012.

Index to the Collection

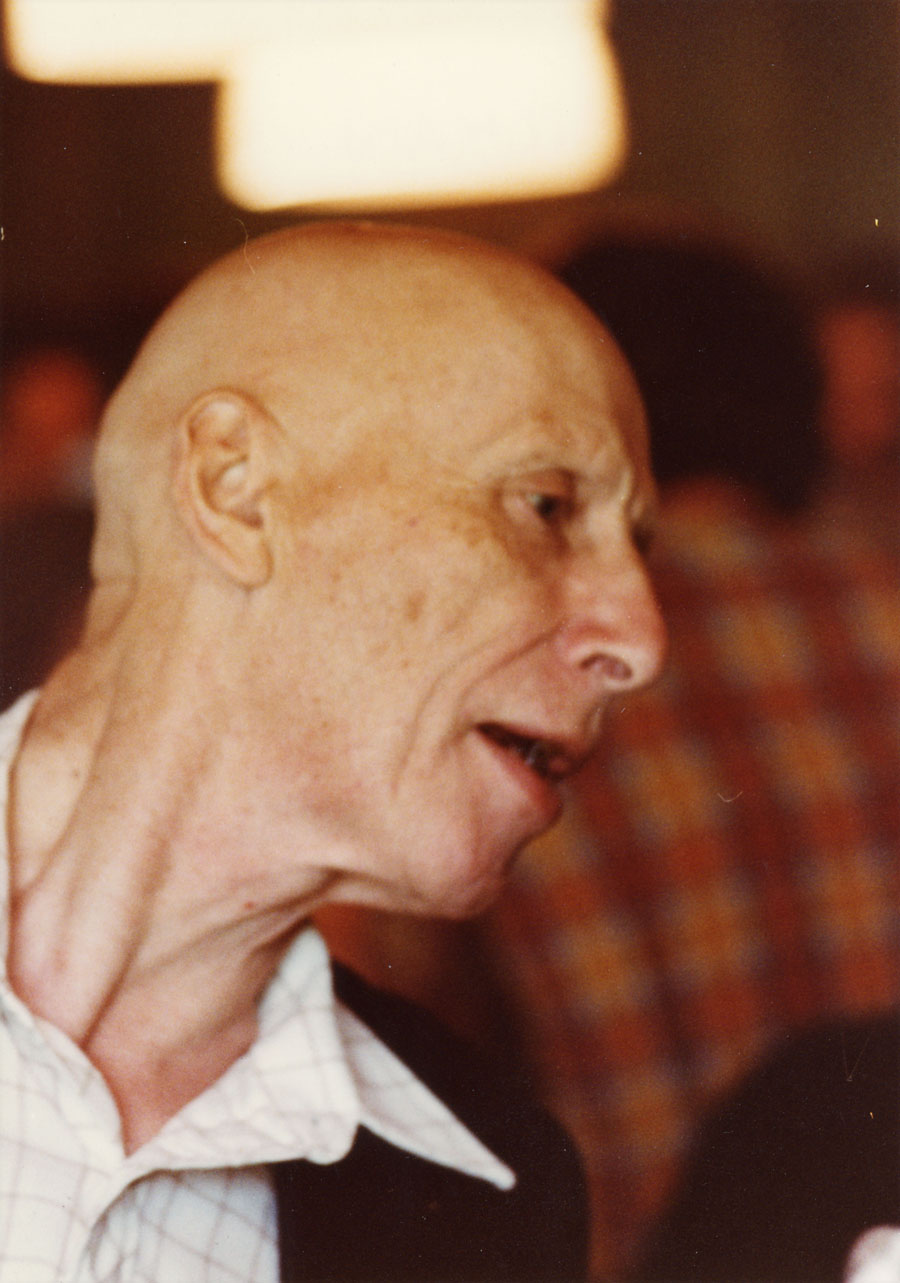
Halmos photographed David Milman (1912-1982) in 1980 at the Oberwolfach Conference Center in Germany. Halmos and Milman were participating in one of a series of seven Oberwolfach conferences on approximation theory organized approximately every three years from 1963 to 1983 by Paul Butzer and Béla Szökefalvi-Nagy. (For a photo of Butzer and Sz.-Nagy at the 1983 conference, see page 9 of this collection.) In his book, I Want to Be a Mathematician: An Automathography (Springer, 1985), Halmos described his attraction to Milman’s “ebullient and instantly likable personality” (p. 387).

Milman earned his Ph.D. in functional analysis in 1939 from Odessa State University in Odessa, Ukraine. He emigrated to Israel during the 1970s and became a professor at Tel Aviv University. The following paragraph appeared at many websites, including Wikipedia, as of Sept. 15, 2012:

Milman is known for his development of functional analysis methods, particularly in operator theory, in close connection with concrete problems coming from mathematical physics, in particular differential equations and normal modes. The Krein–Milman theorem and the Milman–Pettis theorem are named after him.

The source of this paragraph was apparently the Wikipedia article on Milman, for which I hope the source was the introductory article “David Milman (1912-1982)” on pages ii-7 of the “David Milman Memorial Issue” of the journal Integral Equations and Operator Theory 9:1 (Springer 1986). Milman’s sons Vitali Milman and Pierre Milman are professors of mathematics at Tel Aviv University and the University of Toronto, Canada, respectively. There is another photograph of Milman that appears to be from about 1980 in Gil Kalai’s blog post about a geometry and functional analysis conference held June 24-30, 2009, at Tel Aviv University in honor of David’s son Vitali Milman’s 70^th birthday (the first photograph there is of Vitali Milman). (Sources: Mathematics Genealogy Project, Wikipedia, SpringerLink, Gil Kalai’s blog post from 7/12/2009 at WordPress.com)

John Milnor was photographed by Halmos in October of 1979 at Indiana University in Bloomington, Indiana. Halmos was a mathematics professor at Indiana from 1969 to 1985. Milnor earned his Ph.D. in 1954 from Princeton University with the dissertation “Isotopy of Links,” written under advisor Ralph Fox. He had accepted a faculty position at Princeton in 1953, and in 1956 he published the paper, “On manifolds homeomorphic to the 7-sphere,” in Annals of Mathematics. In 1962, he was awarded the Fields Medal for proving “that a 7-dimensional sphere can have several different structures; this led to the creation of the field of differential topology” (see “Fields Medals 1962”). In 1960, he had been promoted to Professor at Princeton and, in 1962, besides winning the Fields Medal, he also became editor of Annals of Mathematics. During his career, Milnor has worked on algebraic topology, algebraic K-theory, differential geometry (including knot theory), and dynamical systems. In 1988, he joined the mathematics faculty at the State University of New York (SUNY) at Stony Brook, where he is Professor of Mathematics and Co-Director of the Institute for the Mathematical Sciences. (Sources: MacTutor Archive, International Mathematical Union Fields Medals 1962, SUNY Stony Brook Mathematics)

Halmos photographed George Minty on October 8, 1969, at Indiana University in Bloomington. Halmos had just taken up a faculty position at Indiana that fall and would remain there until 1985. Minty had been a mathematics professor at Indiana since the early 1960s and worked in graph theory, electrical networks, and nonlinear functional analysis. After service in the U.S. Army Signal Corps, Minty earned his Ph.D. in 1959 from the University of Michigan with the dissertation, “Integrability Conditions for Vector Fields in Banach Spaces.” His first job out of grad school was as an applied mathematician at the General Motors Research Laboratory in Detroit, Michigan, and it was here that he first melded the discrete and the continuous to obtain significant results. According to Minty’s colleague and friend, Andrew Lenard, now Professor Emeritus of Mathematics at Indiana University:

Perhaps his most significant work during that period was his analysis of the general electrical circuit system, consisting of a finite number of "units" (with arbitrary non-linear characteristics) joined together at junction points, and subject to prescribed external electric signals. He proved mathematically the existence and uniqueness of a solution for the "outputs" using ingenious mathematical methods: He treated first an approximating finite problem with methods of graph- and graph-minimizing theories, and then embedded it in a general functional analysis space to carry out the limit indicated. This work has stimulated much later research by him and others, and was the source of Minty's lifelong interest in discrete mathematics, as well as (mostly non-linear) functional analysis.

Lenard listed Minty’s three most important accomplishments after becoming a mathematics professor at Indiana University as

• the theory of monotone operators on normed linear spaces (also developed independently by Brower),
• the Lemma of the Colored Arcs in matroid theory, and
• in the theory of computation (efficiency of algorithms), a proof that a certain decision problem in a certain type of graph has an algorithmic solution that is only polynomially bounded.

Lenard also described Minty as a “passionate teacher.” Minty’s most-cited result may be his and Victor Klee’s worst-case analysis of the simplex method, including an m-cube example that requires an exponential number (2^m) of pivots, published in 1972 (see David Gale’s article). Minty died at age 56 sometime between 1985 and 1990. (Sources: Mathematics Genealogy Project; Andrew Lenard’s post to the Historia Matematica listserve dated Oct. 20, 1999; David Gale, “Linear Programming and the Simplex Method,” AMS Notices 54:3 (March 2007), pp. 364-369)

Edwin E. Moise (1918-1998) was photographed by Halmos in August of 1985 at the AMS-MAA Joint Summer Meetings at the University of Wyoming in Laramie, Wyoming. After serving as a crytanalyst during World War II, Moise earned his Ph.D. in general topology in 1947 from the University of Texas at Austin under advisor R. L. Moore. According to fellow Moore Ph.D. student Richard Anderson, Moise’s thesis, in which he constructed and named the pseudoarc, was one of the best of the 50 dissertations advised by Moore (see “Interview”). Moise was on the faculty at the University of Michigan from 1947 to 1960, and then spent two years at the Institute for Advanced Study (IAS) in Princeton, New Jersey, where he obtained his most famous mathematical result, proving that every 3-manifold could be triangulated. He was a professor at Harvard University in Cambridge, Massachusetts, from 1961 to 1971 and at Queens College in Queens, New York, from 1971 to 1987. During the 1960s, Moise participated in the “New Math” project in the U.S. and wrote geometry textbooks for students and teachers. His first Ph.D. student was James Munkres (University of Michigan, 1956) of topology textbook fame and his second Ph.D. student was Ross Finney (UM, 1962) of calculus textbook fame. Moise was president of the MAA during 1967-68. (Sources: Mathematics Genealogy Project; MAA Presidents; “An Interview with Edwin Moise,” Topology Atlas, April 10, 2000; ICMI Biography)

Halmos photographed topologist Deane Montgomery (1909-1992) in August of 1985 at the AMS-MAA Joint Summer Meetings at the University of Wyoming in Laramie. Montgomery earned his Ph.D. in point-set topology in 1933 from the University of Iowa. After one year each at Harvard and Princeton (one year after the Institute for Advanced Study was founded), he taught at Smith College in Northampton, Massachusetts, from 1935 to 1946; then, after two years at Yale University, he became a permanent faculty member at the Institute for Advanced Study (IAS) in 1948. He was president of the AMS during 1961 and 1962 and president of the International Mathematical Union from 1974 to 1978. During the 1930s, Montgomery turned from point-set topology to transformation groups. He is best known for his contributions to the solution of Hilbert’s Fifth Problem, solving it in dimension 3 in 1948 and in the finite-dimensional case in 1952. The problem was solved in 1952 by Andrew Gleason, Montgomery, Leo Zippin, and Hidehiko Yamabe, with Gleason providing the final piece of the solution. (Sources: MacTutor Archive, AMS Presidents)

Halmos photographed Robert L. Moore (1882-1974) and Virginia Halmos (1915-2015) on January 20, 1970, at the University of Texas in Austin, Texas.

R. L. Moore earned his Ph.D. in 1905 from the University of Chicago with the dissertation, “Sets of Metrical Hypotheses for Geometry,” written under Oswald Veblen and E. H. Moore (no relation). After one year at the University of Tennessee, two years at Princeton, three years at Northwestern University in Evanston, Illinois, and nine years at the University of Pennsylvania in Philadelphia, he returned to his alma mater, the University of Texas at Austin, in 1920. His research was in geometry and point-set topology, but he is even better known for the teaching method known as the “Moore Method,” which is still in use today. In Moore’s version of his Method, a group of students whose background knowledge in the given course topic was as uniform as possible would compete (and, in a restricted sense, cooperate) to prove as many of the theorems in that area, often geometry or point-set topology, as possible. He first taught a geometry course using this method at the University of Pennsylvania. During his career, Moore advised 50 Ph.D. students, the first three at the University of Pennsylvania and the rest at the University of Texas, from which he was forced to retire at the age of 86. He was president of the AMS during 1937-38. (Sources: MacTutor Archive, Mathematics Genealogy Project, AMS Presidents)

Virginia Halmos married Paul Halmos (1916-2006) in 1945. Virginia and Paul Halmos have been great friends of the MAA at least since the 1980s, when Paul served as editor of the MAA’s flagship journal, The American Mathematical Monthly. In the early 2000s, they contributed funds to restore the carriage house on the grounds of the building housing the MAA national offices in Washington, D.C., and to convert it to the MAA Carriage House Conference Center, completed in 2007 and now in operation. After Paul’s death in 2006, Virginia Halmos contributed his mathematical papers and his photograph collection to the Archives of American Mathematics at the University of Texas at Austin and provided funding to digitize the collection, a project that is now in progress. She continued to live in Los Gatos, California, near San Jose, where she and Paul moved in 1985 when he accepted his friend Gerald Alexanderson’s offer of a position in the mathematics department at Santa Clara University, until her death in January of 2015. (Source: MAA News: "Virginia Halmos Dies at Age 99")

For an introduction to this article and to the Paul R. Halmos Photograph Collection, please see page 1. Watch for a new page featuring six new photographs each week during 2012.

Regarding sources for this page:  Information for which a source is not given either appeared on the reverse side of the photograph or was obtained from various sources during 2011-12 by archivist Carol Mead of the Archives of American Mathematics, Dolph Briscoe Center for American History, University of Texas, Austin.

Index to the Collection