- About MAA
- Membership
- MAA Publications
- Periodicals
- Blogs
- MAA Book Series
- MAA Press (an imprint of the AMS)
- MAA Notes
- MAA Reviews
- Mathematical Communication
- Information for Libraries
- Author Resources
- Advertise with MAA
- Meetings
- Competitions
- Programs
- Communities
- MAA Sections
- SIGMAA
- MAA Connect
- Students
- MAA Awards
- Awards Booklets
- Writing Awards
- Teaching Awards
- Service Awards
- Research Awards
- Lecture Awards
- Putnam Competition Individual and Team Winners
- D. E. Shaw Group AMC 8 Awards & Certificates
- Maryam Mirzakhani AMC 10 A Awards & Certificates
- Two Sigma AMC 10 B Awards & Certificates
- Jane Street AMC 12 A Awards & Certificates
- Akamai AMC 12 B Awards & Certificates
- High School Teachers
- News
You are here
Logarithms: The Early History of a Familiar Function - Appendix: Student Tasks
The Logarithms of Napier and Bürgi
1. Laplace: “Logarithms, by shortening the labors, doubled the life of an astronomer.” What does this quote mean? Give an example of another “device” that can be thought of as having the same effect on mathematicians, scientists, or other professionals.
2. As discussed, Napier's tables were not tabulated in terms of logarithms themselves but rather by the minutes of arc and their sines. But that is not all he included! Ingeniously he organized his data into seven columns, as seen in Figure 8, including the angle, its corresponding sine, and the corresponding logarithm, as well as the logarithm of its tangent, the logarithm of its cosine, its cosine, and the complement of the angle. From your knowledge of trigonometry and especially the relations between sine, cosine, and tangent, identify each of these columns and explain how they are related (hint: the fourth column is called “Differences”).
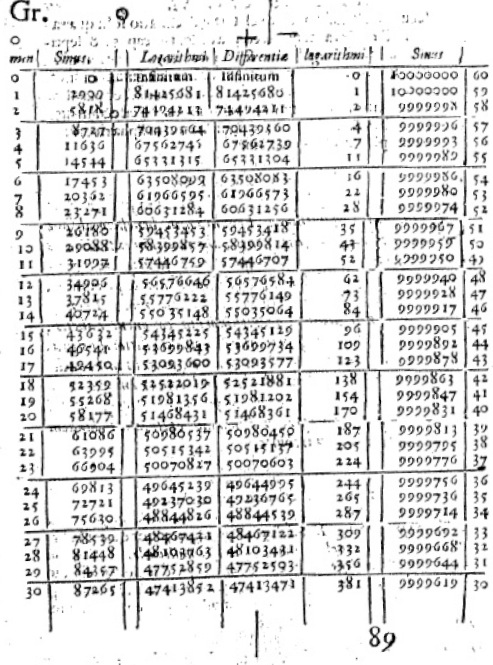
Figure 8. An excerpt from the first page of Napier’s tables
(Image used courtesy of Landmarks of Science Series, NewsBank-Readex)
3. Using the excerpt in Figure 9 from page 3 of Bürgi's Arithmetische und Geometrische Progress Tabulen (1620), explain the calculations (on the red numbers) that he used to determine the fourth value in the following “rule of three” computation:
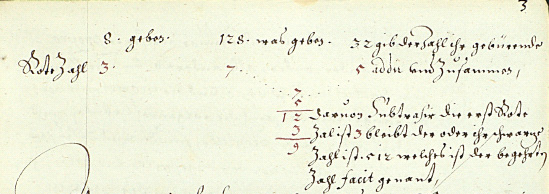
Figure 9. An excerpt from Bürgi's Progress Tabulen (Image used by permission of University Library - Graz)
Translation:
8 gives 128 which gives 32. Into their appropriate red numbers:
| 3 | 7 | 5 add together: | |||||
| 7 | |||||||
| 5 | |||||||
| 12 | Subtract from this the first red number, 3, | ||||||
| 3 | |||||||
| 9 | its black number is 512 which is the desired numer. |
4. Solve the proportion in Problem 3 using a modern method and verify the results.
5. Using the relationship, bn=(108)(1.0001)R/10, where bn is a black number and R is its corresponding red number, verify the table entries for R=221500, 221510, and 221520, as given in the upper right portion of Figure 10.
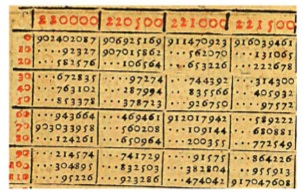
Figure 10. Another excerpt from Burgi’s tables
(Image used by permission of University Library - Graz)
6. The “Temple of Astronomy” (below, in Figure 11) appears as the frontispiece in Kepler's Rudolphine tables, a work commissioned in 1601 (and completed in 1627) by the emperor Rudolf II. The temple shows six muses of astronomy, the goddesses Physica (physics), Optica (optics), Geometrica (geometry), Stathmica (weights), Magnetica (magnetics), and Logarithma (logarithms). Logarithma, placed third from the left, holds in her hands two unequal measuring sticks, rods of the ratio of one to two. Around her head is haloed the number “6931472.”
(a) What does this number represent and why do you think it appears in Logarithma's halo?
(b) Match the remaining five goddesses with their correct names.

Figure 11. Temple of Astronomy from Kepler’s Rudolphine Tables
(Image used by permission of the Columbia University Library. For two more images from Kepler's Rudolphine Tables, see "Mathematical Treasures" in Convergence.)
Kathleen M. Clark (The Florida State University) and Clemency Montelle (University of Canterbury), "Logarithms: The Early History of a Familiar Function - Appendix: Student Tasks," Convergence (January 2011), DOI:10.4169/loci003495





