Limit Points and Connected Sets in the Plane - Mullikan's Nautilus

In October 1919 the renowned mathematician R. L. Moore read a paper written by his third doctoral student Anna M. Mullikin before an AMS meeting at Columbia University in New York City.  Titled “A countable collection of mutually exclusive closed point sets with connected sum,” it presented a clever yet fairly simple example that Miss Mullikin constructed during her first year under Moore’s tutelage at the University of Pennsylvania [1, pp. 146-147].  Ultimately the example, which has come to be known as “Mullikin’s nautilus,” constituted the first part of Mullikin’s doctoral dissertation, which was published in the Transactions three years after her first AMS presentation [3].

Mullikin’s nautilus consists of an infinite collection of arcs M_n, for n = 1, 2, 3, … , each composed of four line segments drawn from the x-intercept (1/2^n-1, 0) to (1/2^n-1, 1/2^n-1) , thence to (-1/2^n-1, 1/2^n-1) and (-1/2^n-1, -1/2^n-1), and finally to (1, -1/2^n-1) .  The abscissa of the final point is always x = 1.  Figure 1, taken from Mullikin’s dissertation, displays the first three color-coded arcs: M₁, M₂, and M₃.  Formally, Mullikin’s nautilus is defined as M = U M_n.

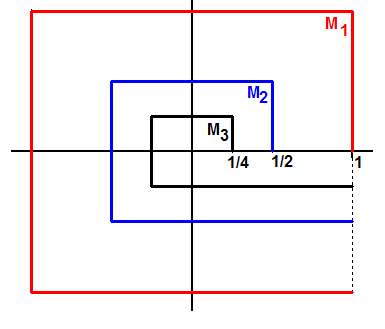
Figure 1

Clearly each arc is connected, but the union M₁U M₂ U M₃ is not.  In Figure 1, for instance, the point (1, -1) on M₁ cannot be connected to the point (1, -1/4) on M₃. We pose the following

FUNDAMENTAL QUESTION: Is Mullikin’s nautilus M = U M_n connected?

The reader might want to hazard a guess now.  [Disclosure: this is not an easy issue to resolve.]  The answer will be divulged shortly.