Sacred Mathematics: Japanese Temple Geometry

Japan’s Tokugawa, or Edo, shogunate (1603–1868) began a pronounced period of pacification. The samurai lost possession of their land and became either peasants or they moved into the cities where they were transformed over a few generations from relative illiteracy into a highly educated class. Many became poorly paid civil servants while, due to the lack of a Japanese university system, some went into teaching in private schools, the juku, to supplement their meager incomes. The beginnings of wasan, or traditional Japanese mathematics, can be traced to 1627 with the publication of the Jinkō-ki and the widespread use of the abacus, or soroban. But while traditional wasan had immediate use in practical applications, a class of samurai mathematicians who had earned their “Master of Mathematics” certificates began more formal abstract forms of study. Of course, much of this was or could be applied, but there was also progressive development of more pure form of mathematical problems for their own sake.

As solutions to these problems were found, many authors would produce a plaque, or sangaku, on which the problem, often along with its final answer; more rarely, the plaque would include its detailed solution. The sangaku would be hung in a Shinto shrine or Buddhist temple, possibly as a praise to the divine inspiration for its discovery. The oldest surviving sangaku was found in the Tochigi prefecture and dates from 1683; the newest was found in the Ubara shrine, dating from about 1870. About 900 sangaku survive today, and it is known that at least 1,738 have been lost. Many of the sangaku are beautiful works of art. One, combining two high-school algebra problems, is on the cover of Sacred Mathematics.

This book was inspired by the efforts of Fukagawa Hidetoshi, the Japanese high-school teacher who, together with the late Daniel Pedoe, published Japanese Temple Geometry Problems, (Charles Babbage Research Centre, Winnipeg, 1989) from his large collection of sangaku. Some while ago Freeman Dyson, formerly one of Pedoe’s students, had sought and briefly was allowed to meet the “lowly” Fukagawa on a trip to Japan (high-school teachers were evidently not highly esteemed by Dyson’s particular academic Japanese hosts; because of the difference in stature between the renowned physicist and Fukagawa, they were only permitted an abbreviated meeting). More recently, Dyson introduced Rothman to Fukagawa and inspired the interesting collaboration that led to this book. In his preface, Rothman notes that the collaboration was quite unusual: he did not meet Fukagawa in person, does not speak Fukagawa’s native Japanese. The collaboration was conducted completely through e-mail thanks to the universality of mathematics.

Sacred Mathematics allocates approximately a third of its chapters to a narrative history of Japanese mathematics and important individual mathematicians along with their contributions and societal memberships. Three additional chapters are devoted to sangaku problems, sorted by difficulty and required mathematical tools. Finally, there is a more technical discussion of sangaku Japanese mathematical methods and tools, along with a comparison with their Western mathematical counterparts. The book concludes with a good bibliography and a useful index.

The problems are presented separate from their solutions. The problems range from high-school algebra and elementary number theory (including applications of the Chinese Remainder Theorem) to plane and solid geometry and a few minimization/maximization problems we would solve using the calculus. When the traditional Japanese solution method is known, it is presented in each chapter’s solution section. However, all too often the technique used by the problem’s author was not on the plaque and is either hypothesized or a modern solution is presented.

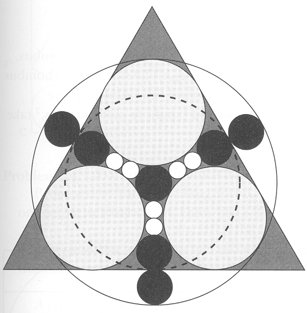
Sangaku geometry problems are distantly removed from the class of geometry problems presented in Western geometry texts. A typical ‘easier’ problem, on page 97 of the book, is shown below. In an equilateral triangle, three grey circles of radius a, five dark circles of radius b, and six white circles of radius c touch each other as shown. If R is the radius of the outer circle, and r is the radius of the dashed circle, find c.

This book contains illustrations and color plates of several other sangaku plaques, of which Plate 9, reproduced below, displays seven problems and is my personal favorite. It dates from 1800 and was placed in the Mizuhoshrine in Shimotakaigun in Nagana prefecture. It measures 160 cm by 58 cm. In the second problem from the left, five squares are inscribed in a right triangle. The problem is to express the altitude of the triangle in terms of the sides of the topmost and rightmost inscribed squares.

Sacred Mathematics’ seventh chapter consists of extracts from the diary of Yamaguchi Kanzan, who travelled for the six years from the spring of 1816 to the winter of 1821 visiting shrines and temples all over Japan. Yamaguchi writes about various mathematicians he visits as well as presenting sangaku problems and his solutions. This chapter drives home how widespread and sophisticated the sangaku tradition had become.

In the book’s final chapters, one finds a discussion of inversion as a technique that leads to simpler solutions of many of the most difficult circle-related sangaku. There is also a good discussion of traditional knowledge of derivatives, unknown to sangaku mathematicians, and of enri, the Japanese form of integration that is quite reminiscent of the lower-sum definition of the Riemann integral. Interestingly, 17th and 18th century mathematicians estimated values of π by computing the circumference of inscribed polygons with 2¹⁵ or even 2¹⁷ sides (a 131,072-sided polygon!) yielding a value of π = 3.141592664 computed on a soroban. A disciple of Seki, Takabe Katahiro (1664-1739) obtained a value correct to 41 places!

I found this to be an exciting and enjoyable book. The treatment is fluid and consistently accessible. Most of the mathematical prerequisites are summarized early in the text, and a dimly-remembered knowledge of Euclidean geometry and trigonometry is sufficient for addressing most of the problems. But be warned, even the simplest of the geometry problems is far from trivial, and most will provide the reader with a rewarding challenge.

Sacred Mathematics is handsomely printed on high gloss paper with crisp, high contrast type and is durably bound in cloth with sewn signatures. The color plates are clear and bright. Though it has the appearance of a “coffee table” book, the content is anything but superficial and will offer the reader days or weeks of intensive problem solving. The improbable collaboration between Fukagawa and Rothman has produced a beautiful and technical introduction to Japanese mathematics. My only real complaint is that the reproductions of the wide sangaku plaques are too small to afford close study. Like all books of this complexity, the reader will encounter a few typographical errors, but none that cannot be readily corrected.

Marvin Schaefer (bwapast@erols.com) is a computer security expert and was chief scientist at the National Computer Security Center at the NSA, and at Arca Systems. He has been a member of the MAA for 40-plus years and now operates an antiquarian book store called Books With a Past.